Area

In this article, we will learn what area is, and understand how it is calculated for each shape, in the most practical and simple way there is.
Shall we start?

What is the area?

Area is the definition of the size of something. In mathematics, which is precisely what interests us now, it refers to the size of some figure.
In everyday life, you have surely heard about area in relation to the surface of an apartment, plot of land, etc.
In fact, when they ask what the surface area of your apartment is, they are asking about its size and, instead of answering with words like "big" or "small" we can calculate its area and express it with units of measure. In this way, we can compare different sizes.

Units of measurement of area

Large areas such as apartments are usually measured in meters, therefore, the unit of measurement will be $m^2$ square meter.
On the other hand, smaller figures are generally measured in centimeters, that is, the unit of measurement for the area will be $cm^2$ square centimeter.
Remember:
Units of measurement for the area in $cm => cm^2$
Units of measurement for the area $m=>m^2$

Detailed explanation

Start practice

Test yourself on area of the square!

Complete the sentence:

To find the area of a right triangle, one must multiply ________________ by each other and divide by 2.

Practice more now

Now we will learn to calculate the area of (almost) all the shapes we know! Are we ready?

Area of the Square

$a$ Side of the square

$a\times a=Area~ of ~the ~square$

$A=a^2$

We will multiply the side of the square by itself

Another way:

$\frac{diagonal \times diagonal}{2}=Area~ of ~the ~square$

For more information, enter the link of Area of a square

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

Indicate the correct answer

The next quadrilateral is:

Question 2

Indicate the correct answer

The next quadrilateral is:

Question 3

Indicate the correct answer

The next quadrilateral is:

Area of the Rectangle

$a\times b=Area~of~the~rectangle$

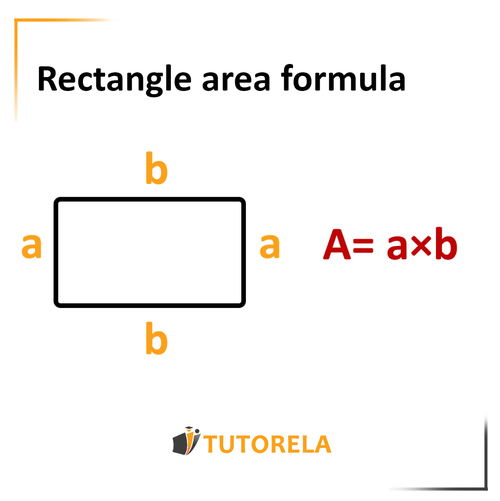
We will multiply one side of the rectangle by the adjacent side (the side with which it forms a $90^o$ degree angle)

For more information, enter the link of Rectangle area

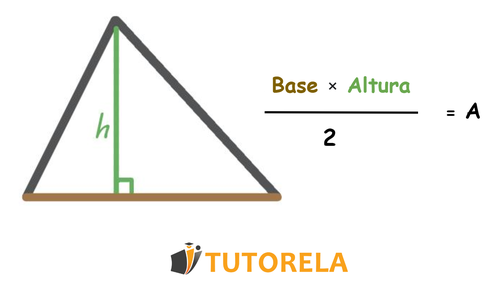
Area of the triangle

$\frac{height~\times corresponding~side}{2}=Area~ of ~the ~triangle$

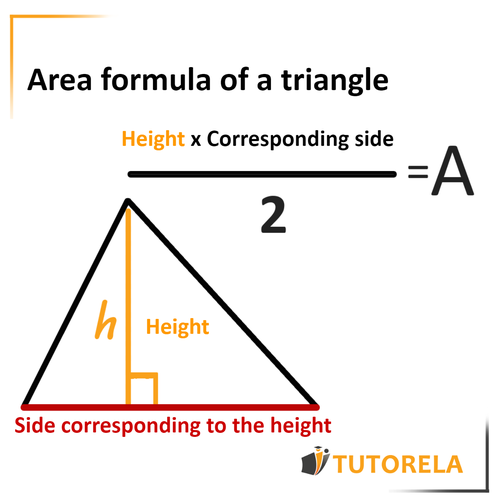
We will multiply the height by the corresponding side - that is, the side with which it forms a $90^o$ degree angle and divide the product by $2$ .

For more information, enter the link to Triangle Area

Do you know what the answer is?

Question 1

Indicate the correct answer

The next quadrilateral is:

Question 2

Indicate the correct answer

The next quadrilateral is:

Question 3

Indicate the correct answer

The next quadrilateral is:

Area of the Rhombus

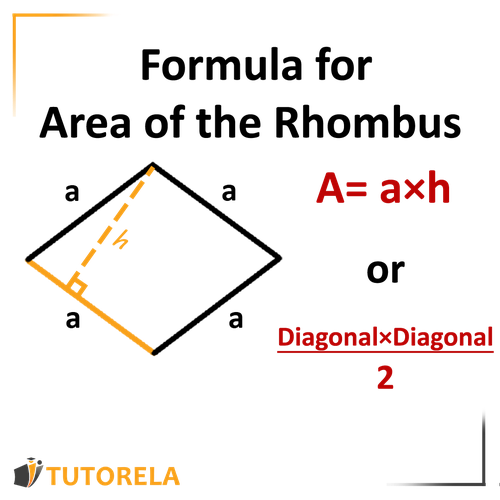
$a$ –> Side of the rhombus
$h$ –> Height

$a\times h= Area~ of ~the~ rhombus$

We will multiply the height by the corresponding side, that is, the side with which it forms a right angle of $90^o$ degrees.

Another way :

$\frac{diagonal\times diagonal}{2}=Area~ of~ the~ rhombus$

For more information, enter the link of Rhombus area

Area of the parallelogram

$H$ –> Height
$B$ –> The side that forms a $90^o$ degree angle with the height $H$ .

We will multiply the height by the side to which the height reaches and forms with it a $90^o$ degree angle.

$B\times H=Area~ of ~the~ parallelogram$

For more information, enter the link of Parallelogram area

Check your understanding

Question 1

Indicate the correct answer

The next quadrilateral is:

Question 2

Indicate the correct answer

The next quadrilateral is:

Question 3

Indicate the correct answer

The next quadrilateral is:

Area of the Circle

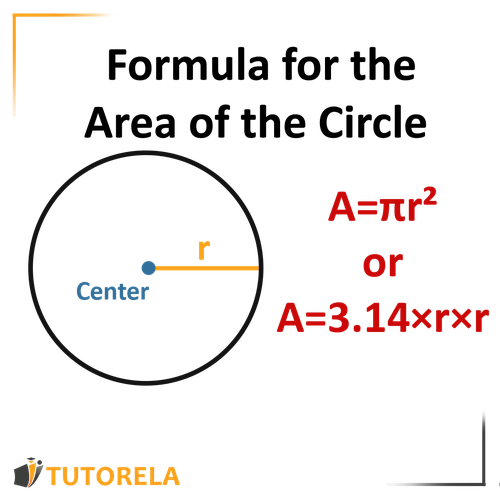
$r$ The radius of the circumference
$π$ PI
It will be calculated as the number $3.14$

$π\times r^2=Area~ of ~the ~circle$

We will multiply PI $3.14$ by the radius of the circumference squared, that is $r^2$
Or, more simply, the formula is:

$r\times r\times 3.14=Area~ of ~the ~circle$

For more information, enter the link of Circle area

Area of the trapezoid

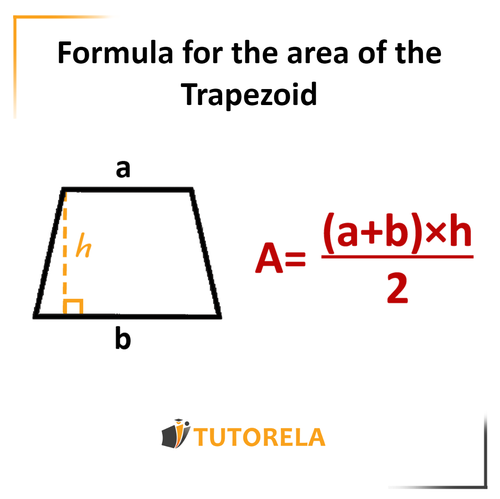
We will add the bases and multiply the result by the height of the trapezoid.
We will divide the result by $2$ .

$\frac{(a+b)\times h}{2}=Area~ of~ the~ trapezoid$

For more information, enter the link of Trapezoid area

Do you think you will be able to solve it?

Question 1

Indicate the correct answer

The next quadrilateral is:

Question 2

Indicate the correct answer

The next quadrilateral is:

Question 3

Indicate the correct answer

The next quadrilateral is:

Area of the Kite

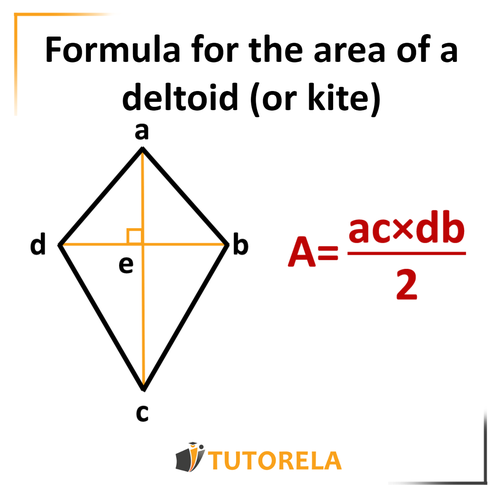
We will multiply the diagonals and divide by $2$ .

$\frac{ac\times db}{2}=Area~ of~ the~ trapezoid$

For more information, enter the link of Area of the kite

Area of Composite Figures

You don't have to worry about this pair of terms - composite figures. They are not called composite because they are complicated or difficult, but rather, they are composite figures because they are really made up of several figures that you already know.
The great key to calculating the area of this type of figures is to separate them into several simple figures on which you know how to calculate their area.

Let's look at an example

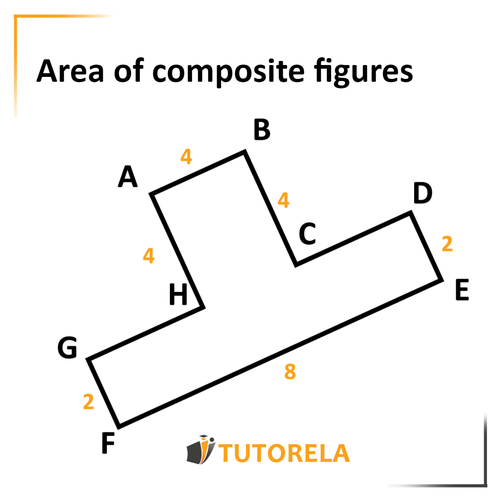
At first glance, it might scare us a bit since the figure seems very strange. But, very quickly we will remember the suggestion that we have written here above and apply it.
We will realize that we can divide the composite figure into two that we know and know how to calculate their area, rectangle and square.
We will calculate the area of each figure separately and then add them together.
In this way, we will obtain the area of the entire figure.

Test your knowledge

Question 1

Indicate the correct answer

The next quadrilateral is:

Question 2

A parallelogram has a length equal to 6 cm and a height equal to 4.5 cm.

Calculate the area of the parallelogram.

Question 3

Complete the sentence:

To find the area of a right triangle, one must multiply ________________ by each other and divide by 2.

What is the difference between surface area and volume?

To understand the difference, let's remember a daily term we use in another context: superficial.
Superficial implies something or someone without depth, so, in geometry, the surface indicates the size of something flat, without depth. For example, if we draw a ball and paint it, that painted part would be its surface.
On the other hand, volume refers to the actual size of the ball, the space that we could fill inside it.
Volume is not the surface on the sheet of paper, but, really the size we can see (in a three-dimensional way) - the space it occupies in space.
The calculation of volume differs from the calculation of the surface.

A10 - Volume of the cylinder and Volume of the cube

Examples and exercises with solutions for area calculation

Exercise #1

Complete the sentence:

To find the area of a right triangle, one must multiply ________________ by each other and divide by 2.

Step-by-Step Solution

To solve this problem, begin by identifying the elements involved in calculating the area of a right triangle. In a right triangle, the two sides that form the right angle are known as the legs. These legs act as the base and height of the triangle.

The formula for the area of a triangle is given by:

A = \frac{1}{2} \times \text{base} \times \text{height}

In the case of a right triangle, the base and height are the two legs. Therefore, the process of finding the area involves multiplying the lengths of the two legs together and then dividing the product by 2.

Based on this analysis, the correct way to complete the sentence in the problem is:

To find the area of a right triangle, one must multiply the two legs by each other and divide by 2.

Answer

the two legs

Exercise #2

Indicate the correct answer

The next quadrilateral is:

Video Solution

Step-by-Step Solution

Initially, let us examine the basic properties of a deltoid (or kite):

A quadrilateral is classified as a deltoid if:

It has two distinct pairs of adjacent sides that are equal in length.

In the question's image, we observe the following:

There are lines connecting A to B, B to C, C to D, and D to A, suggesting a typical quadrilateral.
The shape, given its central symmetry (as it is formed by joining these particular points which extend equal lines), is reminiscent of a symmetric or bilaterally mirrored formation.
Given the symmetry, it suggests all internal angles are less than 180 degrees, confirming the figure as a convex shape.

From this analysis, the quadrilateral satisfies the characteristic of having pairs of equal adjacent sides which confirms it as a deltoid. The symmetry suggests it is not concave (which occurs when at least one interior angle is greater than 180 degrees).

Therefore, the given quadrilateral, based on its properties and symmetry, is a convex deltoid.

Answer

Convex deltoid

Exercise #3

Indicate the correct answer

The next quadrilateral is:

Video Solution

Step-by-Step Solution

To solve this problem, let's analyze the given quadrilateral ABCD by examining its geometric properties:

Step 1: Identifying characteristics of a deltoid
A deltoid, or kite, is a quadrilateral that has two distinct pairs of adjacent sides that are equal. To classify a shape as a deltoid, we need to verify these properties.
Step 2: Examining the quadrilateral ABCD
The deltoid can be either concave or convex. If the shape is concave, it will have an indentation, meaning at least one angle is greater than $180^{\circ}$ . A convex deltoid does not have such an indentation.
Step 3: Analyze the sides of ABCD
Looking at the segments from the given points:
- Verify if pairs of adjacent sides are equal.
If we cannot find two equal pairs of adjacent sides, the quadrilateral is not a deltoid.
Step 4: Drawing conclusions
Having analyzed the sides of the quadrilateral, if none of the pairs of adjacent sides conform to the deltoid property as outlined—two pairs of equal adjacent sides—then ABCD is identified as not a deltoid.

Therefore, the correct answer is: Not deltoid.

Answer

Not deltoid

Exercise #4

Indicate the correct answer

The next quadrilateral is:

Video Solution

Step-by-Step Solution

To solve this problem, let's analyze the quadrilateral depicted:

Step 1: Analyze the given quadrilateral's shape using its geometric features, noting potential symmetry and side equivalence.
Step 2: Identify if the quadrilateral fulfills the characteristics of a deltoid, which involve pairs of adjacent sides being equal.
Step 3: Determine if it is possible to accurately categorize the quadrilateral as a convex or concave deltoid based on the given image and without explicit measurements.
Step 4: In the absence of direct measurable evidence, consider if categorization is feasible.

Assessing visuals alone can lead to assumptions about equal lengths or angles, but without numerical data, it's challenging to make definitive geometrical claims about sides or symmetry.

Given these limitations, it is reasonable to conclude that we cannot definitively prove whether the quadrilateral is a deltoid (convex or concave) using just the visual representation provided.

Therefore, the solution to the problem is "It is not possible to prove if it is a deltoid or not."

Answer

It is not possible to prove if it is a deltoid or not

Exercise #5

Indicate the correct answer

The next quadrilateral is:

Video Solution

Step-by-Step Solution

The problem requires determining if a given quadrilateral is a deltoid, and if so, whether it is convex, concave, or indeterminate based on the provided diagram. A deltoid, or kite, is generally defined as a quadrilateral with two pairs of adjacent sides being of equal length. Thus, a visual analysis is essential here as only diagrammatic data is available.

To address this, one must closely analyze the properties of the given quadrilateral in terms of similarity and its symmetry relative to a conventional deltoid structure:

Typically, you'd look for simultaneous symmetry or patterns indicating two equal-length adjacent pairs of sides.
After examining the diagram and the naming convention (vertices labelled A, B, C, D), see if it implies any such congruency visually or through label symmetry.
Lack of distinct clues for equal side pairs or diagonals prevents concluding its specific nature without additional information, especially since no specific length measures or angles are provided.

Given this and under diagram-only conditions, it's not possible to definitively prove that the shape is completely a deltoid (convex or concave). Therefore, without further data, identifying the indicated quadrilateral deltoid nature is beyond determining from the given data itself.

Consequently, the correct answer is: It is not possible to prove if it is a deltoid or not.

Answer

It is not possible to prove if it is a deltoid or not

Do you know what the answer is?

Question 1

Indicate the correct answer

The next quadrilateral is:

Question 2

Indicate the correct answer

The next quadrilateral is:

Question 3

Indicate the correct answer

The next quadrilateral is:

Start practice

Area

What is the area?

Units of measurement of area

Test yourself on area of the square!

Area

Area of the Square

Area of the Rectangle

Area of the triangle

Area of the Rhombus

Area of the parallelogram

Area of the Circle

Area of the trapezoid

Area of the Kite

Area of Composite Figures

Let's look at an example

What is the difference between surface area and volume?

Examples and exercises with solutions for area calculation

Exercise #1

Step-by-Step Solution

Answer

Exercise #2

Video Solution

Step-by-Step Solution

Answer

Exercise #3

Video Solution

Step-by-Step Solution

Answer

Exercise #4

Video Solution

Step-by-Step Solution

Answer

Exercise #5

Video Solution

Step-by-Step Solution

Answer

More Questions

Area of a Triangle

Area of a Parallelogram

Area of a Rectangle

Area of a Trapezoid

Area of the Square

Area of a Deltoid

Area of a Circle

Area of a Rhombus