The Area of a Rhombus

The first formula to calculate the area of a rhombus.

$Area=\frac{Diagonal_1\times Diagonal_2}{2}$

The second formula to calculate the area of a rhombus.

$Area=Height\times Side$

Below is a variety of exercises to calculate the area of a rhombus:

• Diagonal $a=8$
• Diagonal $b=13$

Question:

What is the area of the rhombus?

Explanation

The calculation is done as follows: $13\times8=104$. Now, the amount should be divided by $2$, and then the obtained answer is $52$. So, you will calculate the area of the rhombus according to the data presented to you in the question.

Answer:

$Area = 52$

Diagonal $a=2$
Diagonal $b=5$

Question:

What is the area of the rhombus?

Explanation
The area of the rhombus $=2\times5=10$.

Now we divide this result by $2$

Answer

The area of the rhombus is $5$.

Diagonal $a=6$
Diagonal $b=4$

Question:

What is the area of the rhombus?

Explanation
The area of the rhombus $=6\times4=24$.

Then we divide by $2$

Answer

The area of the rhombus is therefore $12$.

Calculating the area of a rhombus using the formula of base x Height:
Base $=4$

Height $=2$

Question:

What is the area of the rhombus?

Explanation

The area of the rhombus $=4\times2=8$

Answer

The area of the rhombus is then $8$.

Calculating the area of a rhombus using the formula of base x Height:

Base $=7$
Height $=3$

Question:

What is the area of the rhombus?

Explanation
The area of the rhombus $=7\times3=21$

Answer: $21$

Question:

Find the value of $X=?$

Explanation:

To solve the exercise, we must use the formula for the area of a rhombus, and solve it "backwards".

We will input the data we know into the formula:

$\frac{5\times X}{2}=40$

We'll start by removing the denominator, multiplying the equation by $2$.

$80=X\times5$

Now, we need to isolate $X$. To do this, we divide the equation by $5$.

$X=16$

Answer

The area of the rhombus is then $X=16$.

Question:

What is the area of a rhombus?

Explanation:

To find the solution, we'll need to use another tool to find the missing diagonal.

We know that the diagonals of a rhombus are perpendicular to each other and divide the rhombus into four right triangles.

Therefore, we can examine our triangle and use the Pythagorean theorem to find the missing side.

$A^2+B^2=C^2$

We'll plug in the data we know into the Pythagorean theorem:

$3^2+X^2=5^2$

$9+X^2=25$

Now we'll construct the exercise according to the rules, to solve for $X$.

$25-9=X^2$

$X^2=16$

Now we'll apply the square root to the equation to eliminate the powers

$X=4$

We haven't reached the solution to the exercise yet!

Now that we have found the missing side, we can use the formula for the area of a rhombus to find its area.

(Diagonal A $\times$ Diagonal B): 2

It's important to remember that the data we have is not for the full diagonals, but only from the intersection point of the diagonals to the vertices.

We know that the diagonals of a rhombus also bisect each other, therefore:

Diagonal $1=3\times2=6$

Diagonal $2=4\times2=8$

Now all that's left is to plug the numbers into the formula and solve:

$\left(6\times8\right):2$

$\frac{48}{2}$

$24$

Answer

the area is equal to $24$

If you're interested in learning how to calculate the areas of other geometric shapes, you can check out one of the following articles:

• How do you calculate the area of a trapezoid?
• How to calculate the area of a triangle
• The area of a parallelogram: what is it and how is it calculated?
• Circular area
• Surface area of triangular prisms
• How to calculate the area of a regular hexagon?
• Area of a rectangle
• How to calculate the area of an orthohedron
• Diagonals of a rhombus
• Symmetry of a rhombus
• From parallelogram to rhombus

On the Tutorela blog, you'll find a variety of articles about mathematics.

Given that the rhombus in the graphic has an area of $24^{}\operatorname{cm}^2$

Task:

What is the value of $X$?

Rhombus area formula

$\frac{diagonal1\times diagonal2}{2}$

Let's plug the data into the formula.

$\frac{8\times X}{2}=24$

$4X=24$ Now we divide by $4$

$X=6$

Finding the diagonals from the formula:

Given the drawing of the rhombus, the area is

$65^{}\operatorname{cm}^2$

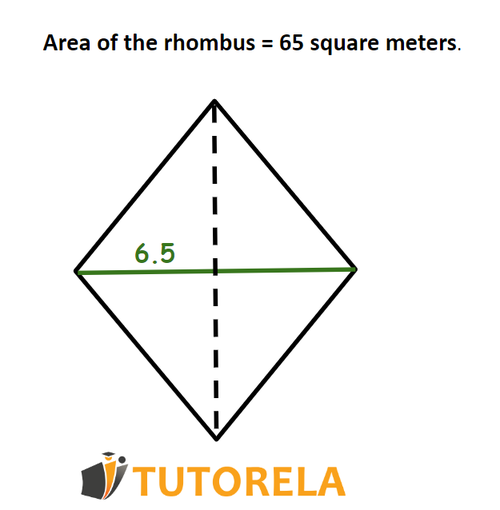
Task:

What is the length of the main diagonal in the rhombus?

Solution:

The formula for the area of a rhombus.

(Diagonal times Diagonal)/$2$

$A=\frac{Diagonal1\times Diagonal2}{2}$

When the area is $65$ cm²

We will denote a, which is the main and longer diagonal among the two.

$65=\frac{6.5\times Diagonal2}{2}$ we multiply both sides by $2$

$65\times2=6.5\times Diagonal2$ Now we divide by $6.5$

$Diagonal2=\frac{65\times2}{6.5}=\frac{130}{6.5}=20$

Answer

The length of the main diagonal in the rhombus $=20$

Homework:

What is the area of the rhombus?

Solution:

A rhombus whose pair of adjacent angles equals $90^o$ degrees is a square,

Remember that the area of a square can be calculated using the formula:

Side $\times$ Side = square

We will put the numbers in the formula and solve

$7\times7=49$

Answer:

The area of the rhombus or the square $=49$

Given the drawing of the rhombus, we have the following data as shown $3$ and $5$

Task:

What is the area of the rhombus?

Solution:

To find the solution, we will need to use another tool to get to the missing diagonal.

We know that the diagonals of the rhombus are perpendicular to each other and divide the rhombus into four right triangles.

Therefore, we can look at our triangle and use the Pythagorean theorem to find the missing side.

$A^2+B^2=C^2$

We will present the data we know in the Pythagorean theorem:

$3^2+X^2=5^2$

$9+X^2=25$

Now we will move the numbers to "isolate" the $X$.

$25-9 = X^2$

$X^2 = 16$

We apply the square root to the equation to get rid of the power.

$X=4$

This is still not the solution to the exercise!

Now that we have found the missing side, we can use the formula for the area of a rhombus to find its area.

(Diagonal 1 $\times$ Diagonal 2)

It is important to remember that the data we have is not for all the diagonals, but only from the perspective of the diagonals to the vertices.

We know that the diagonals of the rhombus also intersect each other, therefore

Diagonal 1 $=3\times2=6$

Diagonal 2 $=4\times2=8$

Now we just have to put it in the formula and solve:

$\frac{(6\times8)}{2}$

$\frac{48}{2}$

$24$

Answer:

The area is equal to $24$

Given the drawing of the rhombus

Question:

What is the length of the secondary diagonal?

Solution:

Calculate the area of the rhombus

Area of the rhombus = side X Height

$6\cdot8=48$

It's important to remember that there is another formula to calculate the area of a rhombus

Area of the rhombus = (Diagonal 1 $\times$ Diagonal 2) / $2$

$Area=\frac{d2\times d1}{2}$ we multiply by $\times 2$ (where $d1$ and $d2$ are the diagonals)

$2Area=d2\times d1$; We divide both sides by $d1$

$\frac{2Area}{d1}=d2$

We will introduce the area and the first diagonal into the formula and solve:

$d2=\frac{2Area}{d1}=\frac{2\times48}{6}=\frac{96}{6}=16$

Answer:

The correct answer is $16$ cm.

Topic: Calculating the area of a rhombus using the ratio

Given the rhombus in the figure:

Given that the ratio between the length of the major diagonal and the minor diagonal is $9:2$

Task:

Find the area of the rhombus

Solution:

From the data that the ratio between the diagonals:

Diagonal1 / Diagonal2 $=\frac{9}{2}$

In the given figure for diagonal 2 (the shorter one) we will establish its length in the formula we find.

(Multiplying diagonally) $\frac{9}{2}=\frac{Diagonal1}{4}$

$36=4\times9=2\times Diagonal1$ We will divide by /$:2$

$18=\frac{36}{2}=Diagonal 1$

Let's calculate the area of the rhombus:

$A=\frac{Diagonal 1\times Diagonal 2}{2}=\frac{4\times18}{2}=\frac{72}{2}=36$

Answer:

The answer is $36$ cm²

The answer is yes and no. On one hand, they can prepare for the test in the best possible way, but still make small calculation errors (mainly due to stress), which will cause them to lose some points. In front of you, there are some tips that will significantly help your chances of getting a $100$ on a math test or exam. The key to success: perseverance!

• Attendance to math classes at school - essential! Self-study cannot replace learning the material from your teachers. If you have missed a class, make sure to catch up on the taught topic as quickly as possible.
• Doing homework consistently increases your chances of excellence. Why? Because homework allows you to practice with a variety of exercises, face challenges, and understand what your common mistakes are. Also, in the subject of math, it can be tough in training but easier in the battle (at the exam).
• Participation in class: essential! The more you participate in class, the better. Why? Because participation keeps you alert and involved in the learning process. Suggest solutions to the questions the teacher raises and ask relevant questions about the study topics.
• Private math class: welcome! No matter what your level is, your grades, and the confidence you have in yourself. Reinforcement classes strengthen your foundations, advance you both material-wise and mentally, and allow you to reap successes and higher grades.
• Plan your learning days before the test well in advance. Prepare a weekly task board, detailing learning hours and learning topics. That way, you'll always arrive ready for the exam and even be able to plan a "study-free day" the day before the exam. The goal: to arrive at the test relaxed, calm, and at peace.

As is known, many times students memorize different formulas. For example: a formula to calculate the area of a rhombus. Instead of memorizing an "empty" formula without data, we memorize a formula with data. Why? Because this is an example of a question with an answer, which you can use both in preparing homework and during an exam. When you memorize an example of each topic, you have another "internal aid" that assists you and allows you to better understand additional questions.

One example of each topic is enough to "light the way" and enable you to solve questions quickly and easily. Sometimes, all you need to do is simply replace the data from the question (as indicated in the exam), when the solution is already well integrated in your mind.

There isn't a student who isn't good at math, but there is a student who doesn't understand math. Often, what prevents students from getting good grades is not their inability, but their lack of understanding. As is known, school curriculum lessons are conducted at a certain pace, which not all students can keep up with. In this way, the gaps gradually widen and do not narrow.

Private math tutoring can strengthen the student and provide them with a quality toolbox to continue. Unlike classes in a classroom, the private lesson focuses solely on the points where the student is weak: from the difficulty of understanding what is being asked, to the difficulty of understanding how to apply the formulas based on the data presented in the question.

Some students might feel a bit embarrassed to tell their peers about their intention to bridge gaps in a private math class. In practice, there's really no reason for embarrassment, quite the opposite: students who attend a private class get to reinforce the material taught in an extra session. Often, the result is that the same students who study in the private lesson are actually more advanced in the material than the rest of the class. The main advantages of the private lesson are:

• Bridging gaps: the student keeps up with the pace of the class.
• Assimilation of learned material in the form of "understanding" rather than "memorization"
• Strengthening the student's self-confidence: they prove to themselves that they can do it!

This is a question that does not have an unquestionable answer. Some students are interested in a private meeting once a week. However, there are students who meet with a private tutor just before tests, in order to reinforce formulas such as how to calculate the area of a rhombus, create simulations, and challenge themselves with questions that are more difficult than those expected on the test.