How is the radius calculated using its circumference?

More examples:

Given: A circle whose perimeter is $25.45$, we must calculate the radius of the circle
We will place the data we know into the formula: $25.45=2πR$
The circumference can be translated in terms of $π$, that is: $25.45=$
Then we obtain: $25.45=6.28R$

To isolate the value of $R$ divide $25.45/6.28$
Therefore, the result is that the radius of the circle $=4.05$

Given a circle with a circumference of $50.25$, we must calculate the radius of the circle
We will place the known data into the formula: $50.25=2πR$
The circumference can be expressed in terms of $π$, that is: $50.25=$
Then we obtain: $50.25=6.28R$
To isolate the value of $R$, it is necessary to divide $50.25/6.28$
Therefore, the result is that the radius of the circle $=8$

The data of a circle whose circumference is $11$, the radius of the circle must be calculated.
To isolate the value of $R$ divide $11/6.28$
Therefore, the result is that the radius of the circle $=1.75$

Exercise 1:

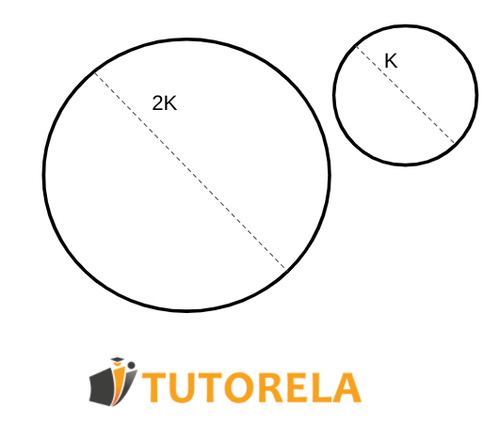
Task:

How will the circumference change if we double its diameter?

Solution:

The radius is equal to $K$

$P=2π\times r$

$=2π\times (\frac{K}{2} )$

$=2π\times (\frac{K}{2} )=\pi K$

The radius is equal to $2K$

$P=2π\times r$

$=2π\times (\frac{2K}{2} )$

$=2π\times (\frac{2K}{2} )=2 \pi \cdot K$

$\frac{P2K}{PK}=\frac{2πK}{πK}=2$

Answer:

The circumference will double

Exercise 2:

Given the shape of the figure.

The quadrilateral is a square with $5$ cm side length.

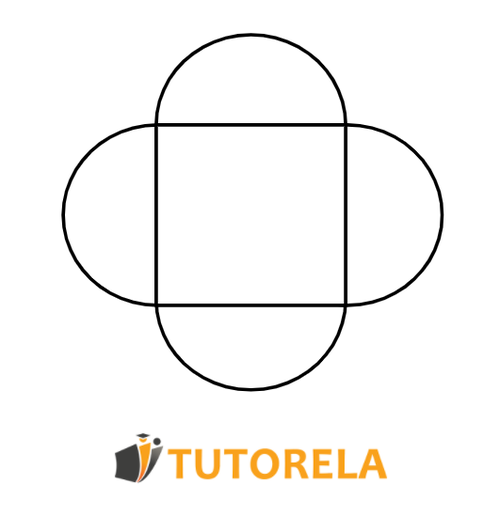
Task:

What is the perimeter of the figure?

Solution:

The perimeter is made up of $4$ halves of the circle.

$4\times\frac{1}{2}P=2P$

That is, a total of $2$ circumferences with $5$ cm diameter.

$Diameter = 2 radius$

$2\times radius=5$

$radius=\frac{5}{2}=2.5$

Circle diameter $5$ cm

$P=2π\times2.5=5π$

$P=2\times P=2\times5π=10\pi$

Answer:

$10\pi$

Exercise 3:

Given:

Mirta runs at a speed of $7$ minutes per kilometer.

She runs on a circular path with a radius of $20$ meters and circles it $4$ times.

Task:

How long will Mirta run?

Solution:

First, we calculate the length of the path.

Path length = The circumference has a radius of $20$ meters.

$2π\times20=2\times3.14\times20=125.6$

Mirta's path distance = Path length $\times4$ = $125.6\times4=502.4$ (meters)

That is: $0.5024$ km

$\text{Time}=7\times0.5024=3.52$

Answer:

It will take Mirta $3.52$ minutes to complete the run.

Exercise 4:

Given the circle with a circumference of $6.28$

Question:

What is its area?

Solution:

Let's recall the circumference formula:

$2πR$

We replace with the known data:

$6.28=2πR$

We divide by $2$

$3.14=πR$

Now we divide by $π$

$1=R$

From here we can replace the data in the area formula of the circumference:

$A=πR^2$

$A=π1^2$

$A=π\times1$

$A=π$

Answer:

$Area=π$

If you're interested in this article, you might also be interested in the following articles:

• The circumference
• The center of the circumference
• Circle
• Radius
• Diameter
• Pi
• The perimeter of the circumference
• Circular area

On the Tutorela blog, you'll find a variety of articles about mathematics.

Are you having trouble answering questions where you need to calculate the radius using the perimeter? A private tutor can help you. The role of the tutor is not only to teach you, but also to understand the reasons why you are having difficulties with a particular topic. For example:

• The difficulty in calculating the radius using the perimeter may be due to a lack of understanding of the formula.
• Partial familiarity with the properties of the radius.
• Difficulty in extracting data from the request to apply the formula.

In this way, the teacher not only teaches you but also works with you on strengthening weak areas (memorizing formulas, assimilating features of certain shapes, and more...).

On our site, you will find recommended math teachers! Here are some tips for choosing a tutor:

• Read reviews: On each teacher's page, you can read testimonials. The more recommendations there are about the teacher, the better. Through the suggestions, you will learn about the teacher's learning approach, how patient they are, how available they are, the quality of the lesson, etc.
• Choose a free time: Each teacher has a schedule in which they can book their private lessons. Keep in mind that there are teachers who are not available for a private lesson in the next week, and their next lesson will be in a week. Even if you have received assertive recommendations about a particular teacher, give priority to a teacher with high availability. The goal: to reduce gaps and prepare as much as possible for the next lesson/exam/test.
• Specify what subject you need help with: When booking the private lesson on our website, a window will open where you must indicate the topics you need help with. It is very important to specify what course you are studying, the topics learned, and the name of the subject. This way, the teacher can prepare a lesson plan and come more prepared for your joint lesson.
• Price per hour: Each tutor charges a different price for the private lesson. You should consult with your parents and choose a teacher according to the available budget.

More and more students are lamenting the way they are taught the material, but they are not explained how to study for exams. There is a big difference between solving a question in class with the teacher and with the help of classmates, and dealing with questions in real time during the exam. The secret to success? Practice the material and mentally prepare to believe in yourself, and above all, reduce feelings of stress. So, how do you prepare for the exam in the best way?

1. Start studying about a week and a half before the exam

Some schools distribute the exam schedule to students on the first day of classes. This means that you know when you will be tested, so you can prepare in advance according to your own time and priorities. A week and a half is a sufficient amount of time to study for a math exam, assuming that you have mastered most of the material and need this period just for practice and memorizing formulas.

2. Create a detailed personal learning program.

Additionally, it's worth creating a schedule for yourself that details what you will study each day of the week. It's not enough to say: "On Monday, I will study circles and radii", but you should specify exactly what you are doing:

• List the hours. For example: from 4:00 PM to 8:00 PM.
• What will you do exactly (memorize formulas, review the properties of shapes, solve exercises)?
• In just a few pages, you will progress through the workbook and so on.

3. Breaks during learning are very important

When creating a learning schedule for yourself, also incorporate breaks. If you study for hours on end and intensely, you can reach burnout quickly. The goal is to maintain your motivation and not to arrive at a pre-exam situation of exhaustion. For every two hours of study, take a fresh half-hour break.

4. Blessed Learning Groups!

Is it worth studying with friends? Yes! Just make sure that you're actually studying and it's not turning into an impromptu party. Every three days of study, use a study group with 2-3 members. The goal is to solve exercises together, while each of you can contribute your knowledge to the other members and thus strengthen certain topics. Those of you who want to simulate an exam situation can open timers and check how much time it takes to solve the exercises.

5. Come to math class with questions.

During home study, it's likely that some more challenging and complex questions will arise, which is fine. Teachers allow you to raise questions that you want them to solve in class, and it's definitely worth asking questions. Your teacher will guide you through solving the question, while you will learn how to deal with questions at this specific level during the test.

And what about a private tutor? A personalized math class can definitely contribute to your understanding. The recommendation is not to wait for private lessons only just before the exam, but to make sure you gradually assimilate the material learned throughout your studies. The private lesson can be conducted at the student's home, at the teacher's home, or online.