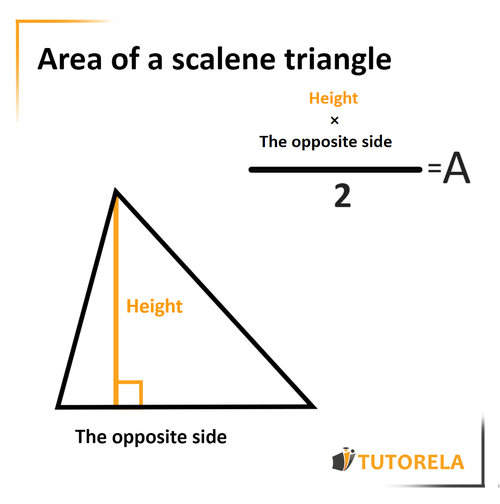
Formula to calculate the area of a scalene triangle:
Complete the sentence:
To find the area of a right triangle, one must multiply ________________ by each other and divide by 2.
It's very simple to calculate the area of a scalene triangle if we remember the formula and strictly follow the steps. Don't worry, we're here to teach you exactly what to pay attention to—we won't leave you adrift!
First of all, let's look at the formula you need to remember in order to calculate the area of the scalene triangle:

Multiply the height by the base (the side corresponding to that height) and divide by .
Pay attention:
Make sure to place in the formula the corresponding height and side. That is, if a certain height and a side that does not form a right angle of degrees with the used height is placed in the formula, it will be wrong.

Given the triangle
Given that:
Height
What is the area of the triangle?
Solution:
We will see that the given side actually forms, with the height, an angle of degrees.
After verifying the data, we will go to the formula and place there:
The area of the triangle is
Calculate the area of the following triangle:
Calculate the area of the following triangle:
Calculate the area of the triangle using the data in the figure below.
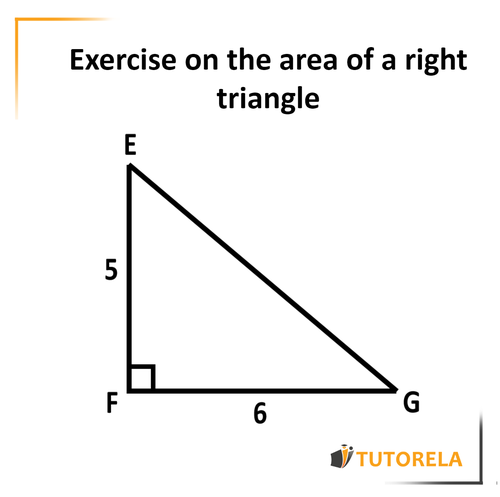
Given the right triangle
Given that:
angle
Calculate the area of the triangle.
Solution:
Let's remember that the key to calculating the area of any triangle is to multiply the height
by the corresponding side and then divide that product by
In a right triangle, we actually already have the height!
We don't need to calculate another height and, in fact, we can afford to use the given height along with the side that forms the degree angle.
In our exercise: The side is or
What conclusion do we reach?
The conclusion is that the formula to calculate the area of a right triangle is the product of the two legs divided by Let's put it in the formula and we will get:
The area of the triangle is
Calculating the area of an obtuse triangle is a bit more complicated, but I assure you that once you understand the basic principle, you will be able to calculate the area of an obtuse triangle even in your sleep...
In certain cases, in an obtuse triangle, we will be given a height that is outside the triangle.
As in the following illustration:
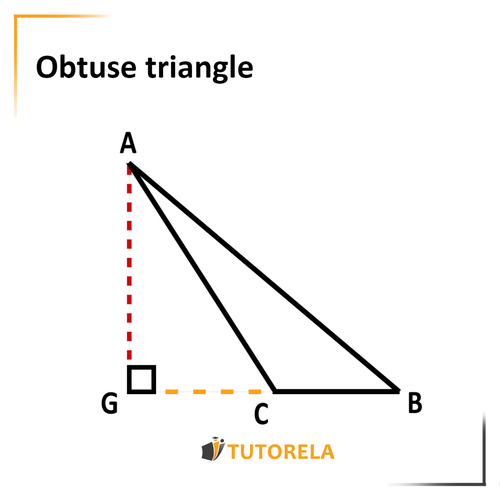
In this illustration, the height has been drawn outside of the triangle. In reality, if we were to extend the side (marked in green), it would form a right angle with the height.
How is the area of an obtuse triangle calculated?
Remember the following guidelines and you will do well:
Now let's solve an exercise so you can understand it more easily:
Given the triangle
Given that:
Height of the triangle
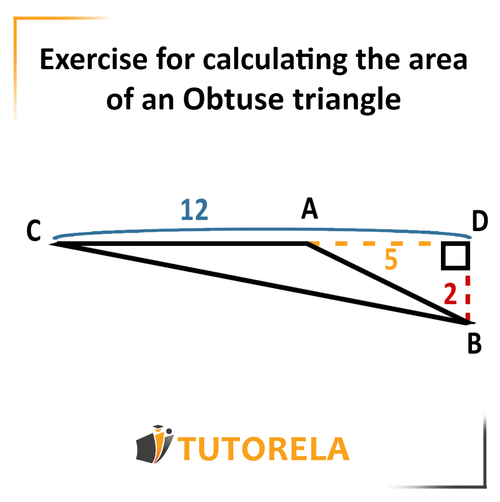
What is the area of the triangle?
Solution:
We observe that the length of the side
and the corresponding side that forms with it a degree angle (the dotted part outside the triangle) is
If we go back to the first point we needed to remember - we will understand that, to calculate the area, we must only take into account the length of without its dotted extension.
Therefore, we will see it as
And now we can safely place the data, according to the basic formula:
The area of the triangle is
Calculate the area of the right triangle below:
Due to the fact that AB is perpendicular to BC and forms a 90-degree angle,
it can be argued that AB is the height of the triangle.
Hence we can calculate the area as follows:
24 cm²
Calculate the area of the triangle ABC using the data in the figure.
First, let's remember the formula for the area of a triangle:
(the side * the height that descends to the side) /2
In the question, we have three pieces of data, but one of them is redundant!
We only have one height, the line that forms a 90-degree angle - AD,
The side to which the height descends is CB,
Therefore, we can use them in our calculation:
36 cm²
What is the area of the triangle in the drawing?
First, we will identify the data points we need to be able to find the area of the triangle.
the formula for the area of the triangle: height*opposite side / 2
Since it is a right triangle, we know that the straight sides are actually also the heights between each other, that is, the side that measures 5 and the side that measures 7.
We multiply the legs and divide by 2
17.5
Calculate the area of the following triangle:
The formula for calculating the area of a triangle is:
(the side * the height from the side down to the base) /2
That is:
We insert the existing data as shown below:
10
Calculate the area of the following triangle:
The formula for the area of a triangle is
Let's insert the available data into the formula:
(7*6)/2 =
42/2 =
21
21
Calculate the area of the triangle using the data in the figure below.
Calculate the area of the following triangle:
What is the area of the given triangle?