Before going deeper into opposite angles, we will pause a moment to visualize the types of scenarios where this type of angle can be found. To make it easier to understand, we will draw two parallel straight lines cut by a secant or transversal, as shown in the following illustration:
Vertically Opposite Angles
What are opposite angles?
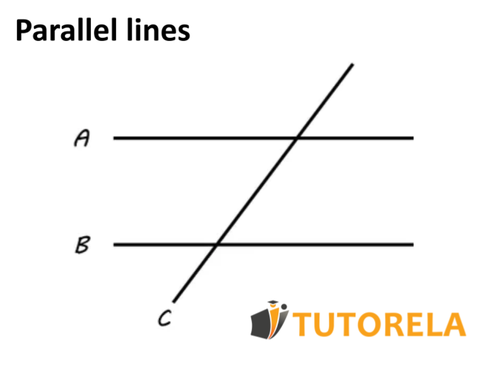
What do we see here? The transversal intersects with each one of the straight lines and (in our case and are parallel, although this is not required in order to get opposite angles).
With this example in mind, we are ready to move on to the formal definition of opposite angles, which will help us to identify them more easily:
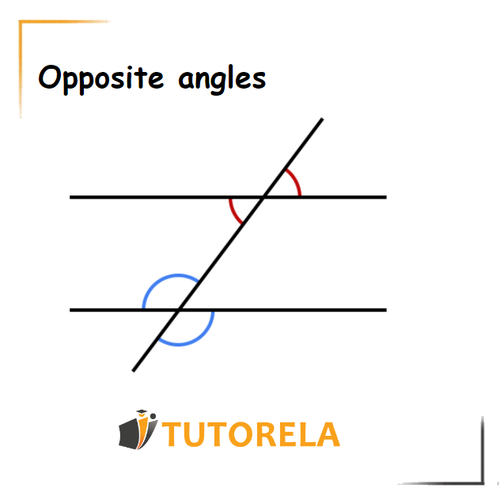
Opposite angles are a pair of angles that arise when two straight lines intersect. These angles are formed at the point of intersection (which we will call the vertex), one in front of the other. Opposite angles are equal.
In the following illustration, we can see two examples of opposite angles, the first pair is marked in red and the second pair in blue.
Test yourself on angles in parallel lines!
If one of two corresponding angles is a right angle, then the other angle will also be a right angle.
More types of angles
Opposite angles are not the only types of angles that we can find in geometric problems. Even the example seen in the introduction actually includes several other types of angles that we will mention briefly below:
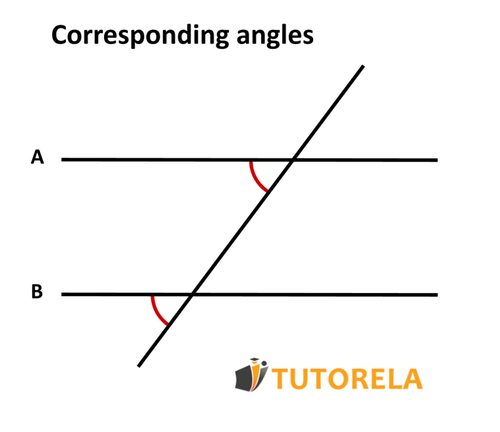
Corresponding angles
Corresponding angles are a pair of angles that can be found on the same side of a transversal intersecting two parallel lines. These angles are on the same side of the parallel lines to which they belong. Corresponding angles are equal.
If you wish to read a more extensive explanation, you can consult the article on the subject "Corresponding angles".
It is possible for two adjacent angles to be right angles.
The sum of adjacent angles is 180 degrees.
If one vertically opposite angle is acute, then the other will be obtuse.
Alternate angles
Alternate angles are a pair of angles that can be found on opposite sides of a transversal intersecting two parallel lines. These angles are on the opposite sides of the parallel lines to which they belong. Alternate angles are equal.

For a more detailed explanation, please refer to the article "Alternate angles".
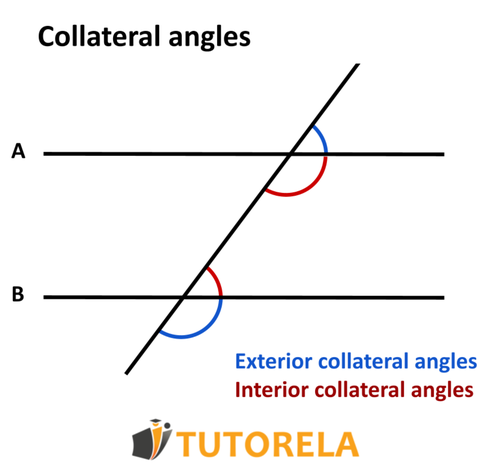
Collateral angles
Collateral angles are a pair of angles that can be found on the same side of a transversal intersecting two parallel lines. These angles are on the opposite side of the parallel lines to which they belong. The sum of the collateral angles equals .
For a more detailed explanation, please refer to the article "Collateral angles".
The lines in the figure are parallel.
Are the angles \( \alpha \) and \( \beta \) corresponding?
Does the diagram show an adjacent angle?
Does the diagram show an adjacent angle?
Exercise problems with opposite angles
Exercise 1
In each of the following diagrams, determine if the angles are opposite angles, and if not, specify the type of angle.
Scheme1:
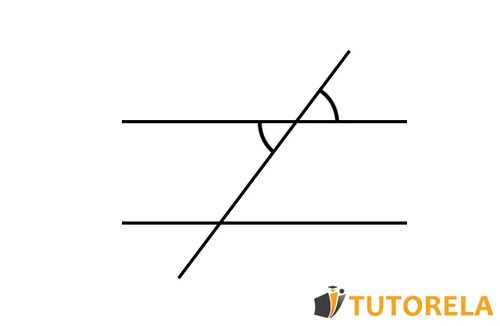
Scheme 2:

Scheme 3:

Solution:
Scheme 1:
In this scheme if we are dealing with opposite angles. The reason is that they meet the criteria, that is to say, two straight lines intersect and the opposite angles are formed at the point of intersection, one in front of the other.
Scheme 2:
In this scheme we are not dealing with opposite angles, but rather with corresponding angles. The reason is that the two angles are on the same side of the transversal that cuts the two parallel lines. Moreover, these angles are on the same side of the parallel lines to which they belong.
Scheme 3:
In this scheme we are not dealing with opposite angles either, but rather collateral angles. The reason is that the two angles are on the same side of the transversal that cuts the two parallel lines. Moreover, these angles are at the opposite side of the parallel lines to which they belong.
So:
Scheme 1: opposite angles
Scheme 2: corresponding angles
Scheme 3: collateral angles
Exercise 2

Given the parallelogram as illustrated in the diagram.
The point is the point at which the diagonals meet in the parallelogram. .
The angle measures .
The angle measures .
Based on the given information calculate the angle .
Solution:
First of all, to help us find the answer, we will label the angles as follows:
We will name the angle (measure )
We will name the angle
We will name the angle (measures )
We will name the angle (the angle we are looking for)
First, we will focus on the triangle since the angle is in it.
We will build on what we already know. The sum of the interior angles of a triangle is equal to . The angle measures according to the given information. That is, if we can find the measure of the angle then we can calculate angle .
As can be understood from the data and the illustration, the point is the intersection of the diagonals and in the parallelogram . We can see that, according to its definition, this intersection forms the opposite angles and . The opposite angles are equal, therefore .
Now we can go back to the triangle and find angle :
That is, the angle which is in fact the angle we are looking for measures .
Then:
The angle measures .
Does the drawing show an adjacent angle?
Does the drawing show an adjacent angle?
Does the drawing show an adjacent angle?
Exercise 3

Given the isosceles trapezoid as illustrated in the diagram.
The point is the point where the diagonals of the trapezoid meet. .
The angle measures .
Based on the given information, calculate the angles of the triangle. .
Solution:
First of all, to help us find the answer, we will label the angles as follows:
We will name the angle (measure )
We will name the angle (one of the angles we are looking for)
We will name the angle (one of the angles we are looking for)
We will name the angle (one of the angles we are looking for)
We will focus on triangle ABM since we must find its angles.
We will start with angle .
As we can see from the data and the illustration, the point is the intersection of the diagonals and in the trapezoid . According to its definition, this intersection point forms opposite angles and . Opposite angles are equal, therefore, .
Now we turn to another piece of information we have, the side . This implies that the triangle is an isosceles triangle. We know that in an isosceles triangle the two base angles are equal.
That is to say,
To calculate the angles, remember that the sum of the interior angles of a triangle is and that the measure of is already known.
Therefore, that gives us:
Then:
The angle (angle ) measures .
The angle (angle ) measures .
The angle (angle ) measures .
Exercise 4

What is the value of given that the lines are parallel?
Solution:
The marked angles are exterior angles to the parallel lines. We can use our knowledge of opposite angles, which share the same properties.
If we take one of the opposite angles and the angle external to the other parallel line, we see that they are actually supplementary angles and therefore are equal.
That is,
Then:
We find that the value of .
Does the drawing show an adjacent angle?
Does the drawing show an adjacent angle?
Does the drawing show an adjacent angle?
Exercise 5
Knowing that is parallel to , find the value of .

Solution:
We have that the angle as it is opposite to angle . The same is true for the angle .
Then, due to the parallel lines we get: that is , .
Then solving for the value of we get:
Therefore:
Review questions:
What are opposite angles?
They are angles that are formed when two straight lines intersect, one in front of the other just at the point of intersection.
What is the main characteristic of opposite angles?
The main characteristic is that opposite angles are equal.
In an illustration of parallel lines cut by a transversal, which pair of angles have the same property of the opposite angles, that is, that they are equal?
The corresponding angles and the alternate angles.
If you are interested in learning more about other angles, you can visit one of the following articles:
- Angle notation
- Sides, vertices, and angles
- Bisector
- Right angles
- Adjacent angles
- Alternate angles
- Corresponding angles
- Sum of the angles of a triangle
- Sum of the angles of a polygon
On Tutorela you will find a variety of articles about mathematics.
Does the drawing show an adjacent angle?
Does the drawing show an adjacent angle?
If one of two corresponding angles is a right angle, then the other angle will also be a right angle.
Examples with solutions for Vertically Opposite Angles
Exercise #1
Does the drawing show an adjacent angle?
Video Solution
Step-by-Step Solution
Adjacent angles are angles whose sum together is 180 degrees.
In the attached drawing, it is evident that there is no angle of 180 degrees, and no pair of angles can create such a situation.
Therefore, in the drawing there are no adjacent angles.
Answer
Not true
Exercise #2
Does the drawing show an adjacent angle?
Video Solution
Step-by-Step Solution
Adjacent angles are angles whose sum together is 180 degrees.
In the attached drawing, it is evident that there is no angle of 180 degrees, and no pair of angles can create such a situation.
Therefore, in the drawing there are no adjacent angles.
Answer
Not true
Exercise #3
Which type of angles are shown in the figure below?
Step-by-Step Solution
Alternate angles are a pair of angles that can be found on the opposite side of a line that cuts two parallel lines.
Furthermore, these angles are located on the opposite level of the corresponding line that they belong to.
Answer
Alternate
Exercise #4
Which type of angles are shown in the diagram?
Step-by-Step Solution
First let's remember that corresponding angles can be defined as a pair of angles that can be found on the same side of a transversal line that intersects two parallel lines.
Additionally, these angles are positioned at the same level relative to the parallel line to which they belong.
Answer
Corresponding
Exercise #5
Identify the angles shown in the diagram below?
Step-by-Step Solution
Let's remember that vertical angles are angles that are formed when two lines intersect. They are are created at the point of intersection and are opposite each other.
Answer
Vertical
More Questions
Angles in Parallel Lines
- Adjacent Angle Identification: Analyzing Line Intersection Diagram
- Adjacent Angles: Identifying Intersecting Lines in Geometric Diagram
- Adjacent Angles: Analyzing Intersecting Lines in Geometric Diagram
- Adjacent Angles: Analyzing Line Segment Diagram for Angle Properties
- Adjacent Angle Identification: Analyzing Intersecting Line Segments
- Area
- Trapezoids
- Area of a trapezoid
- Perimeter of a trapezoid
- Parallelogram
- The area of a parallelogram: what is it and how is it calculated?
- Perimeter of a Parallelogram
- Kite
- Area of a Deltoid (Kite)
- Parallel lines
- Rectangle
- Calculating the Area of a Rectangle
- The perimeter of the rectangle
- Congruent Rectangles
- The sides or edges of a triangle
- Triangle Height
- Sides, Vertices, and Angles
- Angle Notation
- Angle Bisector
- Types of Angles
- Right angle
- Acute Angles
- Obtuse Angle
- Plane angle
- Sum and Difference of Angles
- Sum of Angles in a Polygon
- The Sum of the Interior Angles of a Triangle
- Perpendicular Lines
- Exterior angles of a triangle
- Perimeter
- Triangle
- Types of Triangles
- Obtuse Triangle
- Equilateral triangle
- Identification of an Isosceles Triangle
- Scalene triangle
- Acute triangle
- Isosceles triangle
- The Area of a Triangle
- Area of a right triangle
- Area of Isosceles Triangles
- Area of a Scalene Triangle
- Area of Equilateral Triangles
- Perimeter of a triangle
- Areas of Polygons for 7th Grade
- Right Triangle
- Area of a right-angled trapezoid
- Area of an isosceles trapezoid
- Median in a triangle
- Center of a Triangle - The Centroid - The Intersection Point of Medians
- How do we calculate the area of complex shapes?
- How to calculate the area of a triangle using trigonometry?
- How do we calculate the perimeter of polygons?
- All terms in triangle calculation
- Types of angles (right, acute, obtuse, straight)









