Height in a triangle

In this article, we will learn everything you need to know about medians in a triangle! Don't worry, the material about medians in a triangle is both easy and straightforward to understand.

A median in a triangle is a line segment that extends from a vertex to the midpoint of the opposite side, dividing it into two equal parts.
Remember that "median" in real life represents the middle point, and similarly here it divides the side in the middle!

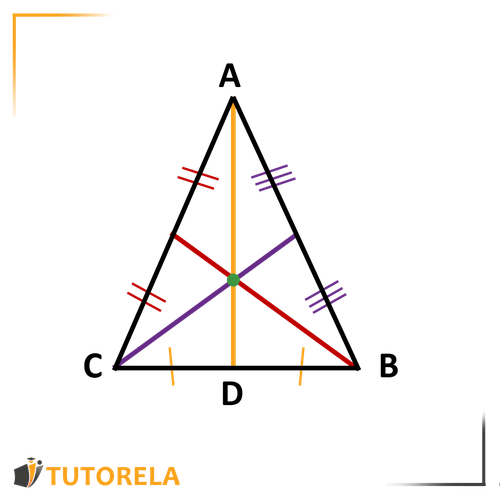
We can observe this in the following drawing:

In triangle $ABC$
$AD$ is a median - it extends from the vertex $A$ and divides the opposite side $CB$ into two
equal parts: $CD=BD$

Additional Properties of a Median in a Triangle:

1. In every triangle, it is possible to draw 3 medians.
2. All 3 medians intersect at one point.
3. The median to a side of a triangle creates 2 triangles of equal area.

You can observe this below:

Since there are 3 vertices in a triangle, there can be 3 medians.
Each median extends from a vertex to the opposite side and bisects it.
All medians intersect at one point.

Reminder:
How do we calculate the area of a triangle?

$height*corresponding~side~to~height \over 2$

If we take for example the triangle $ABC$ and want to calculate its area when:
$AD$ height = $6$
$BC = 8$

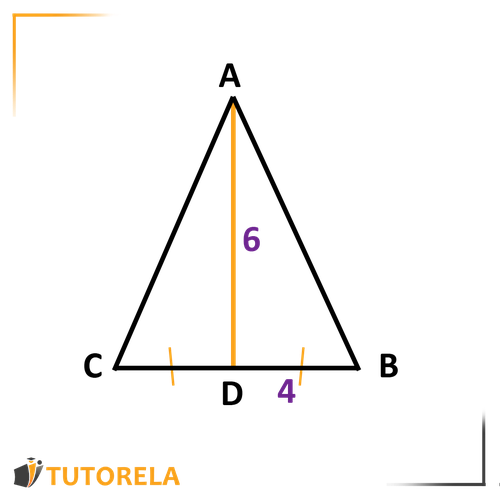
We can deduce that the area of triangle $ABC$ is:
$\frac{6*8}{2}=24$
Now if we draw the median $AD$ we can observe that the two triangles it creates are equal in area.
The side is divided in the middle thus it is identical in both triangles and the height is identical.
Therefore, the area of each created triangle is identical and will be equal to half the area of triangle $ABC$

$\frac{6*4}{2}=12$

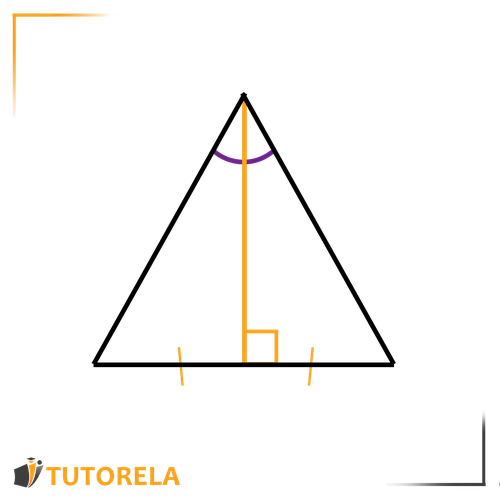
1. In an equilateral triangle - the median is also a height and an angle bisector.
   As shown in the figure:
   
   $AD$ is a height to side $CB$
   and also a median to side $CB$ (divides it into two equal parts)
   as well as an angle bisector

$A$

1. In an isosceles triangle - the median drawn from the vertex angle is also a height and an angle bisector.
   Let's observe this in the figure below:
   

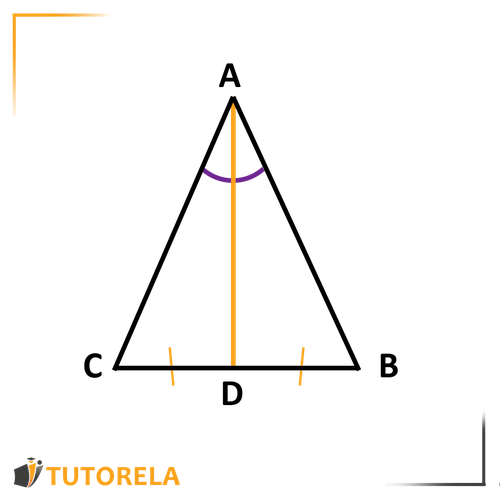
$AD$ is a median drawn from vertex angle $A$.
It is also a height to side $CB$, as well as a median to $CB$, in addition to bisecting the vertex angle $A$.

3. In a right triangle - the median to the hypotenuse equals half the hypotenuse.

We can observe this in the figure below:

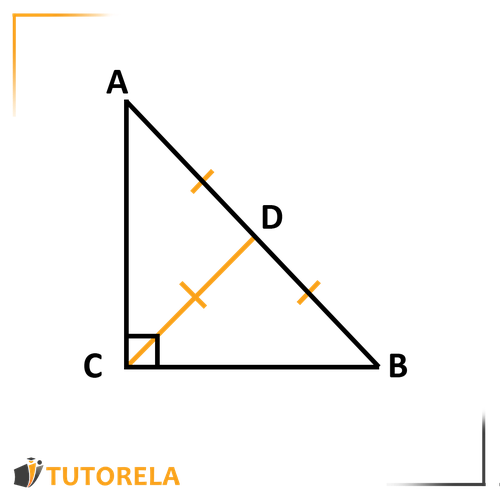
Triangle $ABC$ is a right triangle.
$CD$ is the median to the hypotenuse and equals half of the hypotenuse.
That is
$CD=AD=DB$

Given:

$CD$ is a median in triangle $ABC$
$CE$ is a median in triangle $ACD$

$AB=14$

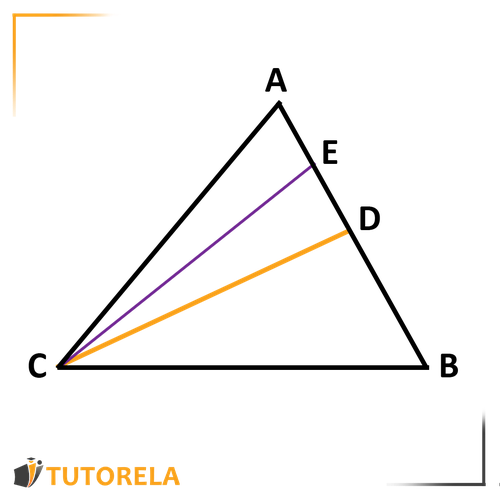
1. Calculate the length of segment $AE$.
2. Determine the area of triangle $ECD$ if it is known that the area of triangle $ACE$ is $6$?
3. Based on your answer in part b, determine the area of triangle $DCB$

Solution:

1. Let's mark the given information in the drawing.
   

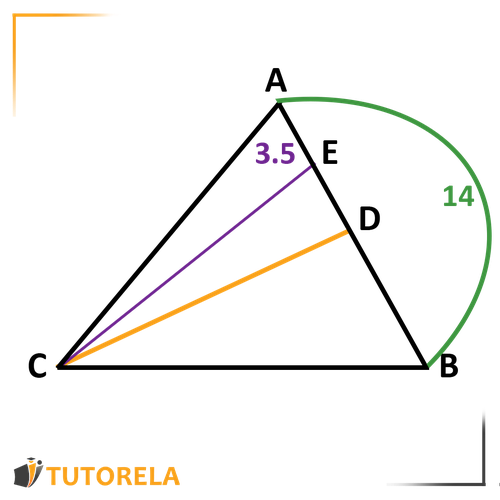
Given that – $AB =14$
Since $CD$ is a median,
$AD=DB=7$
due to the fact that the median bisects the side at its midpoint.
Given that $CE$ is also a median.
Therefore $AE=ED=3.5$.

2. Given that the area of triangle $ACE$ is $6$
The area of triangle $ECD$
must also be $6$. A median divides the triangle into two triangles of equal area.

3. The area of triangle $DCB$ must be equal to the area of triangle $ACD$.

Triangle $ACD$ consists of two triangles with equal areas that sum up to $12$.
Therefore, the area of triangle $DCB$ is $12$.