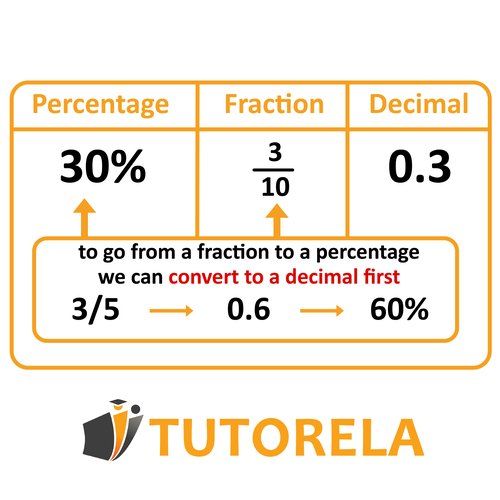
Converting between fractions and percentages and vice versa

In this article, we will teach you how to quickly convert fractions to percentages and percentages to fractions with ease. All you need to do is follow the steps and be proficient in expanding and reducing fractions.

Let's convert from percentage notation to fraction notation.
In the numerator, we write the given percentage number (without the percentage sign)
and in the denominator, we always write the number $100$.
For example:
$45\%$ will be written as $45 \over 100$.
Although we have succeeded in converting it to a fraction, this is not the final answer and we must continue to the second stage.

We must reduce the fraction we received as much as possible in order to obtain the final answer.
For example:
We reduce the fraction we received by $5$.
$\frac{9}{20}=\frac{45}{100}$
$\frac{9}{20}$ is the final answer.

Reminder - How to Reduce Fractions?
We perform the same division operation on both the numerator and the denominator - using a number that divides evenly into both. We do this until we obtain a fraction where no number can be found that divides into both the numerator and the denominator without leaving a remainder .

Convert $25\%$ to a fraction

Solution:
According to the first step, we write the percentage number in the numerator and write $100$ in the denominator.
We obtain the following $25 \over 100$
According to the second step, we reduce the fraction as much as possible.
We divide by $25$ and obtain:
$\frac{1}{4}=\frac{25}{100}$

The final answer is $1 \over 4$.

Another exercise:
Convert $67\%$ to a fraction

Solution:
We'll write $67$ in the numerator and $100$ in the denominator
We obtain the following:
$67 \over 100$
$67$ is a prime number - divisible only by itself and $1$ and $100$ is not divisible by $67$.
Therefore, we cannot reduce the fraction and the final result remains $67 \over 100$ .

Additional exercise:
Convert $225\%$ to a fraction

Solution:
Let's write $225$ in the numerator and $100$ in the denominator.
Note - don't get confused. Even if the number is large/small, we always write $100$ in the denominator.
We obtain the following:
$225 \over 100$
Now let's move to the second step and reduce the fraction as much as possible.
We can reduce in several steps in order to avoid mistakes.
First, let's reduce by $5$.
We obtain the following:
$\frac{225}{100}=\frac{45}{20}$
Notice that we can reduce the fraction even more. Let's reduce it again by $5$ and we obtain:
$\frac{45}{20}=\frac{9}{5}$
Let's convert our result to a mixed number as follows:
$\frac{9}{4}=2\frac{1}{4}$
The final result is $2\frac{1}{4}$.

We will expand or reduce the fraction so that its denominator will be the number $100$.
We will make sure to perform the expansion / reduction operation on both the numerator and denominator.
For example-
If we have the fraction $3 \over 25$ we will expand it by $4$ and obtain - $12 \over 100$

After we obtain a fraction with a denominator of $100$, we can write the numerator as a percentage and that will be the final answer!
For example –
After expanding we obtained the fraction $12 \over 100$.
The final answer will be $12\%$.

Pay attention!! Not every fraction can be converted to percentages (without a calculator). Not every given denominator can be converted to $100$ through expansion or reduction.

Convert the fraction $4 \over 5$ to a percentage

Solution:
According to the first step, the denominator must be $100$. To do this, we will expand the fraction by $20$.
We obtain the following $\frac{80}{100}=\frac{4}{5}$
According to the second step, the final answer is $80\%$.

Convert the fraction $10 \over 11$ to a percentage

Solution:
We cannot convert the denominator from $11$ to $100$ without the aid of a calculator.

Convert the fraction $60 \over 50$ to a percentage

Solution:
We expand by $2$
We obtain the following
$\frac{120}{100}=\frac{60}{50}$
The answer is $120\%$.

Convert $67\%$ to a simple fraction.

Solution:
Let's perform a simple division and obtain: $67\%=\frac{67}{100}$.

Convert the fraction $7 \over 20$ to a percentage.

Solution:
We'll expand the denominator to $100$ : $\frac{7}{20}=\frac{35}{100}=35\%$.

Let's convert the fractions $\frac {1}{2}, \frac {3}{4}, \frac {5}{20}, \frac {1}{25},$ to percentages.

Solution:
In each case we'll multiply by $100$ as follows:

$\frac {1}{2}*100=50\%$

$\frac {3}{4}*100=75\%$

$\frac {5}{20}*100=20\%$

$\frac {1}{25}*100=4\%$

Let's convert from percentages to fractions $2\%, 10\%, 35\%, 70\%,63\%$

Solution:
In each case we divide by $100$ as follows:

$2 \% = \frac{2}{100}= \frac{1}{50}$

$10 \% = \frac{10}{100}= \frac{1}{10}$

$35 \% = \frac{35}{100}= \frac{7}{20}$

$70 \% = \frac{70}{100}= \frac{7}{10}$

$63 \% = \frac{63}{100}$