The denominator is the bottom number of a fraction and represents the whole in its entirety.
For example:
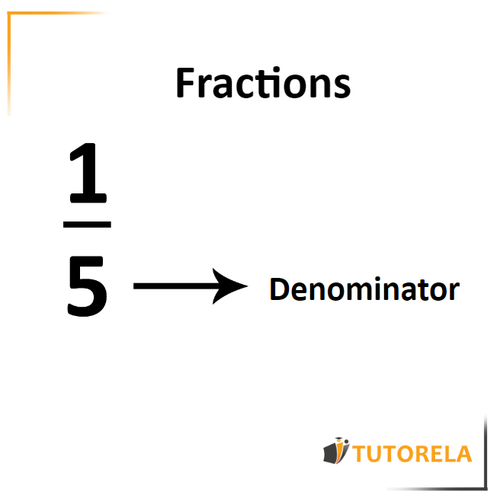
The denominator is the bottom number of a fraction and represents the whole in its entirety.
For example:


My numerator is 2 and my denominator is 9.
Which fraction am I?
In this article, you will learn everything you need to know about the denominator and its function in fractions.
The denominator is one of the components of a fraction, therefore, to better understand what the denominator is, let's first talk about fractions.
A fraction is a number that is composed of two numbers:
The upper one which is called the numerator
A fractional line that represents a division
And the lower number which we call the denominator
For example:

The fraction could represent a certain part or even the entirety of a whole.
My numerator is 3 and my denominator is 8.
Which fraction am I?
My numerator is 5 and my denominator is 8.
Which fraction am I?
My numerator is 6 and my denominator is 7.
Which am I?
The denominator represents the whole itself, that is, the totality of parts or portions there are.
For example, in the fraction
,
The denominator indicates that is the whole, in total there are parts.
Explanatory note as a gift: The in the numerator represents a certain part within the whole parts within , that is, eighths.
Let's see it illustrated:
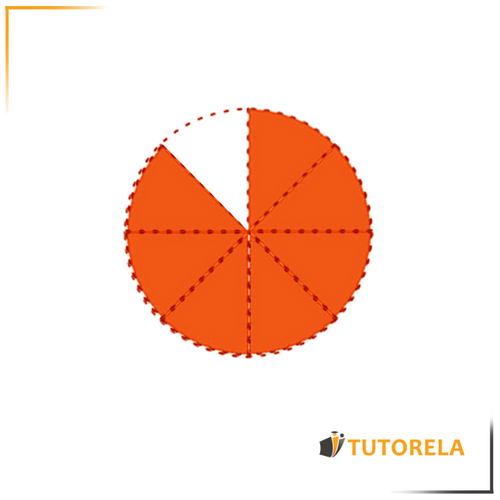
Discover the fractions whose denominator is :
Solution:
In this fraction, the denominator is –> the number located at the bottom.
In this fraction, the denominator is –> the number located at the bottom.
My numerator is 8 and my denominator is 11.
Which fraction am I?
Solve the following expression:
\( \frac{10}{5}= \)
Solve the following expression:
\( \frac{13}{13}= \)
Write fractions whose denominator is :
Solution:
In the three fractions we wrote, the denominator is . Any fraction you write that has the number as the denominator and any whole number as the numerator will be a correct answer.
My numerator is 2 and my denominator is 9.
Which fraction am I?
Let's remember that the numerator is the number at the top of the fraction, whilst the denominator is the number at the bottom of the fraction.
If we arrange the given values accordingly we should obtain the following:
My numerator is 3 and my denominator is 8.
Which fraction am I?
Let's remember that the numerator is the number at the top of the fraction , whilst the denominator is the number at the bottom of the fraction.
If we insert the given values accordingly we should obtain the following:
My numerator is 5 and my denominator is 8.
Which fraction am I?
Remember that the numerator is the number at the top of the fraction, whilst the denominator is the number at the bottom of the fraction.
If we place the given values accordingly we should obtain the following:
My numerator is 6 and my denominator is 7.
Which am I?
Remember that the numerator of the fraction is the top half, whilst the denominator of the fraction is the bottom half.
If we position them accordingly we should obtain the following:
My numerator is 8 and my denominator is 11.
Which fraction am I?
Remember that the numerator is the number at the top of the fraction, whilst the denominator is the number at the bottom of the fraction.
If we arrange the given data accordingly we should obtain the following:
Solve the following expression:
\( \frac{2}{18}= \)
Solve the following expression:
\( \frac{29}{29}= \)
Solve the following expression:
\( \frac{56}{7}= \)