A common denominator is a denominator that will be common and equal for all the fractions in the exercise. We will reach such a denominator by reducing or enlarging the fraction - an operation of multiplication or division.
We can arrive at several correct common denominators.
Common denominator
We will divide the search for the common denominator into 3 cases:
- The first case: one of the denominators appearing in the original exercise will be the common denominator.
In this case, we will notice that we only have to multiply one denominator by an integer to reach the same denominator as in the other fraction. - The second case: find a number that both denominators in the exercise can reach by multiplication.
- The third case: find the common denominator by multiplying the denominators.
Test yourself on common denominators!

Solve the following equation:
\( \frac{1}{2}+\frac{3}{8}= \)
Common denominator
A common denominator is a topic that will accompany you for a long time from now until the end of your math studies, so you should know how to find it easily.
What is a common denominator?
It is a denominator that will be common and equal in all the fractions of the exercise. We will reach such a denominator by reducing or expanding the fraction - a multiplication or division operation.
We can arrive at several correct common denominators.
We will divide the search for the common denominator into 3 cases:
The first case: one of the denominators that appears in the original exercise - will be the common denominator.
In this case, we will notice that we only have to multiply a denominator by an integer so that it reaches the same denominator as in the other fraction.
Let's look at an example
Find the common denominator in the exercise:
Solution:
We will notice that we can multiply by to reach the denominator .
and therefore the common denominator will be .
We will obtain:
Pay attention: When we multiply the fraction to reach a common denominator, we must multiply both the numerator and the denominator so as not to change the value of the fraction.
Solve the following equation:
\( \frac{2}{4}+\frac{1}{2}= \)
Solve the following equation:
\( \frac{2}{3}+\frac{1}{6}= \)
Solve the following equation:
\( \frac{2}{4}+\frac{1}{8}= \)
Now we will move on to the second case: finding a number that both denominators in the exercise can reach through multiplication.
Pay attention: sometimes, we might not reach that number and then we will move directly to the third case.
Let's look at an example
Find the common denominator in the exercise.
Solution:
If we look at the denominators, we can come to the fact that is a number whose denominators can reach it through a multiplication operation.
If we multiply by we get the result
If we multiply by we get the result
Remember that when we find a common denominator, we also perform the operation on the numerator and not just on the denominator.
We will obtain:
The common denominator is .
The third case: finding the common denominator by multiplying the denominators
Sometimes, we will not be able to reach a common denominator using the first and the second method, so we will resort to this method.
Keep in mind that multiplying denominators is always a safe way to find a common denominator and you can operate immediately (unless you want to find the least common denominator.
Let's look at this in an exercise
Find the common denominator.
Solution:
We multiply the denominator of the first fraction by the entire second fraction and the denominator of the second fraction We multiply by the entire first fraction.
Remember to perform the operation both in the numerator and the denominator.
Also, it is customary to mark the multiplication operation with a line above the fraction as follows:
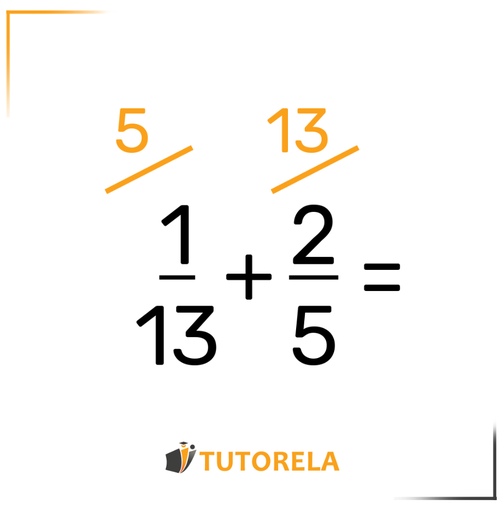
The common denominator is .
Another exercise
Find the common denominator in the exercise.
Solution:
We can solve this exercise in several ways. It is also suitable for the first case, as well as for the second and third.
Basically, we will arrive at different common denominators, all of which will have a correct answer.
Pay attention: You can use the method of multiplying the denominators in any exercise and, therefore, its use is always recommended.
But if you want to find the least common denominator that is possible to achieve (we will always go in order) first we will see if we can reach the common denominator by the first case, then the second and only then if we have not achieved it, we will move to the third.
Solution through the first case and finding the least common denominator:
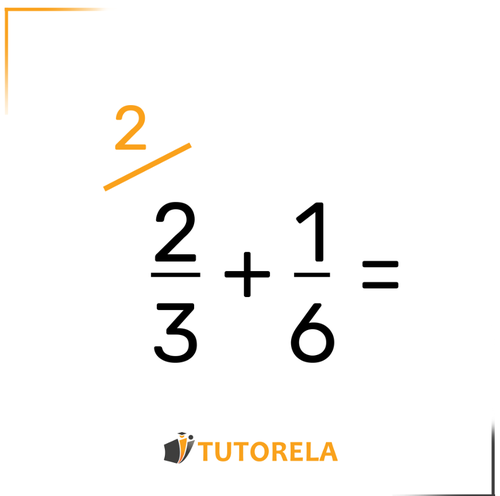
We will notice that if we multiply by we will arrive at .
We will obtain:
The common denominator is .
Solution through the second case: finding a common number through a multiplication operation
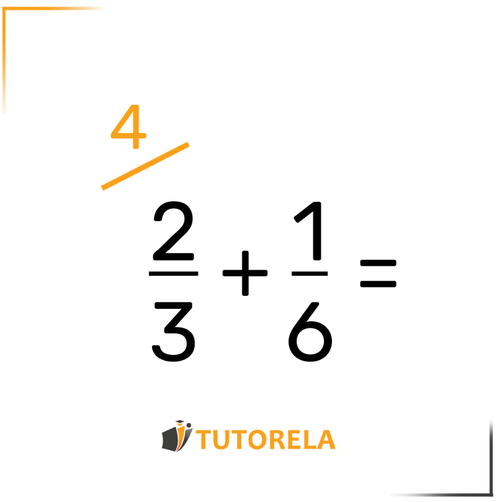
If we multiply by we will arrive at If we multiply by we will arrive at.
We obtain:
Solution through the third case: multiplying the denominators
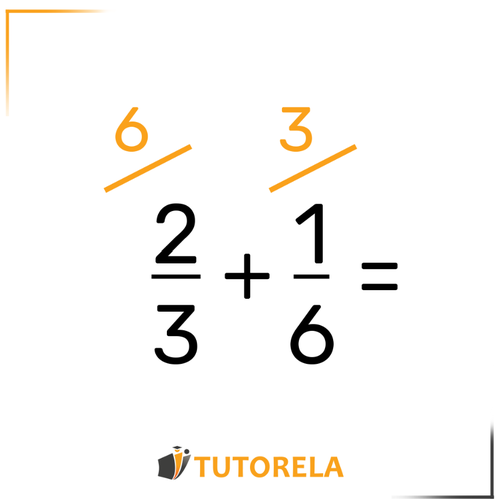
Multiply the denominator of the first fraction In the second fraction and in the denominator of the second fraction Multiply by the first fraction.
We obtain:
Examples and exercises with solutions of common denominator
Exercise #1
Solve the following equation:
Video Solution
Step-by-Step Solution
Let's first identify the lowest common denominator between 2 and 8.
In order to determine the lowest common denominator, we need to first find a number that is divisible by both 2 and 8.
In this case, the common denominator is 8.
We'll then proceed to multiply each fraction by the appropriate number in order to reach the denominator 8.
We'll multiply the first fraction by 4
We'll multiply the second fraction by 1
Finally we'll combine and obtain the following:
Answer
Exercise #2
Solve the following equation:
Video Solution
Step-by-Step Solution
Let's first identify the lowest common denominator between 4 and 2.
In order to identify the lowest common denominator, we need to find a number that is divisible by both 4 and 2.
In this case, the common denominator is 4
We will then proceed to multiply each fraction by the appropriate number in order to reach the denominator 4
We'll multiply the first fraction by 1
We'll multiply the second fraction by 2
Finally we will combine and obtain the following:
Answer
Exercise #3
Solve the following equation:
Video Solution
Step-by-Step Solution
Let's begin by identifying the lowest common denominator between 3 and 6.
In order to determine the lowest common denominator, we need to find a number that is divisible by both 3 and 6.
In this case, the common denominator is 6.
Let's proceed to multiply each fraction by the appropriate number in order to reach the denominator 6.
We'll multiply the first fraction by 2
We'll multiply the second fraction by 1
Finally we'll combine and obtain the following result:
Answer
Exercise #4
Solve the following equation:
Video Solution
Step-by-Step Solution
We must first identify the lowest common denominator between 4 and 8
In order to determine the lowest common denominator, we need to find a number that is divisible by both 4 and 8.
In this case, the common denominator is 8.
We will proceed to multiply each fraction by the appropriate number to reach the denominator 8.
We'll multiply the first fraction by 2
We'll multiply the second fraction by 1
Finally we'll combine and obtain the following:
Answer
Exercise #5
Solve the following equation:
Video Solution
Step-by-Step Solution
We must first identify the lowest common denominator between 5 and 10.
In order to determine the lowest common denominator, we need to find a number that is divisible by both 5 and 10.
In this case, the common denominator is 10.
We will proceed to multiply each fraction by the appropriate number to reach the denominator 10.
We'll multiply the first fraction by 2
We'll multiply the second fraction by 1
Finally we'll combine and obtain the following:
Answer
Solve the following equation:
\( \frac{1}{5}+\frac{6}{10}= \)
Solve the following equation:
\( \frac{1}{3}+\frac{3}{6}= \)
Solve the following equation:
\( \frac{3}{4}+\frac{1}{8}= \)
More Questions
- The Order of Basic Operations: Addition, Subtraction, and Multiplication
- Order of Operations: Exponents
- Order of Operations: Roots
- Division and Fraction Bars (Vinculum)
- The Numbers 0 and 1 in Operations
- Neutral Element (Identiy Element)
- Order of Operations with Parentheses
- Order or Hierarchy of Operations with Fractions
- Opposite numbers
- Elimination of Parentheses in Real Numbers
- Addition and Subtraction of Real Numbers
- Multiplication and Division of Real Numbers
- Multiplicative Inverse
- Integer powering
- Positive and negative numbers and zero
- Real line or Numerical line
- Fractions
- A fraction as a divisor
- How do you simplify fractions?
- Simplification and Expansion of Simple Fractions
- Hundredths and Thousandths
- Part of a quantity
- Sum of Fractions
- Subtraction of Fractions
- Multiplication of Fractions
- Division of Fractions
- Comparing Fractions
- Placing Fractions on the Number Line
- Numerator
- Denominator
- Decimal Fractions
- What is a Decimal Number?
- Reducing and Expanding Decimal Numbers
- Addition and Subtraction of Decimal Numbers
- Comparison of Decimal Numbers
- Converting Decimals to Fractions
- Remainder of a fraction
- Decimal fraction remainder
- Remainders
- Order of Operations - Exponents and Roots
- Special cases (0 and 1, reciprocals, fraction line)









