The Numbers 0 and 1 in Operations

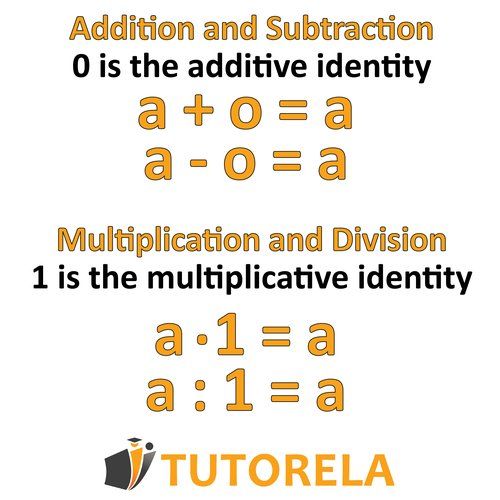
When we add zero to a number, it will remain unchanged because no value has been added to it.
$5+0=5$

The same happens when we subtract $0$ from a number: the number does not change because we are not taking anything away from it.
$\ 5-0=5$

In multiplications, the result will always be $0$.
$\ 5 \cdot 0=0$

We can summarize multiplication by $0$ as follows (whether it is multiplied by a positive or negative number):

$\ 0\cdot a=0$ and $\ a\cdot 0=0$.

Even when dividing $0$ by another number, the result will always be $0$.
$\ 0:5=0$

$\ 0:\frac{1}{2}=0$

$\ 0:1000=0$

$\ {0 \over a}=0$ and $\ 0: a=0$ (Assuming that (a) is not $0$).

For addition and subtraction operations, 1 adds or subtracts one unit to the number.

$\ 5+1=6$,$\ 5-1=4$

$\ 1+1=2$,$\ 1-1=0$

$\ 10+1=11$,$\ 10-1=9$

For multiplications, when a number is multiplied by $\ 1$ it will not change.

$\ 5 \cdot 1=5$

$\ 253 \cdot 1=253$

$\ 10.000 \cdot 1=10.000$

Something very similar happens with division: if we divide a number by one, the number remains unchanged.

$\ 5:1=5$

$\ 200:1=200$

$\ 1.000.000:1=1.000.000$

After reviewing the characteristics of 0 and 1, let's try to use them to solve combined exercises according to the order of operations.

Calculate $\left(10:2+3-6\right)\left(3^2-2×4\right)$.

Solution:

We perform the division inside the first parenthesis before the power inside the second parenthesis.

$\left(5+3-6\right)\left(9-2×4\right)$

We perform the additions and subtractions inside the first parenthesis (from left to right) and then the multiplication inside the second parenthesis.

$\left(8-6\right)\left(9-8\right)$

We perform the subtractions.

$\left(2\right)\left(1\right)$

We have obtained a multiplication of a number by one. Therefore, the result is:

$2$

If you are enjoying this article, you may also be interested in the following:

• Order of basic operations: addition, subtraction, multiplication and division.
• Order of basic operations (powers)
• Order of basic operations: roots
• Neutral element / Neutral elements

On Tutorela website, you will find a variety of useful articles on mathematics!

They are numbers that have very important characteristics when we perform operations with them. 1 is known as multiplicative neutral and zero is known as additive neutral. This is because the numbers remain the same when you multiply them by one or add zero to them.

Zero in mathematics is used to represent the null value or absence.

Zero is an integer. It is neither positive nor negative and is used to represent the null value or as an origin in various situations.

Division by zero is not defined.