Multiplicative Inverse

Two numbers are multiplicative inverses when their product results in $1$ .

For example:

${\Large {1 \over 2}}$ and $2$ are multiplicative inverses because ${\Large 2 \cdot {1 \over 2}=1}$

Formulation of the Rule for Multiplicative Inverse Numbers:

Whenever a is different from $0$ , it follows that ${\Large a\cdot{1 \over a} = 1}$

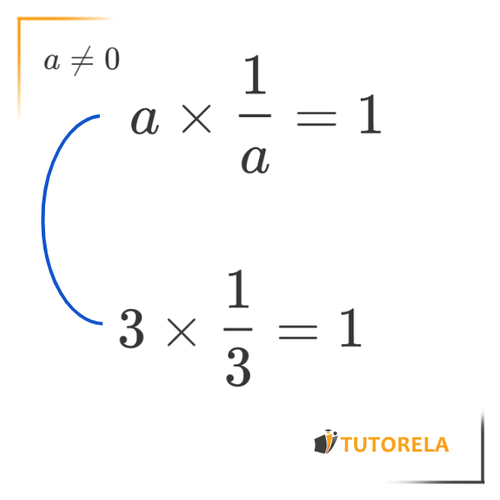
Multiplication and Division of Multiplicative Inverses

Division is equivalent to multiplication by its multiplicative inverse,

That is: ${\Large {{2 \over {1 \over 3}} = 2 \cdot 3 = 6}}$

Because $3$ is the multiplicative inverse of ${\Large {1 \over 3}}$

Generally: $\frac{a}{\frac{1}{b}}=a⋅b$

Detailed explanation

Start practice

Test yourself on special cases (0 and 1, inverse, fraction line)!

$8\times(5\times1)=$

Practice more now

Two numbers are multiplicative inverses when their multiplication results in $1$ .

For example:

${\Large {1 \over 2}}$ and $2$ are multiplicative inverses because ${\Large 2 \cdot {1 \over 2}=1}$

More examples:

The multiplicative inverse of $5$ is ${\Large {1 \over 5}}$
${\Large 5 \cdot {1 \over 5}=1}$

The multiplicative inverse of $3$ is ${\Large {1 \over 3}}$
${\Large 3 \cdot {1 \over 3}=1}$

The multiplicative inverse of ${\Large {5 \over 7}}$ is ${\Large {7 \over 5}}$
${\Large {7 \over 5} \cdot {5 \over 7}=1}$

The multiplicative inverse of ${\Large {9 \over 23}}$ is ${\Large {23 \over 9}}$
${\Large {23 \over 9} \cdot {9 \over 23}=1}$

The multiplicative inverse of $0.5$ is $2$

${\Large 2 \cdot 0.5=1}$

The multiplicative inverse of $0.25$ is $4$

${\Large 4 \cdot 0.25=1}$

Formulation of the Rule for Multiplicative Inverse Numbers:

Whenever a is different from $0$ , it happens that ${\Large a\cdot{1 \over a} = 1}$

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

$\frac{6}{3}\times1=$

Question 2

$(3\times5-15\times1)+3-2=$

Question 3

$(5\times4-10\times2)\times(3-5)=$

Multiplication and Division of Multiplicative Inverses

Division is equivalent to multiplication by the multiplicative inverse,

that is: ${\Large {{2 \over {1 \over 3}} = 2 \cdot 3 = 6}}$

This is because $3$ is the multiplicative inverse of ${\Large {1 \over 3}}$

In general: ${\Large a /{1 \over b} = a \cdot b}$

Exercises on Multiplicative Inverses

Solve the following exercises

${\Large {5+{{4 \over 7} \over 2} =}}$
${\Large {{6 \over 0.75} - 2 \cdot 3 =}}$
${\Large {{3{1 \over 2}-{{1 \over 3} \over {1 \over 6}}}=}}$
${\Large {{{{10 \over 7} \over 2 } + {2 \over {7 \over 8} }}=}}$
${\Large {{{3 \over 5} \over {9 \over 10}} + {{7 \over 9} \over {1 \over 3}}=}}$

Examples and Exercises with Solutions for Multiplicative Inverse

Exercise #1

$8\times(5\times1)=$

Video Solution

Step-by-Step Solution

According to the order of operations, we first solve the expression in parentheses:

$5\times1=5$

Now we multiply:

$8\times5=40$

Answer

Exercise #2

$(3\times5-15\times1)+3-2=$

Video Solution

Step-by-Step Solution

This simple rule is the order of operations which states that exponentiation precedes multiplication and division, which precede addition and subtraction, and that operations enclosed in parentheses precede all others,

Following the simple rule, multiplication comes before division and subtraction, therefore we calculate the values of the multiplications and then proceed with the operations of division and subtraction

$3\cdot5-15\cdot1+3-2= \\ 15-15+3-2= \\ 1$ Therefore, the correct answer is answer B.

Answer

$1$

Exercise #3

$(5\times4-10\times2)\times(3-5)=$

Video Solution

Step-by-Step Solution

This simple rule is the order of operations which states that multiplication precedes addition and subtraction, and division precedes all of them,

In the given example, a multiplication occurs between two sets of parentheses, thus we simplify the expressions within each pair of parentheses separately,

We start with simplifying the expression within the parentheses on the left, this is done in accordance with the order of operations mentioned above, meaning that multiplication comes before subtraction, we perform the multiplications in this expression first and then proceed with the subtraction operations within it, in reverse we simplify the expression within the parentheses on the right and perform the subtraction operation within them:

What remains for us is to perform the last multiplication that was deferred, it is the multiplication that occurred between the expressions within the parentheses in the original expression, we perform it while remembering that multiplying any number by 0 will result in 0:

Therefore, the correct answer is answer d.

Answer

$0$

Exercise #4

$(5+4-3)^2:(5\times2-10\times1)=$

Video Solution

Step-by-Step Solution

In the given expression, the establishment of division between two sets of parentheses, note that the parentheses on the left indicate strength, therefore, in accordance to the order of operations mentioned above, we start simplifying the expression within those parentheses, and as we proceed, we obtain the result derived from simplifying the expression within those parentheses with given strength, and in the final step, we divide the result obtained from the simplification of the expression within the parentheses on the right,

We proceed similarly with the simplification of the expression within the parentheses on the left, where we perform the operations of multiplication and division, in strength, in contrast, we simplify the expression within the parentheses on the right, which, according to the order of operations mentioned above, means multiplication precedes division, hence we first perform the operations of multiplication within those parentheses and then proceed with the operation of division:

$(5+4-3)^2:(5\cdot2-10\cdot1)= \\ (-2)^2:(10-10)= \\ 4:0\\$ We conclude that the sequence of operations within the expression that is within the parentheses on the left yields a smooth result, this result we leave within the parentheses, these we raised in the next step in strength, this means we remember that every number (positive or negative) in dual strength gives a positive result,

As we proceed, note that in the last expression we received from establishing division by the number 0, this operation is known as an undefined mathematical operation (and this is the simple reason why a number should never be divided by 0 parts) therefore, the given expression yields a value that is not defined, commonly denoted as "undefined group" and use the symbol :

$\{\empty\}$ In summary:

$4:0=\\ \{\empty\}$ Therefore, the correct answer is answer A.

Answer

No solution

Exercise #5

Solve the following exercise:

$12+3\cdot0=$

Step-by-Step Solution

According to the order of operations, we first multiply and then add:

$12+(3\cdot0)=$

$3\times0=0$

$12+0=12$

Answer

$12$

Do you know what the answer is?

Question 1

$(5+4-3)^2:(5\times2-10\times1)=$

Question 2

Solve the following exercise:

$12+3\cdot0=$

Question 3

Solve the following exercise:

$$ 2+0:3= $$

Start practice