Constant Function

Constant Function - Examples, Exercises and Solutions
🏆Practice increasing and decreasing intervals of a function
5 Questions
Functions
Increasing and Decreasing Intervals of a Function
Practice
Add a new subject

We will say that a function is constant when, as the value of the independent variable X X increases, the dependent variable Y Y remains the same.

Let's assume we have two elements X X , which we will call X1 X1 and X2 X2 , where the following is true: X1<X2 X1<X2 , that is, X2 X2 is located to the right of X1 X1 .

  • When X1 X1 is placed in the domain, the value Y1 Y1 is obtained.
  • When X2 X2 is placed in the domain, the value Y2Y2 is obtained.


The function is constant when: X2>X1 X2>X1 and also \(Y2=Y1).

The function can be constant in intervals or throughout its domain.

Constant Function

Constant Function

Start practice

Test yourself on increasing and decreasing intervals of a function!

Determine in which domain the function is negative?

–0.5–0.5–0.50.50.50.51111.51.51.5222000

Practice more now

If you are interested in this article, you might also be interested in the following articles:

Graphical representation of a function

Algebraic representation of a function

Notation of a function

Domain of a function

Indefinite integral

Assignment of numerical value in a function

Variation of a function

Increasing function

Decreasing function

Functions for seventh grade

Intervals of increase and decrease of a function

In the blog of Tutorela you will find a variety of articles with interesting explanations about mathematics

Constant Function Exercises

Exercise 1

Assignment

Find the decreasing and increasing area of the function

f(x)=5x2−25 f(x)=5x^2-25

Solution

In the first step, let's consider that a=5 a=5

Therefore a>0 a>0 and the parabola is at the minimum

In the second step, we find x x of the vertex

according to the data we know:

a=5,b=0,c=−25 a=5,b=0,c=-25

We replace the data in the formula:

x=−b2⋅a x=\frac{-b}{2\cdot a}

x=−02⋅5 x=\frac{-0}{2\cdot5}

x=−010 x=\frac{-0}{10}

x=0 x=0

Answer

x<0 x<0 Decreasing

0<x 0<x Increasing

Join Over 30,000 Students Excelling in Math!
Endless Practice, Expert Guidance - Elevate Your Math Skills Today
Test your knowledge
Question 1

Does the function in the graph decrease throughout?

YYYXXX

Question 2

In what domain does the function increase?

–20–20–20–10–10–10101010202020–10–10–10101010000

Question 3

In what domain does the function increase?

000

Exercise 2

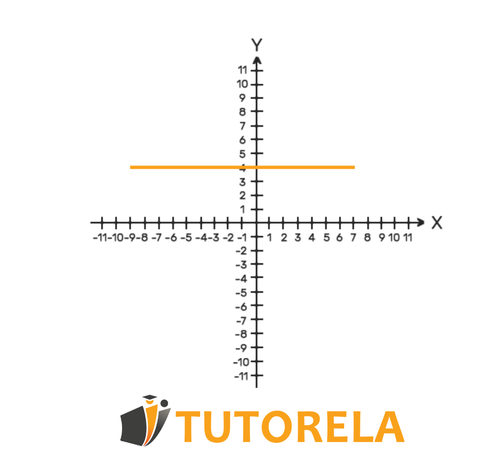
Assignment

Given the linear function of the graph

What is the domain of negativity of the function?

the function is always above the x-axis

Solution

Keep in mind that the function is always above the axis: x x

That is, the function is always positive and has no negative domain. Therefore, no x x

Answer

The function is always positive

Exercise 3

Assignment

Find the increasing area of the function

f(x)=6x2−12 f(x)=6x^2-12

Solution

In the first step, let's consider that a=6 a=6

Therefore a>0 a>0 and the parabola is a minimum

In the second step, we find x x of the vertex

according to the data we know that:

a=6,b=0,c=−12 a=6,b=0,c=-12

We replace the data in the formula

x=−b2⋅a x=\frac{-b}{2\cdot a}

x=−02⋅6 x=\frac{-0}{2\cdot6}

x=012 x=\frac{0}{12}

x=0 x=0

Therefore

0<x 0<x Increasing

x<0 x<0 Decreasing

Answer

0<x 0<x

Do you know what the answer is?
Question 1

In what domain is the function increasing?

–5–5–5555101010151515–5–5–5555000

Question 2

Is the function in the graph below decreasing?

yx

Question 3

Is the function in the graph decreasing?

yx

Exercise 4

Assignment

To find the increasing and decreasing area of the function, you need to find the intersection point of the vertex

Answer

True

Exercise 5

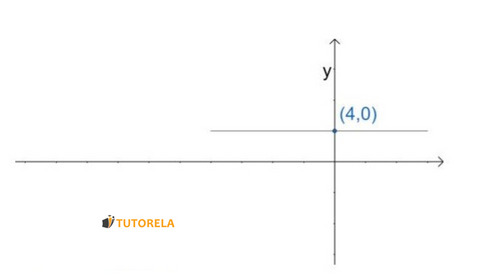
Assignment

Given the function in the diagram, what is its domain of positivity?

what is its domain of positivity

Solution

Note that the entire function is always above the axis: x x

Therefore, it will always be positive. Its area of positivity will be for all x x

Answer

For all x x

Check your understanding
Question 1

Is the function in the graph decreasing? yx

Question 2

Is the function shown in the graph below decreasing?

yx

Question 3

Is the function shown in the graph below decreasing?

yx

Examples with solutions for Constant Function

Exercise #1

Determine in which domain the function is negative?

–0.5–0.5–0.50.50.50.51111.51.51.5222000

Video Solution

Step-by-Step Solution

Remember that a function is increasing if both X values and Y values are increasing simultaneously.

A function is decreasing if X values are increasing while Y values are decreasing simultaneously.

In the graph, we can observe that in the domain x>1 x > 1 the function is decreasing, meaning the Y values are decreasing.

Answer

x>1 x > 1

Exercise #2

Does the function in the graph decrease throughout?

YYYXXX

Step-by-Step Solution

To solve this problem, we'll begin by examining the graph of the function provided:

  • Step 1: Observe the graph from left to right along the x-axis.
  • Step 2: Look for any intervals where the function value (y-coordinate) does not decrease as the x-value increases.
  • Step 3: Pay special attention to segments where the graph might look horizontal or rising.

Upon inspecting the graph, we find:

- There are sections where the function's y-values appear to remain constant or potentially rise as the x-values increase. Specifically, even if the function decreases in major portions, any interval where it doesn't means the function cannot be classified as decreasing throughout.

Thus, the function does not strictly decrease on the entire interval shown. Therefore, the solution to the problem is No.

Answer

No

Exercise #3

In what domain does the function increase?

–20–20–20–10–10–10101010202020–10–10–10101010000

Video Solution

Step-by-Step Solution

Let's remember that the function increases if the x x values and y y values increase simultaneously.

On the other hand, the function decreases if the x x values increase while the y y values decrease simultaneously.

In the given graph, we can see that the function increases in the domain where x>0 x > 0 ; in other words, where the y y values are increasing.

Answer

x>0 x > 0

Exercise #4

In what domain does the function increase?

000

Video Solution

Step-by-Step Solution

Let's remember that the function increases if the X values and Y values increase simultaneously.

On the other hand, the function decreases if the X values increase and the Y values decrease simultaneously.

In the given graph, we notice that the function increases in the domain where x<0 x < 0 , meaning the Y values are increasing.

Answer

x<0 x<0

Exercise #5

In what domain is the function increasing?

–5–5–5555101010151515–5–5–5555000

Video Solution

Step-by-Step Solution

Let's first remember that a function is increasing if both the X and Y values are increasing simultaneously.

Conversely, a function is decreasing if the X values are increasing while the Y values are decreasing simultaneously.

In the graph shown, we can see that the function is increasing in every domain and therefore the function is increasing for all values of X.

Answer

All values of x x

Start practice

More Questions

Related Subjects
👉  Click here  to practice