Linear Function

A linear function, as it is called, is an algebraic expression that represents the graph of a straight line.

When we talk about functions, it's important to highlight that the graphs of functions are represented in an axis system where there is a horizontal axis $X$ and a vertical axis $Y$ .

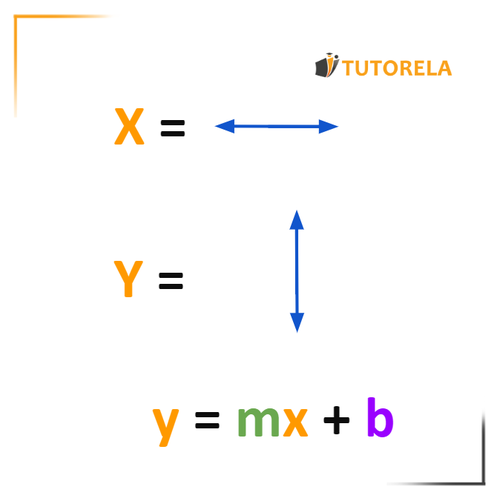
Linear functions can be expressed by the expressions $y = mx$ or $y = mx + b$ , where m represents the slope of the line while $b$ (when it exists) represents the y-intercept.

To plot a linear function, all we need are $2$ points. If the linear function is given, you can substitute a value for $X$ and obtain the corresponding $Y$ value.

Detailed explanation

Start practice

Test yourself on linear functions!

For the function in front of you, the slope is?

Practice more now

A linear function is from the family of functions $Y=mx+b$ and represents a straight line.

$m$

We mark the slope of the function: positive or negative.

$m>0$ : upward sloping line

$m<0$ : downward sloping line

$m=0$ : a line parallel to the $X$ axis.

$b$

Marks the point where the function intersects the $Y$ axis.

Important points:

For every value of $X$ , the function will return a value of $Y$ .

The linear function is a straight line that is either ascending, descending, or parallel to the $X$ axis, but never parallel to the $Y$ axis.

Let's observe the line from left to right.

Different Representations of Linear Functions

$Y=mx+b$ : standard representation

$Y=mx$ : (when $b=0$ , the line cuts the $Y$ axis at the origin, when $Y=0$ )

$Y=b$ (when $m=0$ , the slope of the line is $0$ and therefore parallel to the $X$ axis)

Pay attention:

Sometimes, you'll find an equation that is not arranged and looks like this $mx-y=b$

This is also a linear function. Simply isolate $Y$ and see how it comes to the standard representation.

Sometimes, $X$ will be divided by some number $C$ :

$Y=\frac{mx}{c}+b$

This equation also represents a linear function.

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

For the function in front of you, the slope is?

Question 2

For the function in front of you, the slope is?

Question 3

For the function in front of you, the slope is?

When is a function not a linear function?

When $X$ is raised to a power: $Y=X^2$
When there is a square root for: $Y=\sqrt{X}$
When there is no $Y: X=b$

Graphical Representation of a Function that Depicts a Straight-Line Relationship

The linear function will appear as a straight line that is ascending, descending, or parallel to the $X$ axis but never parallel to the $Y$ axis.

For each value of $X$ , we will obtain only one value of $Y$ and vice versa.

We observe the line from left to right to see whether it ascends or descends.

Want to know more? Check out this article.

The concept of slope in the function $y=mx$

$M$ represents the slope of the function for us and determines whether the straight line goes up, goes down, or is parallel to the $X$ axis.

The linear function $y=mx+b$

The linear function represents a straight line where $M$ represents the slope and $b$ represents the y-intercept of the line with the $Y$ axis.

Do you know what the answer is?

Question 1

For the function in front of you, the slope is?

Question 2

For the function in front of you, the slope is?

Question 3

For the function in front of you, the slope is?

Finding the Equation of a Straight Line

We can find the equation of a straight line in 5 ways:

Using slope and a point
With the help of 2 points the line passes through.
Using parallel lines.
With the help of perpendicular lines.
With the help of a graph.

Want to learn more? Check out this article.

Positivity and Negativity of a Function

The function is positive when it is above the $X$ axis when $Y>0$

Find the values of $X$ for which the function takes on positive Y values.

The function is negative when it is below the $X$ axis as $Y>0$

Find the values of $X$ for which the function takes on negative $Y$ values.

Want to know more? Check out this article.

Check your understanding

Question 1

For the function in front of you, the slope is?

Question 2

For the function in front of you, the slope is?

Question 3

For the function in front of you, the slope is?

Representation of Phenomena Using Linear Functions

A linear function describes the relationship between $X$ and $Y$ .

Therefore, we can represent different phenomena with the help of a linear function.

We will understand what graph represents each situation and draw the correct conclusions.

Want to know more? Click on this article.

Inequality

We can solve inequalities between linear functions in two ways:

Using the given equations and with the help of graphical representations.

Want to know more? Check out this article.

Do you think you will be able to solve it?

Question 1

Look at the linear function represented in the diagram.

When is the function positive?

Question 2

Look at the function shown in the figure.

When is the function positive?

Question 3

What is the solution to the following inequality?

$$ 10x-4\leq-3x-8 $$

Let's illustrate this with an example.

Given the function: $y = 2x + 1$

We are asked to graph it on the coordinate system.

As we have discussed, to do this we need two points, which we will place in the function's expression. Choose any two points we like, it doesn't matter.

Now we will plot the two points on the coordinate system and connect them. This is actually a graph of the function for $y=2x+1$ .

Examples and Exercises with Solutions for Linear Functions

Exercise #1

For the function in front of you, the slope is?

Video Solution

Step-by-Step Solution

For this problem, we need to determine the nature of the slope for a given straight line on a graph.

Based on the graph provided, the red line starts at a higher point on the left (Y-axis) and moves downward toward a lower point on the right (X-axis). This indicates that as one moves from left to right across the graph, the function decreases in value. Consequently, this is typical of a line that has a negative slope.

The slope of a line is typically defined as the "rise over the run," or the ratio of the change in the vertical direction to the change in the horizontal direction. Here, as we proceed from left to right, the line goes "downwards" (negative rise), establishing a negative slope.

Thus, we can conclude that the slope of the line is negative.

Therefore, the solution to the problem is Negative slope.

Answer

Negative slope

Exercise #2

For the function in front of you, the slope is?

Video Solution

Step-by-Step Solution

To determine the slope of the line segment shown in the graph, follow these steps:

Identify the line segment on the graph; it's shown as a red line from one point to another.
Examine the direction the line segment travels from the leftmost point to the rightmost point.
Visually analyze whether the line segment is rising or falling as it moves from left to right.

Here is the detailed analysis:
- The red line segment starts lower on the left side and ends higher on the right side.
- This suggests that as we move from left to right, the line is rising.
- In terms of slope, a line that rises as it moves from left to right has a positive slope.

Therefore, the slope of the line segment is positive.

Thus, the correct answer is Positive slope.

Answer

Positive slope

Exercise #3

For the function in front of you, the slope is?

Video Solution

Step-by-Step Solution

To determine the slope of the line shown on the graph, we perform a visual analysis:

We examine the orientation of the line from left to right.
The red line starts at a higher point on the left and descends to a lower point on the right.
This indicates a downward movement, which corresponds to a negative slope.

Therefore, by observing the direction of the line, we conclude that the slope of the function is negative. This positional evaluation confirms that the correct answer is negative slope.

Answer

Negative slope

Exercise #4

For the function in front of you, the slope is?

Video Solution

Step-by-Step Solution

To determine the slope of the line, we'll examine the direction of the line segment on the graph:

The line depicted moves from the top left, passing through a point with higher $y$ -coordinate values, to the bottom right, ending at a point with lower $y$ -coordinate values.
This movement indicates that as $x$ increases (the direction to the right along the $x$ -axis), the $y$ -coordinate decreases.
When the $y$ -value reduces as the $x$ -value grows, the slope $m$ is negative.

Since the line descends from left to right, the slope of the line is negative.

Therefore, the slope of the function is a negative slope.

Answer

Negative slope

Exercise #5

For the function in front of you, the slope is?

Video Solution

Step-by-Step Solution

To solve this problem, follow these steps:

Step 1: Observe the given graph and the plotted line.
Step 2: Determine the direction of the line as it moves from left to right across the graph.
Step 3: Understand that a line moving downwards from left to right represents a negative slope.

Now, let's work through these steps:

Step 1: The graph shows a straight line that starts higher on the left side and descends towards the right side.

Step 2: As the line moves from left to right, it descends. This is a key indicator of the slope type.

Step 3: A line that moves downward from the left side to the right side of the graph (decreasing in height as it proceeds to the right) is characteristic of a negative slope. Conversely, a positive slope would show a line ascending as it moves rightward.

Therefore, the solution to the problem is the line has a negative slope.

Answer

Negative slope

Test your knowledge

Question 1

Given the linear function:

$$ y=x-4 $$

What is the rate of change of the function?

Question 2

Given the linear function:

$$ y=1-4x $$

What is the rate of change of the function?

Question 3

For the function in front of you, the slope is?

Start practice

Linear Function

Test yourself on linear functions!

Linear Function

$m$

$b$

Different Representations of Linear Functions

When is a function not a linear function?