The Order of Operations

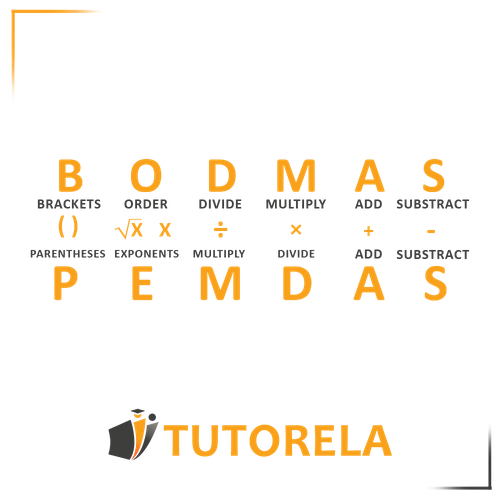
It is important to perform the operations in the right order to arrive at the correct result.

1. The first operations we have to solve are those in parentheses. Once solved, we can continue. Some exercises include parentheses inside other parentheses. In such cases, we must first solve the internal parentheses before solving the external ones.
2. The next operations that we must solve are powers and roots, also referred to as 'exponents'. When these are inside parentheses, we solve them first in order to solve the parentheses.
3. The next operations we must perform are multiplication and division. When there is more than one in the exercise, they must be solved in order from left to right.
4. The last operations to perform are addition and subtraction. These must also be solved from left to right in instances when there is more than one in the same exercise.

Before talking about more advanced mathematical operations, it is important to know the order in which the elementary mathematical operations (addition, subtraction, multiplication and division) are solved.

In each exercise we will solve these operations in the following order:

1. Multiplication and division
2. Addition and subtraction

For example, in the exercise:

• $4+5\times7$

First, we solve the multiplication $\left(5\times7=35\right)$ before adding the $4$. The result is $39$.

When we have only one type of operation (only multiplication and division, or only addition and subtraction), we solve the exercise from left to right.

Let's look at the following example:

• $4-3+7-5$

In this exercise, we have only one type of operation (addition and subtraction). Therefore, we solve it from left to right:

• $4-3=1$
• $1+7=8$
• $8-5=3$

Whenever there are parentheses, the first thing to do is to perform the operations contained within them.

The order of operations in cases where there are parentheses and basic operations is as follows:

1. Parentheses
2. Powers and roots
3. Multiplication and division (from left to right)
4. Addition and subtraction (from left to right)

For example, in the exercise:

• $4\times5+(7-3)$

First, we perform the operation inside the parentheses: $\left(7-3\right)=4$.

Then, we solve the operation outside of the parentheses: $4\times5=20$.

Finally, we add both figures together: $20+4=24$.

Use parentheses '( )' instead of brackets '[ ]'.

When an exercise includes powers or roots, these operations will be solved before the multiplication and division. Therefore, the order to follow is:

1. Parentheses
2. Powers and Roots
3. Multiplication and division
4. Addition and subtraction

For example:

• $4+3\times3^2\times(7-5)=$

First, we solve the operation inside parentheses:

• $7-5=2$
• $4+3\times3^2\times2=$

Next, we simplify the power:

• $3^2=9$
• $4+3\times9\times2=$

Then, we perform the multiplications (from left to right):

• $3X9=27$
• $4+27\times2=$

We continue with the second multiplication:

• $27\times2=54$
• $4+54=$

Finally, we perform the addition that will give us the answer:

• $4+54=58$

1. Parentheses
2. Powers and roots
3. Multiplication and division
4. Addition and subtraction

1. Parentheses
2. Powers and roots
3. Multiplication and division
4. Addition and subtraction

What is the order when solving an equation?

It depends on the type of equation. However, it is important not to confuse the term 'equation' with the term 'expression'. This article deals with arithmetic expressions.

To solve an equation, for example a first-degree equation, both sides must be simplified using the order of operations. If there are unknown variables on both sides, they must be put together on a single side.

What is solved first in an expression?

1. Operations inside parentheses
2. Powers and roots
3. Multiplication and division
4. Addition and subtraction

Which is performed first, addition or subtraction?

They are performed in order from left to right.

What is a combined operation?

An arithmetic expression which include several operations.

What is the order of combined operations without parentheses?

1. Powers and roots
2. Multiplication and division
3. Addition and subtraction

What is the order of operations?

It is the order in which operations are carried out in an exercise.

Which operation has the highest priority?

The powers and roots. Parentheses are not really operations, but rather symbols that help us group operations together.

Which is solved first when there are no parentheses, addition or multiplication?

Multiplication.

What is the solution to this exercise?

$5-2\times\frac{1}{2}+1=$

Solution:

In the first step of the exercise, you must calculate the multiplication.

$2\times\frac{1}{2}=\frac{2}{1}\times\frac{1}{2}=\frac{2}{2}=1$

From here, you can continue with the remaining addition and subtraction operations.

$5-1+1=5$

Answer: $5$

What is the solution to this exercise?

$12:(4\ -\frac{9}{3})=$

Solution:

We begin with the parentheses and the division operation inside it.

$9:3=3$

Then we perform the subtraction operation.

$4-3=1$

Finally, we perform the division operation that is outside of the parentheses.

$12:1=12$

Answer:

$12$

What is the solution to this exercise?

$(X+4)(3+X)$

Given that:

$X=4$

Solution:

We start by substituting the value of $X$.

$(4+4)(3+4)$

Then, we perform the calculations in parentheses.

$(8)(7)$

Once we have solved the parentheses, we simply multiply the resulting figures together.

$7\times 8=56$

Answer: $56$

Determine the missing number in the equation:

$?\colon(-6)+\lbrack-2(94-73)\rbrack=-153$

Solution:

Calculate according to the order of operations to work out the value of the unknown variable.

$?=X$

$X:-6+(-2(94-73))=-153$

$X:-6+(-2(21))=-153$

$X:-6-42=-153$

$X:-6=-153+42$

$X=-111\times 6$ / $\times -6$

$X=666$

Answer:

$X=666$

In a flower field there are a number of flowers.

One bush contains $5$ flowers, and there are $13$ such bushes.

Another area has $9$ plants with $2$ flowers each. The flower pickers picked $30$ flowers from the bushes and $10$ from the plants.

Task:

How many flowers are left in the field?

Solution:

We will turn the question into an expression with which we are familiar:

$\left(5\times 13-30\right)+\left(9\times 2-10\right)$

First, we will solve everything in the parentheses, starting with multiplication and division from left to right.

$\left(65-30\right)+\left(18-10\right)$

Then, we perform the addition and subtraction operations found in parentheses.

$35+8$

Finally, we perform the operations outside the parentheses.

$35+8=43$

Answer:

$43$

If you enjoyed this article, you may also be interested in the following:

• Order of basic operations: addition, subtraction, multiplication and division.
• Order of basic operations (powers)
• Order of basic operations: roots
• The 0 and the 1 in the order of operations
• Neutral element / Neutral elements

On the Tutorela blog, you can find a variety of helpful articles on the subject of mathematics!

• $2+3\times4=$
• $3-5\times2+3=$
• $6\times7+3\times4=$
• $2-3+5\times6:2=$
• $3+4-12:3:2\times6=$

• $3+5\times(2+3)=$
• $\left(3-5\right)\times(2+3)=$
• $3+4-12:3:(2\times2)=$
• $\left(2-3\right)+5\times(6:2)=$

• $2+2\times4^2=$
• $2+(2\times4)^2=$
• $3^2-12:3:(2X2)=$
• $\left(2-3\right)+5\times(6:2)^2+(2^2-3)=$

• $2+3\times4=14$
• $3-5\times2+3=-4$
• $6\times7+3\times4=54$
• $2-3+5\times6:2=14$
• $3+4-12:3:2\times6=-5$

• $3+5\times(2+3)=28$
• $\left(3-5\right)\times(2+3)=-10$
• $3+4-12:3:(2\times2)=6$
• $\left(2-3\right)+5\times(6:2)=14$

• $2+2\times4^2=34$
• $2+(2\times4)^2=66$
• $3^2-12:3:(2^{\times}2)=8$
• $\left(2-3\right)+5\times(6:2)^2+(2^2-3)=45$

• $10\times2:4=$
• $80:4:2:5=$
• $36-4:2=$
• $24:2-4-3:3=$
• $5\times5\times2-12:4=$
• $100:2-10:2+3\times4-6-5\times2=$