Solving algebraic equations is made easier by understanding some basic rules and properties. A few examples of properties that we will learn to use in the seventh grade are: the distributive, associative and commutative properties. These properties get learned and relearned throughout our time in school, each time adding new layers to or understanding. Today we will focus on the distributive property. We will go into depth on what it is and how to use it, and we will briefly get to know the associative and commutative properties as well.
The Distributive Property for Seventh Graders
What is the distributive property?
The distributive property is a method to simplify multiplication and division exercises. Essentially, it breaks down expressions into smaller, easier to manage terms.
Let's see some examples:
If we look at the following examples, we can see that we have broken down the larger number into several smaller numbers that are more manageable. The value is the same as before, but now we can distribute a complex operation into several easy operations.
The distributive property can be described as:
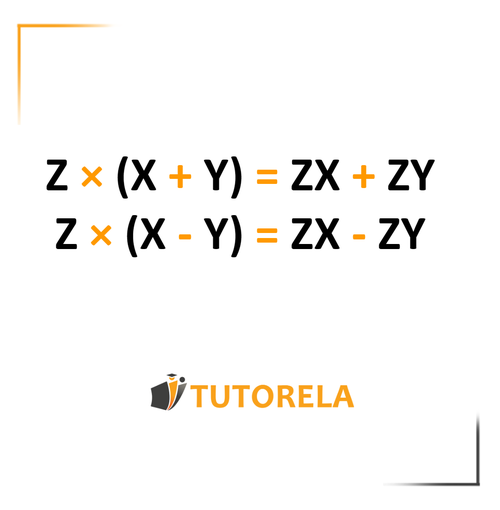
Test yourself on the distributive property for 7th grade!
\( 140-70= \)
The distributive property
Sometimes, an expression will require us to perform both addition and subtraction within our parentheses. Not to worry! The distributive property can simplify these expressions too.
Let's see some examples:
How would you go about using the distributive property in an equation with two sets of parentheses?
First, we multiply the first term of the first parenthesis by the first and second terms of the second parenthesis..
Next, we multiply the second term of the first parenthesis and multiply it by the first and second terms of the second parenthesis.
Remember to place the addition and subtraction signs in the correct places.
Another way to describe the distributive property:
The distributive property in elementary school
When first learning about the distributive property, we practice only with known whole numbers (without variables or fractions) in order to understand the idea of breaking down a larger number into smaller numbers. In this first stage, we use the distributive property mainly to simplify calculation, especially with large numbers.
For example:
By this point, most students have already mastered long addition and subtraction, but they might have less experience multiplying large numbers. The distributive property helps them to solve these problems by reducing into simpler multiplications equations.
\( 143-43= \)
\( 94+72= \)
Solve the exercise:
84:4=
The distributive property in middle school
In middle school, the distributive property gets more interesting. Now, we will start to use not just whole numbers, but variables and exponents too!
For example:
Other properties
As we mentioned earlier, there are other rules and properties out there that help us to simplify algebraic expressions. In this section we will briefly look at two of them: the associative property and the commutative property.
Solve the following exercise
?=24:12
Solve the following exercise
?=93:3
\( 133+30= \)
The associative property
The associative property allows us to group several terms of an equation together without changing the final results, by moving the parentheses. However, we can only use this property to solve addition or multiplication exercises.
For example:
To learn more about the associative property, you can read the following: The Associative Property.
The commutative property
The commutative property allows us to change the order of the terms in an equation without changing the outcome of the equation. Like the associative property, the commutative property can only be used for addition and multiplication.
Let us look at some examples:
To learn more about the commutative property, you can read the following: The Commutative Property.
\( 63-36= \)
Solve the following problem:
\( 72:6= \)
\( 30\times39= \)
Example exercises for seventh graders
Exercise 1
Task:
Using the distributive property, solve the following:
Solutions:
Exercise 2
Task:
Three hundred fifty-one students from a high school were divided into nine equal groups.
How many students are in each group?
Solve the problem using the distributive property.
Solution:
We begin by expressing the problem numerically:
Answer:
There are 39 students in each group.
\( 480\times3= \)
\( 35\times4= \)
\( \)\( 3\times56= \)
Exercise 3
Task:
Dani bought 15 boxes. In each box there were 9 pieces of candy.
How many pieces of candy did Dani buy in total?
Use the distributive property to solve the problem.
Solution:
We begin by expressing the problem numerically:
Answer:
Dani bought 135 pieces of candy.
Exercise 4
Task:
Isabel has packed 246 notebooks into 6 equal packages.
How many notebooks has Isabel put in each package?
Use the distributive property to solve the problem.
Solution:
We begin by expressing the problem numerically:
Answer:
Isabel has put 41 notebooks in each packet.
\( 6\times29= \)
Solve the exercise:
=74:4
\( 140-70= \)
Exercise 5
Task:
A mother had 894 euros. She divided the money equally among her three children.
How much money did each child receive?
Use the distributive property to solve the problem.
Solution:
We begin by expressing the problem numerically:
Answer:
Each child received 298 euros.
Exercise 6
Task:
Solve the following:
Solution:
We simplify the number 93 into 4 smaller numers to make it easier for us to divide it by 3.
For example
Then we will add the results and we will get:
Answer:
\( 143-43= \)
\( 94+72= \)
Solve the exercise:
84:4=
Exercise 7
Task:
Solve the following:
Solution:
We simplify the number 90 into 3 smaller numbers:
(50,20,20)
Then we divide each of them by 5 and add the three results.
Which gives us:
Answer:
Exercise 8
Task:
Solve the following:
Solution:
We simplify the number 72 into two smaller numbers and then divide each of them by 18.
Answer:
Solve the following exercise
?=24:12
Solve the following exercise
?=93:3
\( 133+30= \)
Exercise 9
Task:
Solve the following:
Solution:
Answer:
Exercise 10
Task:
Solve the following:
Solution:
Answer:
\( 63-36= \)
Solve the following problem:
\( 72:6= \)
\( 30\times39= \)
Exercise 11
Task:
Solve the following:
Solution:
,
,
Answer:
More practice
\( 480\times3= \)
\( 35\times4= \)
\( \)\( 3\times56= \)
FAQs about the distributive property
What is the distributive property?
The distributive property is a method used to simplify expressions into smaller, more manageable pieces.
How is the distributive property used?
In an equation, we use the distributive property to break down a large number into two or more smaller numbers (using addition and subtraction), and then by distributing the multiplication.
Example
Can we use the distributive property in division?
Of course we can! In an expression with division, we break down the numerator into smaller numbers (using addition and subtraction), and then the division is distributed.
Example
If you found this article helpful, you may also be interested in the following:
- Equivalent expressions / Equivalent algebraic expressions.
- The Associative Property
- The distributive property in the case of divisions
- The distributive property in the case of multiplication
- The distributive property: extension
- The commutative property of addition
- The commutative property of multiplication
- The associative property
- Associative property of addition
- Associative property of multiplication
- Distributive property
For a wide range of mathematics articles, visit Tutorela website.
\( 6\times29= \)
Solve the exercise:
=74:4
\( 140-70= \)
Examples with solutions for The Distributive Property for 7th Grade
Exercise #1
Solve the following exercise
?=24:12
Video Solution
Step-by-Step Solution
Apply the distributive property of division and proceed to split the number 24 into a sum of 12 and 12, which ultimately renders the division operation easier and allows us to solve the exercise without a calculator.
Note - it's best to choose to split the number based on knowledge of multiples. In this case of the number 12 because we need to divide by 12.
Reminder - The distributive property of division actually allows us to split the larger term in a division problem into a sum or difference of smaller numbers, which makes the division operation easier and allows us to solve the exercise without a calculator
We will use the formula of the distributive property
Therefore the answer is section a - 2.
Answer
2
Exercise #2
Video Solution
Step-by-Step Solution
In order to simplify the calculation , we first break down 94 and 72 into smaller and preferably round numbers.
We obtain the following exercise:
Using the associative property, we then rearrange the exercise to be more functional.
We solve the exercise in the following way, first the round numbers and then the small numbers.
Which results in the following exercise:
Answer
166
Exercise #3
Video Solution
Step-by-Step Solution
In order to simplify the resolution process, we begin by using the distributive property for 140:
We then rearrange the exercise using the substitution property into a more practical form:
Lastly we solve the exercise from left to right:
Answer
70
Exercise #4
Video Solution
Step-by-Step Solution
In order to solve the question, we first use the distributive property for 133:
We then use the distributive property for 33:
We rearrange the exercise into a more practical form:
We solve the middle exercise:
Which results in the final exercise as seen below:
Answer
163
Exercise #5
Solve the exercise:
84:4=
Video Solution
Step-by-Step Solution
There are several ways to solve the following exercise,
We will present two of them.
In both ways, we begin by decomposing the number 84 into smaller units; 80 and 4.
Subsequently we are left with only the 80.
Continuing on with the first method, we will then further decompose 80 into smaller units;
We know that:
And therefore, we are able to reduce the exercise as follows:
Eventually we are left with
which is equal to 20
In the second method, we decompose 80 into the following smaller units:
We know that:
And therefore:
which is also equal to 20
Now, let's remember the 1 from the first step and add it in to our above answer:
Thus we are left with the following solution:
Answer
21
More Questions
The Distributive Property for 7th Grade
- Recurrence Relations
- Sequences
- Algebraic Method
- Factorization: Common factor extraction
- The Extended Distributive Property
- Advanced Arithmetic Operations
- The commutative property
- The Commutative Property of Addition
- The Commutative Property of Multiplication
- The Associative Property
- The Associative Property of Addition
- The Associative Property of Multiplication
- Subtracting Whole Numbers with Addition in Parentheses
- Division of Whole Numbers Within Parentheses Involving Division
- Subtracting Whole Numbers with Subtraction in Parentheses
- Division of Whole Numbers with Multiplication in Parentheses
- The commutative properties of addition and multiplication, and the distributive property









