The Associative Property of Multiplication

Let's see an example:

$X\times 5\times 3=X\times 15$

We associate the second and third factors, multiply them, then multiply the product by the first factor.
We will get an expression equivalent to the first expression, since the associative property does not change the result.

Let's put a number in place of X to verify:
$X=2$
$2\times5\times3=2\times15$

$30=30$
As we can see, after applying the associative property and multiplying the last two terms (the second and third factors), and then multiplying the product by the first term, the result is the same.

Let's take a look at the following examples:

$4\times 5\times 2$

It will be easier for us to solve the exercise if we first solve $5\times2$ and only then multiply the result by 4.
The associative property of multiplication allows us to do just that. We will get:

$4\times 5\times 2=4\times 10=40$

Let's look at another example:

$9\times 25\times 4$

It will be easier for us to solve the exercise if we first solve $25\times 4$ and only then multiply the result by 9.
The associative property of multiplication allows us to do just that. Giving us:

$9\times 25\times 4=9\times 100=900$

Practice this property over and over until it becomes second nature. It will come in hand down the road for many of the different types of mathematical exercises you will encounter.

Task:

$7\times5\times2=$

Solution:

$7\times5\times2=7\times10=70$

Answer:

$70$

Task:

$3\times5\times5=$

Solution:

$3\times5\times5=3\times25=75$

Answer:

$75$

Task:

$35\times6\times2=$

Solution:

$35\times6\times2=$

$(30+5)\times6\times2=$

$=180+30\times2$

$=210\times2$

$=420$

Answer:

$420$

Task:

$(8-3-1)\times4\times3=$

Solution:

$(8-3-1)\times4\times3=\text{?}$

$(5-1)\times4\times3=\text{?}$

$(5-1)\times4\times3=4\times4\times3$

$=16\times3=48$

Answer:

$48$

Task:

$(7-4-3)(15-6-2)+(3\times5\times2)=?$

Solution:

$(7-4-3)(15-6-2)+(3\times5\times2)$

$(3-3)(9-2)+(3\times10)$

$0\times7+30=0+30=30$

Answer:

$30$

Task:

$4.1\times1.6\times3.2+4.7=\text{?}$

Solution:

$4.1\times1.6\times3.2+4.7=\text{?}$

$=4\frac{1}{10}\times1\frac{6}{10}\times3\frac{2}{10}=4\frac{7}{10}$

$\frac{41}{10}\times\frac{16}{10}\times\frac{32}{10}+\frac{47}{10}=\frac{656}{100}\times\frac{32}{10}+\frac{47}{10}$

$\frac{41\times16}{10\times10}=\frac{656}{100}$

$=\frac{656\times32}{100\times10}+\frac{47}{10} =$

$=\frac{20992}{1000}+\frac{4700}{1000}$

$=\frac{25692}{1000}=25.692$

Answer:

$25.692$

Task:

$\frac{4}{7}\times\frac{3}{2}\times\frac{7}{4}=\text{?}$

Solution:

We start by associating the left side of the expression:

$\frac{4}{7}\times\frac{3}{2}=\frac{4\times3}{7\times2}=\frac{12}{14}=\frac{6}{7}$

We continue with the whole expression:

$\frac{4}{7}\times\frac{3}{2}\times\frac{7}{4}=\frac{6}{7}\times\frac{7}{4}=\frac{6}{4}=\frac{3}{2}=1.5$

Answer:

$\frac{3}{2}$

What is the associative property in multiplication?

The associative property of multiplication tells us that in a multiplication expression with three or more factors, we can multiply factors out of order without changing the result.

For example, one way to solve the following

$6\times3\times5=$

is to associate the first two factors first

$18\times5=90$

Or we can associate the second two factors first and the result is multiplied by the first factor.

$6\times3\times5=$

$6\times15=90$

As we can see, in both cases we will get the same answer.

How is the associative property used with addition and multiplication?

In addition

The associative property in addition is represented as follows: $a+\left(b+c\right)=\left(a+b\right)+c=\left(a+c\right)+b$

This means that no matter how we order the addends, the result is the same.

Let's assign values to $a=3$, $b=8$ and $c=15$

Then, applying the associative property, we will have:

$3+\left (8+15\right)=3+23=26$

$\left (3+8\right)+15=11+15=26$

$\left (3+15\right)+8=18+8=26$

We get the same answer in all three cases.

In multiplication

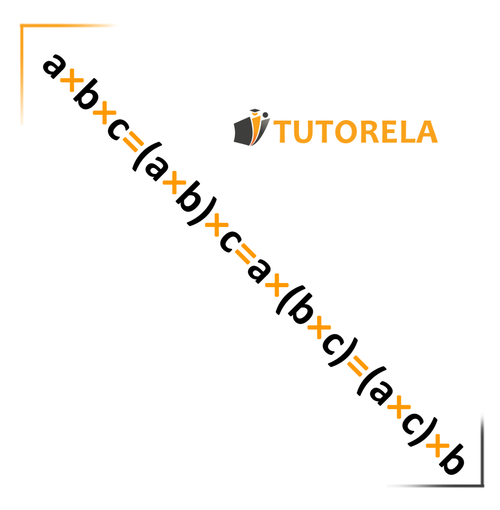
The associative property in multiplication can be represented as follows: $a\times\left(b\times c\right)=\left(a\times b\right)\times c=\left(a\times c\right)\times b$

This means that no matter which numbers are multiplied first the result is the same.

Let's assign values to $a=7$, $b=3$ and $c=2$

Then, applying the associative property, we will get:

$\left (7 \times3\right)\times2=21 \times2=42$

$7\times \left (3\times2\right)=7 \times6=42$

$\left (7\times 2\right) \times3=14 \times3=42$

We can see that all three expressions gave us the same answer, even though the factors were in a different order.

What are the associative, commutative and distributive properties of multiplication?

Associative: We can regroup the factors of an expression without changing the result.$a\times\left(b\times c\right)=\left(a\times b\right)\times c=\left(a\times c\right)\times b$

Commutative: We can reorder the factors in an expression without changing the result.

$a\times b=b\times a$

Distributive: We can break down a multiplication operation into simpler expressions. $a\times\left(b+c\right)=a\times b+a\times c$

If you found this article helpful, you might also be interested in the following:

Commutative property of addition

The commutative property of multiplication

The associative property

Associative property of addition

The distributive property for students of 1st grade of ESO

Distributive property

Abbreviated multiplication formulas

For a wide range of mathematics articles visit Tutorela's website