Frequently Asked Questions
Everything you need to know about The Order of Operations
What is the correct order of operations in math?
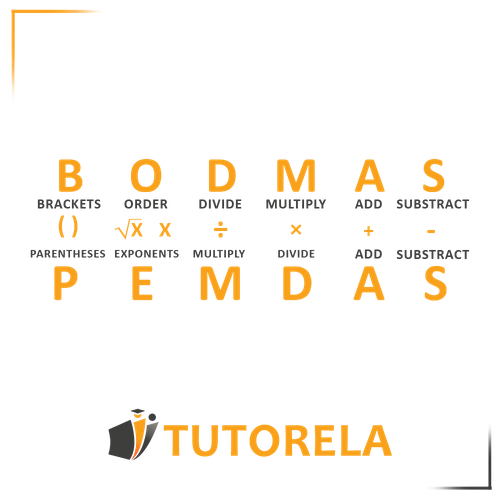
+ The order of operations follows PEMDAS/BODMAS: 1) Parentheses/Brackets, 2) Exponents/Orders (powers and roots), 3) Multiplication and Division (left to right), 4) Addition and Subtraction (left to right). This ensures everyone gets the same answer when solving mathematical expressions.
Why do we solve 4 + 5 × 7 as 39 instead of 63?
+ According to order of operations rules, multiplication comes before addition. So we first calculate 5 × 7 = 35, then add 4 to get 39. If we added first (4 + 5 = 9), then multiplied by 7, we'd incorrectly get 63.
How do you solve expressions with multiple parentheses?
+ Work from the innermost parentheses outward. For nested parentheses like ((2 + 3) × 4), solve the inner parentheses first: (2 + 3) = 5, giving you (5 × 4) = 20. Always complete all operations inside parentheses before moving to the next step.
Do multiplication and division have the same priority?
+ Yes, multiplication and division have equal priority in the order of operations. When both appear in an expression, solve them from left to right. For example, in 12 ÷ 4 × 3, first divide: 12 ÷ 4 = 3, then multiply: 3 × 3 = 9.
What's the difference between PEMDAS and BODMAS?
+ PEMDAS and BODMAS are the same rule with different names: PEMDAS (Parentheses, Exponents, Multiplication, Division, Addition, Subtraction) is used in the US, while BODMAS (Brackets, Orders, Division, Multiplication, Addition, Subtraction) is used in the UK. Both follow identical mathematical principles.
How do you handle exponents in order of operations?
+ Exponents (powers and roots) are calculated after parentheses but before multiplication and division. In 4 + 3 × 3² × (7-5), solve in this order: 1) Parentheses: (7-5) = 2, 2) Exponent: 3² = 9, 3) Multiplication: 3 × 9 × 2 = 54, 4) Addition: 4 + 54 = 58.
What are common order of operations mistakes students make?
+ The most common mistakes include: solving operations left to right without following PEMDAS, forgetting to solve parentheses first, treating addition and subtraction as having different priorities (they're equal), and not recognizing that multiplication by juxtaposition (like 2(3+4)) means multiplication.
Can you use order of operations in word problems?
+ Absolutely! Word problems often require order of operations. For example: 'A field has 13 bushes with 5 flowers each, minus 30 picked flowers, plus 9 plants with 2 flowers each, minus 10 picked flowers.' This becomes (5×13-30)+(9×2-10) = (65-30)+(18-10) = 35+8 = 43 flowers remaining.