Verbal Problem Solving With a System of Linear Equations

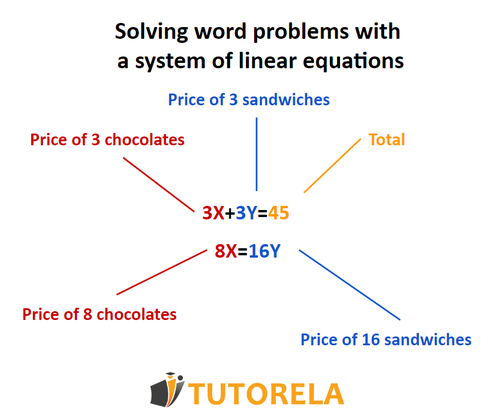
To solve verbal problems with systems of linear equations we will construct the system of equations based on the text of the problem.

We will start by pointing out what we are asked to solve for in the unknowns $X$ and $Y$ .
Based on the conditions met by $X$ and $Y$ in the verbal problem we will write down the relevant equations with the corresponding numbers and unknowns.
After drawing the equations appropriate to the problem, we can solve them in the algebraic way: through the substitution method or the equalization method, in this way we will solve for the two unknowns.

Example of Solving Word Problems with a System of Linear Equations

The price of $3$ chocolates and $3$ snacks is $45$ $.
The price of $8$ chocolates equals the price of $16$ sandwiches.
What is the price of the chocolate and the sandwich?
We will indicate what we are asked to find with the unknowns $X$ and $Y$ :
$X=$ Price of the chocolate
$Y=$ Price of the snack

We will make the equations according to the conditions stated in the problem:
In fact, we will translate the text to equation and we will do it on the basis of what is written.

A - Verbal problem solving with a system of linear equations

We will solve the system of equations in the way that is most comfortable for us. Here we will use the substitution method and isolate the $X$ .
$x=2y$
We will substitute it into the first equation and find the : We will place it in the equation that is most convenient for us to find the : And from here we will give the verbal answer like this: The price of the chocolate is $ and the price of the snack is $. $Y$
$3 \times 2y+3y=45$
$6y+3y=45$
$9y=45$
$y=5$
$X$
$x=2 \times 5$
$X=10$
$10$ $5$

If you are interested in this article you may also be interested in the following articles

In Tutorela's blog you will find a wide variety of mathematical articles.

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today