Find the value of x and and band the substitution method. $$ (I)-x+3and=12 $$ $$ (II)4x+2and=10 $$

$$ x=\frac{3}{7},y=\frac{29}{7} $$

$$ x=\frac{4}{9},y=\frac{5}{9} $$

Substitution method for two linear equations with two unknowns

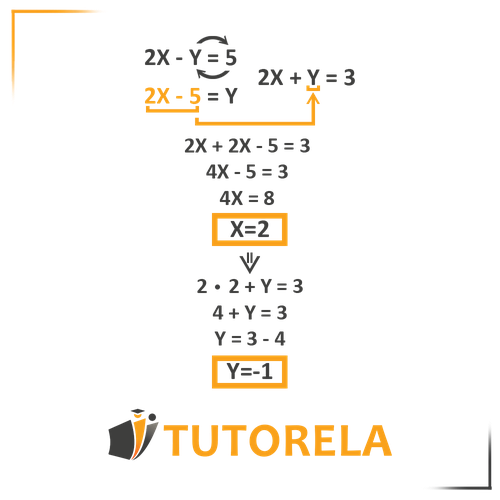
To solve with the substitution method a system of two linear equations with two unknowns we will have to substitute one of the unknowns in some equation and thus obtain an equation with only one unknown.

How do we do it?

Choose the equation in which you can easily isolate one of the unknowns (isolate it in such a way that it cannot express itself).
Put the unknown that you have isolated in the second equation of the system: you will have an equation with one unknown and you will discover the value of the first one.
Go back to the system of equations and place the value of the unknown you found in one of the equations or in the equation obtained to find the second unknown.

Detailed explanation

Start practice

Test yourself on algebraic solution!

Solve the following system of equations:

$\begin{cases} x-y=5 \\ 2x-3y=8 \end{cases}$

Practice more now

Example of the substitution method

$X+2Y=20$
$4X-3Y=14$

Let's isolate the $X$ from the first equation since it is the easiest:
$X=20-2Y$
Let's substitute the $X$ in the second equation:
$4\times (20-2y)-3y=14$
We obtained an equation with one unknown, therefore, we will solve it easily:
$80-8y-3y=14$
$80-11y=14$
$-11y=-66$
$y=6$

Let's place the value obtained in the simpler equation (the equation we obtained after isolating the $X$ ) and find the second unknown:
$x=20-2\times 6$
$x=8$
The solution is: $x=8$
$y=6$