In this article, we will learn how to solve fractional equations. When we encounter an equation that has a number in the denominator, we will want to get rid of that number and achieve having no denominator at all.
We will do this by multiplying both sides of the equation by the smallest possible common denominator.
In this article, we will see several examples of exercises with numbers in the denominator, and we will gradually move up in levels of difficulty.
The first exercises will be relatively simple, having a single fraction with a number in the denominator. In the following, we will see exercises with two or three fractions.
When we encounter an equation that has a number in the denominator, we will want to get rid of that number and achieve having no denominator. We will do this by multiplying both sides of the equation by the smallest possible common denominator.
Examples with solutions of equations with fractions
Example 1
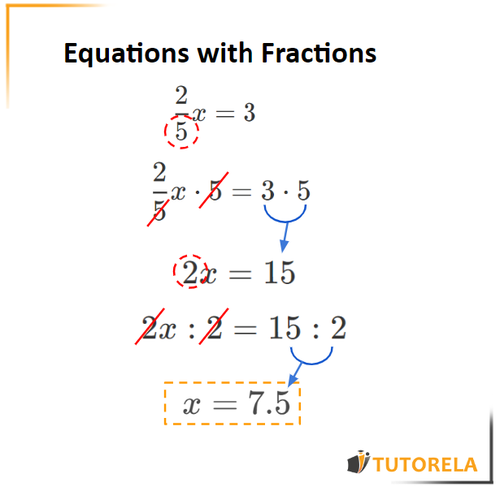
52X=3
In this case, we have a 5 in the denominator. We want to get rid of it. So, we will multiply both sides of the equation by the denominator, that is by five.
It is customary to express it this way:
52X=3 /×5
We will multiply each side separately and obtain:
52X×5=3×5
That is,
2x=15 /:2
x=7.5
Join Over 30,000 Students Excelling in Math!
Endless Practice, Expert Guidance - Elevate Your Math Skills Today
Example 2
31X=2X−4
We will multiply both sides of the equation by the denominator, that is, we will multiply by 3 and we will obtain
31X=2X−4 /×3
31X×3=2X×3−4×3
x=6x−12
12=5x
X=512
Example 3: Equation with Two Denominators
32X+1=23X−2+4
In this exercise, we have two fractions, that is, two different denominators. Also here our goal is to get rid of the denominators. To achieve this, let's multiply both sides of the equation by the smallest possible common denominator. In this case, the common denominator is 6. We will obtain:
At this point, a multiplication sign appears in the graph, as seen in the Word.
32X+1=23X−2+4 /×6
32X+1×6=23X−2×6+4×6
2(2x+1)=3(3x−2)+4×6
4x+2=9x−6+24
−16=5x
X=−516
Example 4
35X−4X+1=2X+1
We would like to get rid of the denominator of the two fractions, therefore, we will multiply again by the least common denominator. The smallest common denominator is 12 in this case.
35X−4X+1=2X+1 /×12
35X×12−(4X+1)×12=2X×12+1×12
20x−3×(x+1)=24x+12
20x−3x−3=24x+12
−15=7x
X=−715
Example 5
2X+3X=41
Also in this situation, we want to get rid of the denominator. We have to multiply by the smallest possible common denominator. We will see that multiplying all the denominators together will give us 24, this is a common denominator that could work for us, but it is not the smallest and, therefore, it is not convenient to use it for multiplication.
Let's remember that to find the smallest common denominator we must multiply all the prime factors of the numbers that appear in the denominator. In our case: 24 is composed of 2 and 2. 3 is a prime number. The 2 is also a prime number, also included within the 4.
Therefore, we have to multiply:
2×2×3=12
That is, 12 is the smallest possible common denominator. So let's multiply both sides by 12 and we will obtain:
2X+3X=41 /×12
2X×12+3X×12=41×12
6x−4x=3
2x=3
X=23