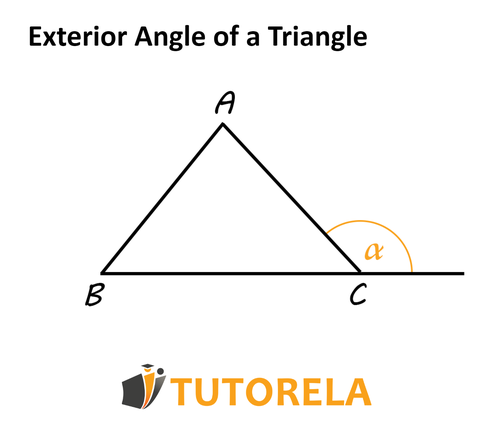
Exterior angles of a triangle
The exterior angle of a triangle is the one that is found between the original side and the extension of the side.
Key Properties:
- The exterior angle is equal to the sum of the two interior angles of the triangle that are not adjacent to it.
- Each exterior angle is supplementary to its adjacent interior angle, meaning their sum is .
- The sum of all exterior angles of a triangle is always , no matter the shape of the triangle.
It is defined as follows: