Vertical Arithmetic Operations

Sometimes we encounter relatively complex addition exercises like the following:
$5658+3641=$

It would be difficult for us to perform this addition in our head.
That's exactly why we'll use the vertical addition method, which should make these addition exercises significantly easier.
How do we write a vertical addition problem?
When we encounter an exercise like this for example:
$5658+3641=$

We will need to write it so that one number is under the other in this way:
ones digit under ones digit, tens digit under tens digit, hundreds digit under hundreds digit, and thousands digit under thousands digit.
For example, the exercise
$5658+3641=$
will be written like this

Pay attention - we will write the + sign on the left side to show that this is an addition problem.
Now what? Let's solve it!
We always start from the ones digit and add them together - meaning $8+1$
Remember to write the result exactly under the ones digit as seen below.

Now let's continue to the next digit - the tens digit.
Let's add them together $5+4$ and write the result right below:

Let's proceed to the hundreds digit and add them together.
Note: $6+6=12$
Since $12$ is a two-digit number, we won't write $12$ instead
we'll only write the ones digit $24$.
We'll write the $1$ above the thousands digit as seen below:

Pay attention -
We wrote $1$ above the thousands digit and now we'll add the thousands digits together with the one we wrote above.
That is:
$1+5+3=9$

We're done!
The result of the exercise is $9299$

Now let's summarize all the rules and steps for vertical addition:

1. Write the numbers vertically one below the other, aligning ones under ones, tens under tens, hundreds under hundreds, and thousands under thousands.
2. Don't forget to mark $+$ on the left side and draw a line separating the exercise from the results row.
3. Start adding the ones digit of the first number with the ones digit of the second number and so on.
4. At each step, check - did we get a two-digit number?
   If yes, write only its ones digit in the results row and write the tens digit above the next column to remember to add it.
5. Only when there are no more digits to add, we can write the two-digit number that we obtained (if we obtained one) as is in the results row.

Click here to learn more about vertical addition!

Sometimes you'll encounter relatively complex subtraction exercises that look like this: $985-577=$
It would be beneficial to write them vertically in order to obtain the correct solution faster than trying to solve them mentally.

How do we solve vertical subtraction?
First rule - write the problem in the correct order!
Ones digit under ones digit, tens digit under tens digit, hundreds digit under hundreds digit, and thousands digit under thousands digit.
Pay attention! It is important that the first number in the exercise is the first and top number.
For example: $88-68=$
We write it as follows:

We will begin by writing the (minus) – sign allowing us to identify it as a subtraction exercise.
Draw a line underneath to separate the exercise from the results line.
We'll start by subtracting the ones digit to obtain the following:

$8-8=0$

Let's continue to subtract the tens digit as follows:

$8-6=2$

We're done! The result is $20$.
Now let's learn the next rule using an example:
The Second Rule - when the upper digit is smaller than the lower digit - we borrow $1$ from the next digit.
Here's a more advanced exercise!
$63-39=$

Solution:

Given that we cannot subtract $3$ minus $9$ we must borrow a digit from the next number!
That means:
$3$ will become $13$ since we'll add one to it and $6$ will become $5$.

We will write it in the following way:

Now we can solve the problem:
$13-9=4$
$5-3=2$
We obtain the following:

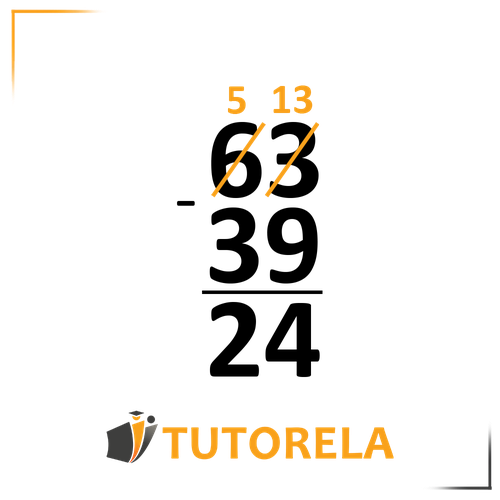
The result is $24$!

What do we do if we need to subtract a number from the digit $0$? For example, in the exercise
$50-19=$
Here too we'll need to borrow $1$ from the digit $5$ and we'll obtain an exercise like this:

Now we can solve the problem:
$10-9=1$
$4-1=3$
We obtain the following:

We're done! The result is $31$.
Now let's see what happens when we are unable to borrow from the next digit because it's also $0$:
For example in this exercise:
$700-285=$

The third rule -$0$ that cannot be borrowed from becomes $9$ until we reach a digit that is not $0$ from which we can borrow $1$.
Note! If there is a third $0$ immediately after, it becomes $8$, if there is a fourth $0$ immediately after, it becomes $7$ and so on..

We will learn the rule through an example:

We want to borrow $1$ for the first $0$ so it will become $10$.
The second $0$ will be $9$ because we are unable to borrow from it
and the digit $7$ will become $6$ since we already borrowed one from it.
We obtain the following:

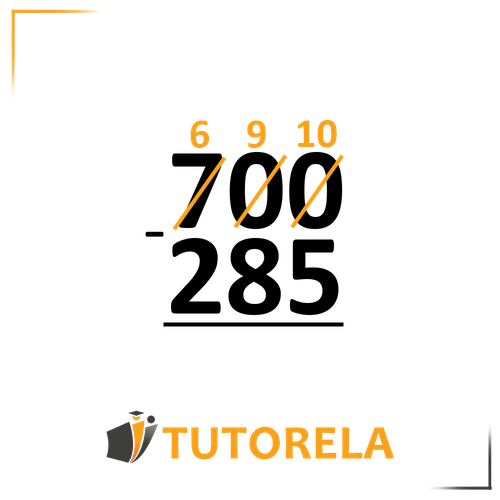
Now we are able to solve the exercise:
$10-5=5$
$9-8=1$
$6-2=4$
Let's write the solution:

We're done! The result is $415$
Click here to learn more about vertical subtraction!

What is vertical multiplication?
If until now we were used to horizontal multiplication that looks like this: $23\cdot4=$
Vertical multiplication is the same exercise just in vertical form and looks like this:

Important rules for solving:

1. Write the exercise correctly, ones under ones, tens under tens, and so on - the number with more digits should be above the number with fewer digits.
2. If the product is greater than 9, we'll keep the tens digit in the upper left and remember to add it to the next product.
3. Before moving to the next product, erase the saved numbers to avoid confusion.
4. Each time we move to a new digit, we'll add a 0 below the answer, in order that each row of answers will start one place to the left of the previous one.

And now, let's solve an exercise together, step by step and apply all the rules to the problem at hand.
Ready?

Here is the exercise $236\cdot25=$

Solve using vertical multiplication.

Solution:

Let's write the exercise correctly - the number with the most digits goes on top and below it the number with less digits. We'll make sure to write it in the correct form as per the first rule $1$.

We obtain the following:

Now we will start multiplying by the bottom digit $5$.

$5\cdot6=30$
Write $0$ in the answer row and $3$ above the $3$ in the exercise.
We obtain the following:

Multiply $5\cdot3=15$ not forgetting to add the $3$ we kept above. We should obtain $18$
Write the $8$ in the answer row and the $2$ above the $2$.
As seen below:

Multiply $5$ by the hundreds digit $2$ not forgetting to add the $1$ that we kept.
As seen below:
$5\cdot2=10$
$10+1=11$
We obtain the following:

Now we'll move to the tens digit $2$ and perform exactly the same sequence of operations. Not forgetting to add $0$ in the answer rows and erase the numbers we crossed out above. Then we'll add the answer rows as follows:

The result of the exercise is $5,900$.

Click here to learn more about vertical multiplication!

What is long division?
If until now we were used to division exercises that look like this: $12:6=$
Long division exercises are the same division exercises with a slightly different appearance -

How do we write a long division problem?
Draw a division bracket.
Write the number being divided inside the division bracket and the number we're dividing by outside the bracket on the right.

How do we solve the problem?
Each time we divide one digit. We'll start with the leftmost digit, write the division result (only whole numbers) above the division sign and move on to find the remainder by multiplying the division result by the number we're dividing by.
Write the product under the digit being divided, subtract and determine the remainder.
We'll move on to divide the next digit by bringing it down.
Once again divide in the same way and determine the remainder.
If there are no more digits to bring down, we've finished the exercise.
If we have a remainder at the end, we'll write it in parentheses next to the result above the division sign.

Let's solve an exercise together, step by step and apply all of the rules we have learned.
Ready?

Here is the exercise $732:3=$

Solve using long division.

Solution:
Let's write it in the correct form-

Begin by dividing the leftmost digit -$7$.
Write the result of the division above the r, only the whole numbers.
We obtain the following:
$7:3=2$
with a remainder.
We write $2$ above the r above the divided digit $7$.
Now let's find the remainder - multiply the result $2$ by the number we're dividing by $3$ and subtract accordingly.

The remainder is $1$.
Now, let's bring down the next digit.
We obtain a completely new number – $13$.
Divide $13$ by $3$.
$13:3=4$
and a remainder.
We'll write the quotient above the r, only the whole numbers.

Let's proceed to find the remainder - multiply the result $4$ by the number we're dividing by $3$ and subtract accordingly.
As seen below:

Bring down the next digit.
We obtain a completely new number – $12$.
We divide $12$ by $3$ and get $4$.
With no remainder.
The result of the exercise – $244$.

Click here to learn more about long division!