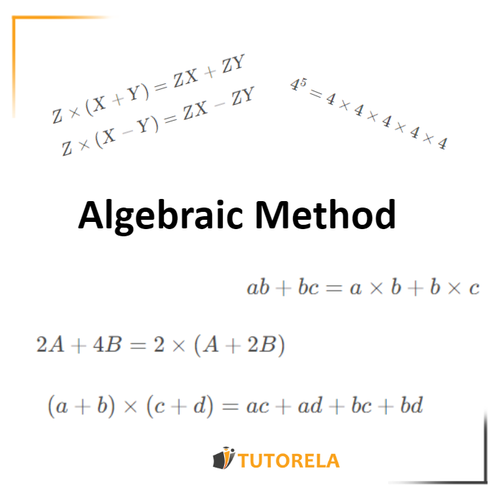
Algebraic Method Practice Problems with Solutions

Understanding Algebraic Technique

Complete explanation with examples

Algebraic Method

Algebraic Method is a general term for various tools and techniques that will help us solve more complex exercises in the future. It is mostly concern about using algebraic operations to isolate variables and solve equations. This approach is fundamental for solving equations in various mathematical contexts.

Distributive Property

This property helps us to clear parentheses and assists us with more complex calculations. Let's remember how it works. Generally, we will write it like this:

$Z\times(X+Y)=ZX+ZY$

$Z\times(X-Y)=ZX-ZY$

Extended Distributive Property

The extended distributive property is very similar to the distributive property, but it allows us to solve exercises with expressions in parentheses that are multiplied by other expressions in parentheses.
It looks like this:

$(a+b)\times(c+d)=ac+ad+bc+bd$

Factoring

The factoring method is very important. It will help us move from an expression with several terms to one that includes only one by taking out the common factor from within the parentheses.
For example:
$2A + 4B$

This expression consists of two terms. We can factor it by reducin by the greatest common factor. In this case, it's the $2$ .
We will write it as follows:

$2A+4B=2\times(A+2B)$

In this article, we’ll explain each of these topics in detail, But each of these topics will be explained even more in detail in their respective articles.

Detailed explanation

Practice Algebraic Technique

Test your knowledge with 57 quizzes

Solve the following problem:

$$ (x-8)(x+y)= $$

Examples with solutions for Algebraic Technique

Step-by-step solutions included

Exercise #1

Resolve -

$(x-3)(x-6)=$

Step-by-Step Solution

To solve this problem, we will expand the expression $(x-3)(x-6)$ using the distributive property, which involves the following steps:

Step 1: Multiply the first terms of each binomial
$(x)(x) = x^2$
Step 2: Multiply the outer terms of the binomials
$(x)(-6) = -6x$
Step 3: Multiply the inner terms of the binomials
$(-3)(x) = -3x$
Step 4: Multiply the last terms of each binomial
$(-3)(-6) = 18$
Step 5: Combine all the products
$x^2 - 6x - 3x + 18$
Step 6: Combine like terms
$-6x - 3x = -9x$ , so we have
$x^2 - 9x + 18$

Therefore, the expanded form of $(x-3)(x-6)$ is $\boxed{x^2 - 9x + 18}$ .

Therefore, the solution to the problem is $x^2 - 9x + 18$ . This corresponds to choice 1.

Answer:

$x^2-9x+18$

Video Solution

Exercise #2

Solve the exercise:

$(2y-3)(y-4)=$

Step-by-Step Solution

To solve the algebraic expression $(2y-3)(y-4)$ , we will apply the distributive property, also known as the FOIL method for binomials. This involves multiplying each term in the first binomial by each term in the second binomial.

Step 1: Multiply the first terms: $2y \times y = 2y^2$ .
Step 2: Multiply the outer terms: $2y \times -4 = -8y$ .
Step 3: Multiply the inner terms: $-3 \times y = -3y$ .
Step 4: Multiply the last terms: $-3 \times -4 = 12$ .

Next, we combine all these results: $2y^2 - 8y - 3y + 12$ .

Then, we combine the like terms $-8y$ and $-3y$ to get $-11y$ .

Therefore, the expanded expression is $2y^2 - 11y + 12$ .

This matches choice (3): $2y^2 - 11y + 12$ .

Thus, the solution to the problem is $2y^2 - 11y + 12$ .

Answer:

$2y^2-11y+12$

Video Solution

Exercise #3

Solve the exercise:

$(3x-1)(x+2)=$

Step-by-Step Solution

To solve this problem, we'll apply the distributive property to expand the expression $(3x-1)(x+2)$ . Below are the steps:

Step 1: Distribute each term in the first binomial to each term in the second binomial:

$3x(x) + 3x(2) + (-1)(x) + (-1)(2)$

Step 2: Calculate each term:

$3x^2 + 6x - x - 2$

Step 3: Combine like terms:

$3x^2 + (6x - x) - 2 = 3x^2 + 5x - 2$

Thus, the expanded expression is $3x^2 + 5x - 2$ .

The correct answer choice is $3x^2 + 5x - 2$ , corresponding to choice id="4".

Answer:

$3x^2+5x-2$

Video Solution

Exercise #4

Solve the exercise:

$(5x-2)(3+x)=$

Step-by-Step Solution

To solve the problem $(5x-2)(3+x)$ , we will use the distributive property, specifically the FOIL (First, Outer, Inner, Last) method, to expand the expression:

Step 1: Multiply the First terms: $5x \times 3 = 15x$ .
Step 2: Multiply the Outer terms: $5x \times x = 5x^2$ .
Step 3: Multiply the Inner terms: $-2 \times 3 = -6$ .
Step 4: Multiply the Last terms: $-2 \times x = -2x$ .

Now combine all these products together:

$5x^2 + 15x - 2x - 6$

Combine the like terms $15x$ and $-2x$ :

$5x^2 + (15x - 2x) - 6 = 5x^2 + 13x - 6$

Thus, the expanded form of the expression is $5x^2 + 13x - 6$ .

Answer:

$5x^2+13x-6$

Video Solution

Exercise #5

$(3+20)\times(12+4)=$

Step-by-Step Solution

Simplify this expression paying attention to the order of arithmetic operations. Exponentiation precedes multiplication whilst division precedes addition and subtraction. Parentheses precede all of the above.

Therefore, let's first start by simplifying the expressions within the parentheses. Then we can proceed to perform the multiplication between them:

$(3+20)\cdot(12+4)=\\ 23\cdot16=\\ 368$

Therefore, the correct answer is option A.

Answer:

368

Video Solution

Frequently Asked Questions

Everything you need to know about Algebraic Technique

What is the distributive property in algebra?

+

The distributive property states that Z × (X + Y) = ZX + ZY. This means you multiply the term outside the parentheses by each term inside the parentheses. It's essential for clearing parentheses and simplifying algebraic expressions.

How do you factor algebraic expressions?

+

To factor an expression, find the greatest common factor (GCF) of all terms. For example, 2A + 4B = 2(A + 2B) because 2 is the GCF. Follow these steps: 1. Identify the GCF of all terms 2. Divide each term by the GCF 3. Write the GCF outside parentheses with remaining terms inside

What's the difference between (-4)² and -4²?

+

(-4)² = (-4) × (-4) = 16 because the negative sign is inside parentheses and gets squared. However, -4² = -(4 × 4) = -16 because you calculate the exponent first, then apply the negative sign.

How does the extended distributive property work?

+

The extended distributive property multiplies two binomials: (a+b) × (c+d) = ac + ad + bc + bd. Each term in the first parentheses multiplies each term in the second parentheses, creating four products that you then combine.

When should I use factoring vs distributive property?

+

Use the distributive property to expand expressions (remove parentheses). Use factoring to simplify expressions by pulling out common factors (create parentheses). They're opposite processes - choose based on whether you want to expand or simplify.

What are common mistakes with exponents in algebra?

+

Common exponent mistakes include: • Confusing (-a)ⁿ with -aⁿ • Forgetting that a⁰ = 1 for any non-zero number • Not following order of operations (parentheses before exponents) • Incorrectly distributing exponents over addition: (a+b)² ≠ a² + b²

How do algebraic methods help solve equations?

+

Algebraic methods like distributive property and factoring help isolate variables by simplifying expressions. They allow you to clear parentheses, combine like terms, and manipulate equations systematically to find solutions.

What grade level learns these algebraic techniques?

+

Students typically learn the distributive property around age 12 (6th-7th grade), factoring in 8th-9th grade, and extended distributive property in Algebra I (9th-10th grade). These form the foundation for advanced algebraic problem-solving.

Practice by Question Type

Choose your preferred learning style and practice format for fraction problems

Applying the formula Ascertain whether the law of distributive property is applicable Complete the missing number Distributive property in geometry Is the equality correct? Matching expressions equal in value Solving an equation using the extended distributive law Solving the problem Using additional geometric shapes Using a rectangle Using variables Applying the formula Complete the missing number Decomposing a Number into smaller numbers/parts Extracting a Common Factor from a group of numbers following decomposition Identifying a Common Factor of a number following decomposition Matching expressions equal in value Number of terms Solving the problem Third power

Algebraic Method Practice Problems with Solutions

Understanding Algebraic Technique