Frequently Asked Questions
Everything you need to know about Order or Hierarchy of Operations with Fractions
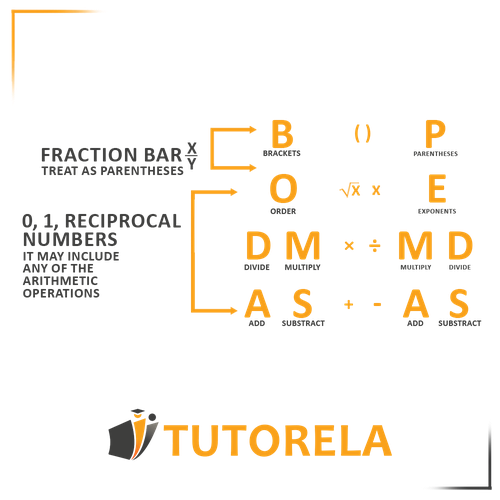
Do fractions change the order of operations rules?
+ No, fractions do not change the order of operations. You treat fractions like any other number and follow PEMDAS/BODMAS: parentheses first, then multiplication and division from left to right, then addition and subtraction from left to right.
How do you handle fraction bars in order of operations?
+ Fraction bars act as grouping symbols, similar to parentheses. You must complete all operations in the numerator and denominator separately before dividing. This gives fraction bars higher priority than regular division symbols.
What happens when you multiply or divide by 0 in order of operations?
+ When multiplying any number by 0, the result is always 0, regardless of other operations. Division by 0 is undefined and cannot be performed. These special cases follow normal order of operations timing.
How do you solve order of operations with reciprocals?
+ Reciprocals (like 1/3 or inverse fractions) follow the same order of operations rules. Multiply by the reciprocal instead of dividing by the original fraction. For example, ÷(2/3) becomes ×(3/2).
Why does multiplying by 1 not change the order of operations?
+ Multiplying by 1 gives the identity property - any number times 1 equals itself. This doesn't change the order of operations, but it can simplify expressions. You still perform multiplication at the correct step in PEMDAS/BODMAS.
What's the correct order for: 3 + 6 × 1/3?
+ Follow PEMDAS: multiplication before addition. First calculate 6 × 1/3 = 2, then add: 3 + 2 = 5. The fraction 1/3 is treated as a regular number in the multiplication step.
How do you handle decimal fractions in order of operations?
+ Decimal fractions (like 0.3 or 0.5) follow identical order of operations rules as common fractions. There's no difference in priority - both are simply numbers that get processed according to PEMDAS/BODMAS timing.
When do you simplify fractions during order of operations?
+ Simplify fractions after completing each operation step, not before following order of operations. For example, if you get 8/8 during multiplication step, simplify it to 1, then continue with the next operation in sequence.