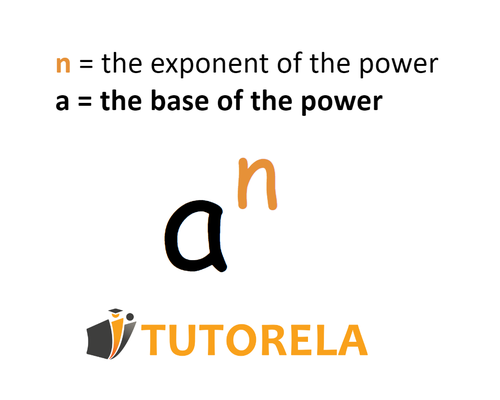
Powers are the number that is multiplied by itself several times.
Each power consists of two main parts:
- Base of the power: The number that fulfills the requirement of duplication. The principal number is written in large size.
- Exponent: the number that determines how many times the power base needs to be multiplied by itself.
The exponent is written in small size and appears on the right side above the power base.