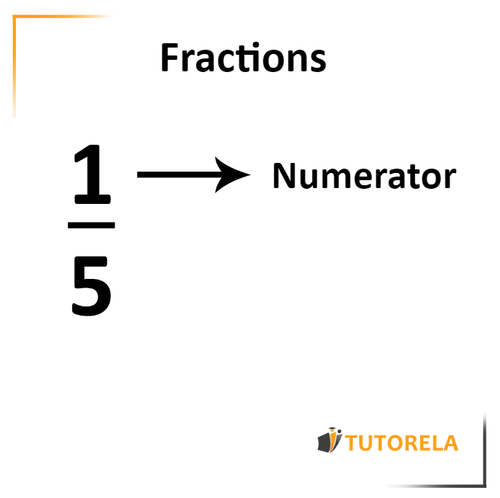
What is the numerator? The numerator is the top number of a fraction and represents the portion within the whole part.
Numerator Practice Problems & Exercises with Solutions
Master numerators in fractions with step-by-step practice problems. Learn to identify, write, and understand numerators with interactive exercises and solutions.
- Identify numerators in simple fractions like 1/3, 5/8, and 2/4
- Write fractions with specific numerator values (1, 2, 3, etc.)
- Understand how numerators represent parts within a whole
- Distinguish between numerator and denominator positions in fractions
- Solve practice problems involving numerator identification
- Apply numerator concepts to real-world fraction scenarios
Understanding Numerator
Numerator
For example:
Practice Numerator
Write the fraction shown in the diagram as a number:
Examples with solutions for Numerator
What is the marked part?
Let's begin:
Step 1: Upon examination, the diagram divides the rectangle into 7 vertical sections.
Step 2: The entire shaded region spans the full width, essentially covering all sections, so the shaded number is 7.
Step 3: The fraction of the total rectangle that is shaded is .
Step 4: Simplifying, becomes .
Therefore, the solution is marked by the choice: Answers a + b.
Answer:
Answers a + b
What is the marked part?
Let's solve this problem step-by-step:
First, examine the grid and count the total number of sections. Observing the grid, there is a total of 6 columns, each representing equal-sized portions along the grid, as evidenced by vertical lines.
Next, count how many of these sections are colored. The entire portion from the first column to the fourth column is colored. This means we have 4 out of 6 sections that are marked red.
We can then express the colored area as a fraction: .
Answer:
Write the fraction shown in the picture, in words:
Step 1: Count the total sections
The circle is divided into 8 equal sections.
Step 2: Count the shaded sections
There are 6 shaded sections in the diagram.
Step 3: Formulate the fraction
The fraction of the shaded area is .
Step 4: Express in words
The fraction in words is "six eighths".
Therefore, the solution to the problem is "six eighths".
Answer:
Six eighths
Write the fraction shown in the drawing, in numbers:
The number of parts in the circle represents the denominator of the fraction, and the number of colored parts represents the numerator.
The circle is divided into 12 parts, 6 parts are colored.
Answer:
Write the fraction shown in the drawing, in numbers:
The number of parts in the circle represents the denominator of the fraction, and the number of colored parts represents the numerator.
The circle is divided into 3 parts, 1 part is colored.
Hence:
Answer: