The integer is multiplied by the denominator. The obtained product is then added to the numerator. The final result is placed as the new numerator.
Nothing is changed in the denominator.
A fraction greater than is a fraction whose numerator is larger than the denominator.
Mixed Numbers and Fractions Greater Than 1
How do you convert a mixed number to a fraction?
Test yourself on mixed numbers and fractions greater than 1!
Write the fraction as a mixed number:
\( \frac{10}{7}= \)
Fraction greater than one
In this article, we will learn everything necessary about mixed numbers and fractions greater than .
We will learn how to convert everything to a fraction, subtract, add, multiply, and compare. All in an easy and efficient way.
What is a mixed number?
A mixed number is a number made up of a whole number and a fraction - hence its name - it combines whole numbers and fractions.
Examples of mixed numbers:
, ,
Write the fraction as a mixed number:
\( \frac{12}{10}= \)
Write the fraction as a mixed number:
\( \frac{10}{6}= \)
Write the fraction as a mixed number:
\( \frac{7}{4}= \)
How do you convert a mixed number to a fraction?
Let's see it by practicing
Let's look at this mixed number
To find the numerator we multiply the whole number by the denominator. To the product obtained we add the numerator.
Nothing is modified in the denominator.
We will obtain:
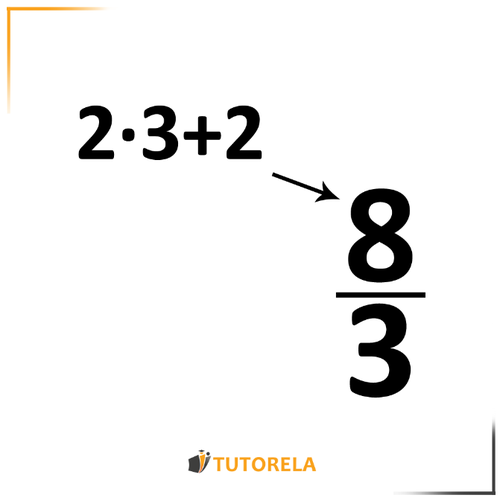
What does a fraction that is greater than 1 look like?
First, let's see what a fraction equivalent to looks like.
A fraction equivalent to is one whose numerator and denominator are equal. For example, or .
A fraction greater than is a fraction whose numerator is larger than the denominator.
Whenever the numerator is larger than the denominator, the fraction will be greater than . For example,
Observe:
Every mixed number is greater than and we can write it in the form of a fraction that is greater than .
Practice:
Convert the mixed number to a fraction greater than .
Solution:
We will multiply the whole number by the denominator and add the numerator to the product. We will write the result in the numerator
The denominator will not be altered.
We obtain:
It is clear that the fraction obtained is greater than –> the numerator is larger than the denominator.
Write the fraction as a mixed number:
\( \frac{8}{5}= \)
Write the fraction as a mixed number:
\( \frac{16}{10}= \)
Write the fraction as a mixed number:
\( \frac{13}{9}= \)
How do you convert a fraction greater than 1 into a mixed number?
In certain cases, when we want to find out the number of units or just to order the final result, we prefer to convert a fraction greater than to a mixed number.
We will do it in the following way:
We will calculate how many whole times the numerator fits into the denominator - this will be the whole number.
What remains, we will write in the numerator, and the denominator will remain unchanged (does not change).
Let's learn by practicing:
Here is a fraction greater than :
To convert it to a mixed number we will divide the numerator by the denominator. Let's ask ourselves how many whole times fits into ?
We will obtain:
3 times -> this will be the whole number of the result.
Now let's see what remains to complete the numerator .
What is the remainder?
We have a remainder of , that is what is placed in the numerator.
The final result is:
Addition and Subtraction
When we talk about addition and subtraction of fractions, the first step is to convert everything to fractions (without whole numbers).
This way we can reach the common denominator and then add or subtract the numerators.
Write the fraction as a mixed number:
\( \frac{12}{8}= \)
Write the fraction as a mixed number:
\( \frac{17}{11}= \)
Write the fraction as a mixed number:
\( \frac{13}{11}= \)
For example
Solution:
Given this addition exercise with a fraction larger than and a mixed number.
The first step is to convert the mixed number to a fraction in the way we have learned before.
It will give us:
Let's rewrite the exercise:
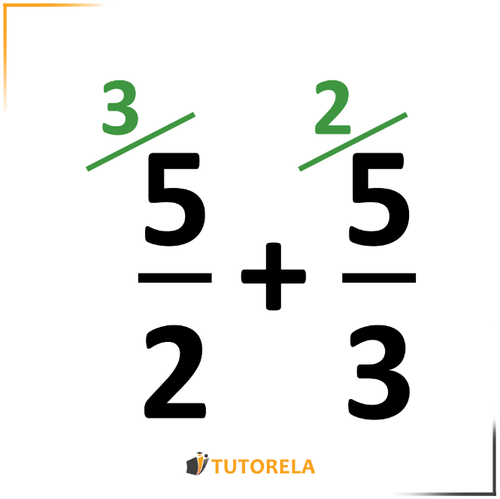
Now we will find the common denominator by multiplying the denominators and we will get:
We can convert the result to a mixed number like this:
Multiplication and Division
When we talk about multiplication and division, indeed, there is no need to find the common denominator, but it is necessary to convert mixed numbers into fractions.
This way, the operations will be carried out easily.
Comparison between a mixed number and a fraction greater than
To be able to compare a mixed number and a fraction greater than ,
The first thing we must do is, clearly, convert the mixed number to a fraction -> that is, a fraction with numerator and denominator.
Then, find the common denominator, only after this can we compare the numerators.
Let's practice:
Mark the corresponding sign
_______________
Solution:
We will convert the mixed number to a fraction and rewrite the exercise.
We will obtain:

We will find the common denominator by multiplying the denominators and we will obtain:
_______>________
Examples and exercises with solutions of mixed number and fraction greater than 1
Exercise #1
Write the fraction as a mixed number:
Video Solution
Step-by-Step Solution
To solve the problem, we will convert the given improper fraction to a mixed number by dividing the numerator by the denominator.
Step 1: Divide the numerator (10) by the denominator (7). This gives a quotient and a remainder.
Step 2: Calculating gives a quotient of 1 because 7 goes into 10 once.
Step 3: Multiply the quotient by the divisor ().
Step 4: Subtract the product obtained in step 3 from the original numerator to find the remainder: .
Step 5: Compose the mixed number using the quotient as the whole number and the remainder over the divisor as the fraction part: .
Thus, the mixed number representation of is .
Answer
Exercise #2
Write the fraction as a mixed number:
Video Solution
Step-by-Step Solution
To solve this problem, we need to convert the improper fraction into a mixed number.
Here's how we'll do it:
- The first step is to divide the numerator by the denominator: .
- This division gives us a quotient of 1 and a remainder of 4.
- The quotient, 1, becomes the whole number part of our mixed number.
- The remainder is used as the new numerator over the original denominator to form the fractional part: .
- The mixed number is thus .
- Finally, since can be simplified, we reduce it to .
Thus, the mixed number representation is correctly simplified as .
However, when selecting from the given choices, the correct choice based on the options provided is (Choice 4), which matches the unsimplified form.
Therefore, the solution to the problem is .
Answer
Exercise #3
Write the fraction as a mixed number:
Video Solution
Step-by-Step Solution
To convert the improper fraction into a mixed number, we need to perform division to separate the whole number from the fractional part.
- Step 1: Divide the numerator by the denominator. - Perform the division: . Since 13 is not a multiple of 11, we obtain a quotient and a remainder. - The division gives a quotient of 1 and a remainder of 2 (since ).
- Step 2: Express the remainder as part of the fraction. - The remainder is 2, and this will be the numerator of the fractional part. - The denominator remains the same, 11.
- Step 3: Write the mixed number. - Combine the whole number and the fraction. - The mixed number is .
Now, let's verify our solution with the given choices. The correct option matches Choice 2, which is .
Therefore, the fraction as a mixed number is .
Answer
Exercise #4
Write the fraction as a mixed number:
Video Solution
Step-by-Step Solution
To convert the improper fraction into a mixed number, we follow these steps:
- Step 1: Perform the division of the numerator by the denominator. Divide 13 by 9.
- Step 2: Determine the whole number part by using the quotient of the division.
- Step 3: Find the remainder to establish the fractional part.
- Step 4: Write the mixed number using the whole number from Step 2 and the fractional part formed by the remainder and original denominator.
Let's carry out these steps in detail:
Divide 13 by 9:
with a remainder of .
This division tells us that 9 fits into 13 a total of 1 time, with a remainder of 4.
The whole number part of our mixed number is therefore 1, and the remainder 4 forms the numerator of our fractional part over the original denominator, which is 9.
So, the fractional part is .
Therefore, the improper fraction as a mixed number is .
Answer
Exercise #5
Write the fraction as a mixed number:
Video Solution
Step-by-Step Solution
To solve the problem of converting the fraction to a mixed number, we proceed with the following steps:
- Step 1: Identify the numerator (16) and the denominator (10).
- Step 2: Divide the numerator by the denominator to find the whole number part.
Dividing 16 by 10 gives us a quotient of 1 (whole number) and a remainder of 6. - Step 3: Express the result as a mixed number.
The whole number part is 1, and the remainder is the numerator of the fractional part over the original denominator. This is . - Step 4: Write the final mixed number as: .
Therefore, the mixed number form of the fraction is .
Answer
Write the fraction as a mixed number:
\( \frac{6}{2}= \)
Write the fraction shown in the drawing:
Write the fraction shown in the drawing:
More Questions
Mixed Numbers and Fractions Greater than 1
- Visual Representation of the Fraction 3/4: Choose the Correct Diagram
- Visual Representation of the Fraction 8/40: Match the Correct Diagram
- Visual Representation of the Fraction 9/6: Choose the Correct Model
- Visual Representation of the Fraction 25/15: Choose the Correct Diagram
- Visual Representation of Improper Fraction 27/12: Choose the Correct Image
- The Order of Basic Operations: Addition, Subtraction, and Multiplication
- Order of Operations: Exponents
- Order of Operations: Roots
- Division and Fraction Bars (Vinculum)
- The Numbers 0 and 1 in Operations
- Neutral Element (Identity Element)
- Order of Operations with Parentheses
- Order or Hierarchy of Operations with Fractions
- Opposite numbers
- Elimination of Parentheses in Real Numbers
- Addition and Subtraction of Real Numbers
- Multiplication and Division of Real Numbers
- Multiplicative Inverse
- Integer powering
- Positive and negative numbers and zero
- Real line or Numerical line
- Addition and Subtraction of Mixed Numbers
- Multiplication of Integers by a Fraction and a Mixed Number
- Order of Operations - Exponents and Roots
- Special cases (0 and 1, reciprocals, fraction line)









