Solving algebraic equations is made easier by understanding some basic rules and properties. A few examples of properties that we will learn to use in the seventh grade are: the distributive, associative and commutative properties. These properties get learned and relearned throughout our time in school, each time adding new layers to or understanding. Today we will focus on the distributive property. We will go into depth on what it is and how to use it, and we will briefly get to know the associative and commutative properties as well.
Distributive Property Practice Problems for 7th Grade Math
Master the distributive property with step-by-step practice problems. Learn to simplify algebraic expressions using multiplication and division exercises for middle school.
📚What You'll Master in This Practice Session
- Apply distributive property to simplify multiplication with large numbers like 7 × 96
- Break down division problems using distributive property such as 294 ÷ 3
- Solve algebraic expressions with variables like (X + 5) × (X + 6)
- Work with two sets of parentheses using (Z + T) × (X + Y) format
- Combine like terms after distributing multiplication across addition and subtraction
- Solve real-world word problems involving equal groups and distribution
Understanding The Distributive Property for 7th Grade
Complete explanation with examples
What is the distributive property?
The distributive property is a method to simplify multiplication and division exercises. Essentially, it breaks down expressions into smaller, easier to manage terms.
Let's see some examples:
If we look at the following examples, we can see that we have broken down the larger number into several smaller numbers that are more manageable. The value is the same as before, but now we can distribute a complex operation into several easy operations.
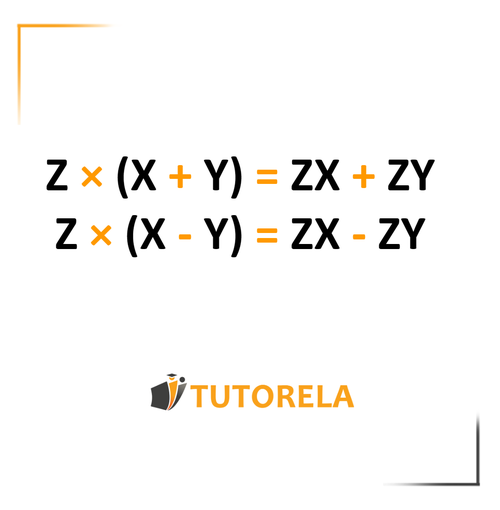
The distributive property can be described as:
Practice The Distributive Property for 7th Grade
Test your knowledge with 25 quizzes
Which equation is the same as the following?
\( 160\times6 \)
Examples with solutions for The Distributive Property for 7th Grade
Step-by-step solutions included
Exercise #1
Step-by-Step Solution
In order to simplify the calculation , we first break down 94 and 72 into smaller and preferably round numbers.
We obtain the following exercise:
Using the associative property, we then rearrange the exercise to be more functional.
We solve the exercise in the following way, first the round numbers and then the small numbers.
Which results in the following exercise:
Answer:
166
Video Solution
Exercise #2
Step-by-Step Solution
To solve the problem, first we will use the distributive property on the two numbers:
(60+3)-(30+6)
Now, we will use the substitution property to arrange the exercise in the way that is most convenient for us to solve:
60-30+3-6
It is important to pay attention that when we open the second parentheses, the minus sign moved to the two numbers inside.
30-3 =Â
27
Answer:
27
Video Solution
Exercise #3
Step-by-Step Solution
We will use the distributive law and split the number 143 into a sum of 100 and 43.
The distributive law allows us to distribute, meaning, to split a number into two or more numbers. This actually allows us to work with smaller numbers and simplify the operation.
We will operate according to the order of operations
We can remove parentheses and perform addition and subtraction operations in any order since there are only addition and subtraction operations in the equation
Therefore, the answer is option C - 100.
And now let's see the solution to the exercise in a centered format:
Answer:
100
Video Solution
Exercise #4
Step-by-Step Solution
In order to solve the question, we first use the distributive property for 133:
We then use the distributive property for 33:
We rearrange the exercise into a more practical form:
We solve the middle exercise:
Which results in the final exercise as seen below:
Answer:
163
Video Solution
Exercise #5
Step-by-Step Solution
In order to simplify the resolution process, we begin by using the distributive property for 140:
We then rearrange the exercise using the substitution property into a more practical form:
Lastly we solve the exercise from left to right:
Answer:
70
Video Solution
Frequently Asked Questions
What is the distributive property in 7th grade math?
+The distributive property allows you to multiply a number by a sum or difference by distributing the multiplication to each term inside parentheses. For example, 6 × (20 + 6) = 6 × 20 + 6 × 6 = 120 + 36 = 156.
How do you use distributive property with variables?
+With variables, you distribute multiplication across each term in parentheses. For instance, (X + 5) × (X + 6) becomes X² + 6X + 5X + 30, which simplifies to X² + 11X + 30 by combining like terms.
Can you use distributive property for division problems?
+Yes! You can break down the dividend into smaller, more manageable numbers. For example, 294 ÷ 3 = (300 - 6) ÷ 3 = 300 ÷ 3 - 6 ÷ 3 = 100 - 2 = 98.
What's the difference between distributive and commutative properties?
+The distributive property involves distributing multiplication over addition or subtraction within parentheses. The commutative property simply changes the order of terms (like 2 + 6 = 6 + 2) without involving parentheses or distribution.
How do you solve two parentheses using distributive property?
+Multiply each term in the first parentheses by each term in the second parentheses. For (X + 2) × (X + 3): multiply X by X and 3, then multiply 2 by X and 3, giving X² + 3X + 2X + 6 = X² + 5X + 6.
What are common mistakes when using distributive property?
+Common errors include: 1) Forgetting to distribute to all terms inside parentheses, 2) Making sign errors with subtraction, 3) Not combining like terms after distributing, and 4) Confusing distributive with other properties like associative or commutative.
When should 7th graders use distributive property?
+Use distributive property when: multiplying large numbers (like 7 × 96), simplifying algebraic expressions with parentheses, solving word problems involving equal groups, and breaking down complex calculations into easier steps.
How does distributive property help with mental math?
+It breaks large numbers into friendlier calculations. Instead of computing 15 × 9 directly, you can use 15 × (10 - 1) = 15 × 10 - 15 × 1 = 150 - 15 = 135, making mental calculation much easier.