A square root of a number is a value that, when multiplied by itself, gives the original number. For example, the principal square root of is , because .
The square root symbol is written as .
for example:
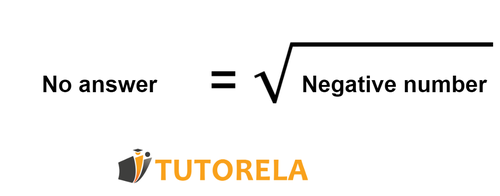
Note that we typically work with non-negative numbers when finding square roots in the real number system.
Square root is basically the inverse operation of a power. Even so, it can be written as a power! Square root is equal to a power of .
If a small number appears to the left of the square root symbol, it indicates the order of the root, also known as the index. For example, in , the small number tells us it is a cube root, meaning we are looking for a number that, when multiplied by itself three times, equals . When no number is shown, it is understood to be a square root (index of ).