Simplifying Expressions (Collecting Like Terms)

The simplification of expressions consists of creating an equivalent expression written in a shorter and simpler way in which we combine all of the similar terms (collecting like terms).

For example, the expression:

$3+3+3+3+3+5X-3X$

After having simplified it, it would be:

$15+2X$

What we have done is created two groups of numbers and variables:
$3+3+3+3+3$ and $5X-3X$ .

This can be simplified further, resulting in only two terms: $15+2X$

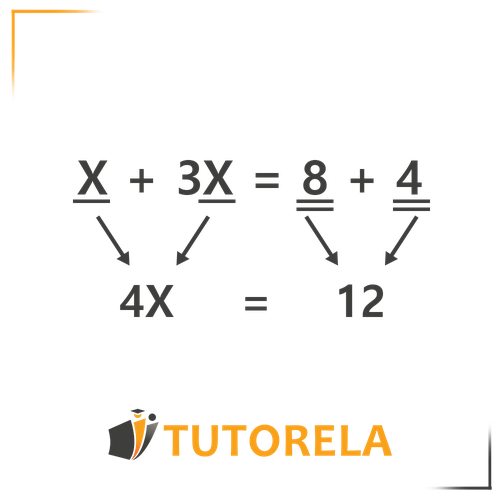
Solving a basic algebraic equation: X + 3X = 8 + 4. Step-by-step breakdown of combining like terms on both sides to get 4X = 12. Fundamental algebra simplification process.

Detailed explanation

Start practice

Test yourself on variables and algebraic expressions!

$$ 11+5x-2x+8= $$

Practice more now

After having studied what algebraic expressions and equivalent algebraic expressions are, the next thing to do is to understand how to collect like terms.

Since the numbers and variables are not similar (or, 'like') terms, they cannot be simplified into a single group and, therefore, we have to write them separately ( $X,Y$ ).

Example with Two Variables

The expression $4+2+2X+3X+Y+2Y=$ can be simplified as follows:

$5X+3Y+6$

Practice Exercises: Collecting Like Terms

Combine like terms in order to obtain shorter expressions:

$X+X=$
$5+8-9+5X-4X=$
$5+0+8X-5=$
$11+5X-2X+8=$
$13X+5-4.5X+7.5X=$

Collect like terms to obtain shorter expressions. Then, using what we have learned about the numerical value of algebraic expressions, apply $X=5$ and solve.

$2X+5X\cdot4=$
$2.3X+0.4X-0.7X=$
${X\over15}+{X\over15}=$
${3\over8}X-{2\over8}X+5=$
$(7+Y):3=$

If you are interested in this article, you may also like the following articles:

On Tutorela website, you can find a wide range of useful articles about mathematics!

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

$$ 5+0+8x-5= $$

Question 2

$$ 5+8-9+5x-4x= $$

Question 3

$$ x+x= $$

Exercises: Collecting Like Terms

Exercise 1

Task:

$3\frac{b}{a}\cdot1\frac{3}{8}a+\frac{5}{8}b+\frac{4}{18}m+\frac{9}{10}a+\frac{2}{3}m=\text{?}$

Solution:

Enter the corresponding elements.

$3\frac{b}{a}\times1\frac{3}{8}a+\frac{5}{8}b+\frac{9}{10}a+\frac{4}{18}m+\frac{2}{3}m=$

Convert the mixed fractions into improper fractions.

$3\frac{b}{a}\times\frac{(8+3)}{8}a+\frac{5}{8}b+\frac{9}{10}a+\frac{4}{18}m+\frac{2}{3}m=$

Solve accordingly.

$\frac{3\times11\times b\times a}{8\times a}+\frac{5}{8}b+\frac{9}{10}a+\frac{4+2\times6}{18}m=$

Simplify to $a$ in the equation.

$\frac{33}{8}b+\frac{5}{8}b+\frac{9}{10}a+\frac{16}{18}m=$

$\frac{33+5}{8}b+\frac{9}{10}a+\frac{8}{9}m=$

$\frac{38}{8}b+\frac{9}{10}a+\frac{8}{9}m=$

$4\frac{3}{4}b+\frac{9}{10}a+\frac{8}{9}m=$

Answer:

$4\frac{3}{4}b+\frac{9}{10}a+\frac{8}{9}m=$

Exercise 2

Task:

$\frac{3}{8}a+\frac{14}{9}b+1\frac{1}{9}b+\frac{6}{8}a=\text{?}$

Solution:

First, group the terms together:

$\frac{3}{8}a+\frac{6}{8}a+\frac{14}{9}b+1\frac{1}{9}b$

Then reduce in correspondence and convert the mixed fractions into improper fractions.

$\frac{3+6}{8}a+\frac{10}{9}b+\frac{14}{9}b=$

$\frac{9}{8}a+\frac{10+14}{9}b=$

$1\frac{1}{8}a+\frac{24}{9}b=$

$1\frac{1}{8}a+2\frac{6}{9}b=$

$1\frac{1}{8}a+2\frac{2}{3}b$

Answer:

$1\frac{1}{8}a+2\frac{2}{3}b$

Do you know what the answer is?

Question 1

Are the expressions the same or not?

$$ 18x $$

$$ 2+9x $$

Question 2

Are the expressions the same or not?

$$ 3+3+3+3 $$

$3\times4$

Question 3

Are the expressions the same or not?

$$ 20x $$

$2\times10x$

Exercise 3

$7.3\times4a+2.3+8a=?$

Solution:

We start with the multiplication operation.

$(7.3\times4a)+2.3+8a=$

$(29.2a)+2.3+8a=$

Then we add together as much as we can and rewrite the equation to make it clearer:

$29.2a+8a+2.3=$

$37.2a+2.3=$

Answer:

$37.2a+2.3$

Exercise 4

Task:

Solve the following equation:

$a+b+bc+9a+10b+3c=\text{?}$

Solution:

The terms are substituted into the expression according to the order: $a, b, c$ .

$a+9a+b+bc+10b+3c=$

We continue with the addition operations.

$10a+11b+bc+3c=$

The terms containing $c$ are converted for the equation since they cannot be simplified further.

$10a+11b+(b+3)c$

Answer:

$10a+11b+(b+3)c$

Check your understanding

Question 1

$3x+4x+7+2=\text{?}$

Question 2

$3z+19z-4z=\text{?}$

Question 3

Are the expressions the same or not?

$$ 15x-30 $$

$$ 45-15-5x+15x $$

Exercise 5

Task:

Solve the following equation:

$3z+19z-4z=\text{?}$

Solution:

We start with the addition operation:

$22z-4z=$

We continue solving accordingly.

$18z$

Answer:

$18z$

Review Questions

What are like terms?

Like terms in an algebraic expression are those that have the same variable with the same exponent, regardless of the sign and the coefficient—that is, the sign and the coefficient can be different, but the variable and the exponent must be the same. For example:

$3x^2$ y $-11x^2$

$8a^5$ y $8a^5$

$-7m$ y $\frac{2}{3}m$

How do you simplify expressions?

In order to simplify algebraic expressions, we need to work out if there are any like terms and then group them together before performing the operations (addition, subtraction, etc.).

Example 1

Task: Simplify the following expression:

$3x^2-7x+6-5x^2-x-1$

Solution:

First we need to group the like terms and then perform the operations.

$3x^2-5x^2-7x-x+6-1$

$-2x^2-8x+5$

Answer:

$-2x^2-8x+5$

Example 2

Task: Simplify the following expression:

$8m^2+2m+7=3m^3+5m^2+2m-5$

Solution:

In this case we need to put all the terms on one side and combine the like terms:

$-3m^3+8m^2-5m^2+2m-2m+7+5=$

$-3m^3+3m^2+12=$

Answer:

$-3m^3+3m^2+12=$

How do you simplify a function?

In order to simplify a function, we must also work out if there are any like terms and group them together in order to simplify them.

Example

Question: Simplify the following function:

$f\left(a\right)=-5a^2+2a-4+2a^2+5a$

Solution:

In this function we can see that there are like terms, so we can group them to simplify them.

$f\left(a\right)=-5a^2+2a^2+2a+5a-4$

$f\left(a\right)=-3a^2+7a-4$

Answer:

$f\left(a\right)=-3a^2+7a-4$

Do you think you will be able to solve it?

Question 1

Are the expressions the same or not?

$0.5x\times1$

$$ 0.5x+0 $$

Question 2

$7a+8b+4a+9b=\text{?}$

Question 3

$18x-7+4x-9-8x=\text{?}$

Examples with solutions for Simplifying Expressions (Collecting Like Terms)

Exercise #1

$3x+4x+7+2=\text{?}$

Video Solution

Step-by-Step Solution

Let's simplify the expression $3x + 4x + 7 + 2$ step-by-step:

Step 1: Combine Like Terms Involving $x$
The terms $3x$ and $4x$ are like terms because both involve the variable $x$ . To combine them, add their coefficients:
$3x + 4x = (3 + 4)x = 7x$
Step 2: Combine Constant Terms
The expression includes constant terms $7$ and $2$ . These can be added together to simplify:
$7 + 2 = 9$
Step 3: Write the Simplified Expression
Now, combine the results from Step 1 and Step 2 to form the final simplified expression:
$7x + 9$

Therefore, the simplified expression is $7x + 9$ .

Reviewing the choices provided, the correct choice is:

Choice 2: $7x + 9$

This matches our simplified expression, confirming our solution is correct.

Answer

$7x+9$

Exercise #2

Are the expressions the same or not?

$18x$

$2+9x$

Video Solution

Step-by-Step Solution

To determine if the expressions $18x$ and $2 + 9x$ are equivalent, we'll analyze their structures.

$18x$ is a linear expression with a single term involving the variable $x$ , and its coefficient is 18.
$2 + 9x$ consists of two terms: a constant term $2$ and a linear term $9x$ with coefficient 9.

For two expressions to be equivalent, each corresponding term must be equal. Here, the expression $18x$ has no constant term, whereas $2 + 9x$ has a constant term of 2. Furthermore, the linear term coefficients differ: $18 \neq 9$ .

Therefore, the expressions $18x$ and $2 + 9x$ are not the same. They structurally differ and cannot be made equivalent just through similar values of $x$ .

Therefore, the solution to this problem is: No.

Answer

Exercise #3

$5+0+8x-5=$

Video Solution

Step-by-Step Solution

To simplify the expression $5 + 0 + 8x - 5$ , follow these steps:

Step 1: Identify and group like terms. In this case, there are constants (5, 0, -5) and a term with a variable (8x).
Step 2: Combine the constants: $5 + 0 - 5$ .
Step 3: Calculate: $5 - 5 = 0$ .

Now, our expression simplifies to $0 + 8x$ , which is simply $8x$ .

Therefore, the simplified expression is $8x$ .

Answer

$8X$

Exercise #4

$x+x=$

Video Solution

Step-by-Step Solution

To solve this algebraic problem, follow these steps:

Step 1: Identify the coefficients of the variable $x$ in the expression $x + x$ . Here, the coefficient for each $x$ is 1.
Step 2: Add the coefficients together. This gives $1 + 1 = 2$ .
Step 3: Multiply the result by the variable. This results in $2x$ .

Since the problem is a multiple-choice question, review the available choices to select the correct answer. The expression simplifies to $2x$ , which corresponds to choice 3: $2x$ .

Therefore, the solution to the problem is $2x$ .

Answer

$2x$

Exercise #5

Are the expressions the same or not?

$3+3+3+3$

$3\times4$

Video Solution

Step-by-Step Solution

To solve this problem, we'll analyze the expressions $3+3+3+3$ and $3 \times 4$ to determine if they are equivalent.

First, evaluate the expression $3+3+3+3$ :

Add the numbers: $3 + 3 = 6$
Add again: $6 + 3 = 9$
Add the last $3$ : $9 + 3 = 12$

The result of $3+3+3+3$ is $12$ .

Next, evaluate the expression $3 \times 4$ :

Perform the multiplication: $3 \times 4 = 12$

The result of $3 \times 4$ is also $12$ .

Since both expressions result in the same number, we conclude that

The expressions are the same.

Therefore, the correct answer is Yes.

Answer

Yes

Start practice

Simplifying Expressions (Collecting Like Terms)

Test yourself on variables and algebraic expressions!

Practice Exercises: Collecting Like Terms

Exercises: Collecting Like Terms

Exercise 1

Exercise 2

Exercise 3

Exercise 4

Exercise 5

Review Questions

Examples with solutions for Simplifying Expressions (Collecting Like Terms)

Exercise #1

Video Solution

Step-by-Step Solution

Answer

Exercise #2

Video Solution

Step-by-Step Solution

Answer

Exercise #3

Video Solution

Step-by-Step Solution

Answer

Exercise #4

Video Solution

Step-by-Step Solution

Answer

Exercise #5

Video Solution

Step-by-Step Solution

Answer

More Questions

Variables and Algebraic Expressions