Principles and methods for solving first-degree equations with one unknown
Examples and exercises
Exercise 1
Solve the following equation:
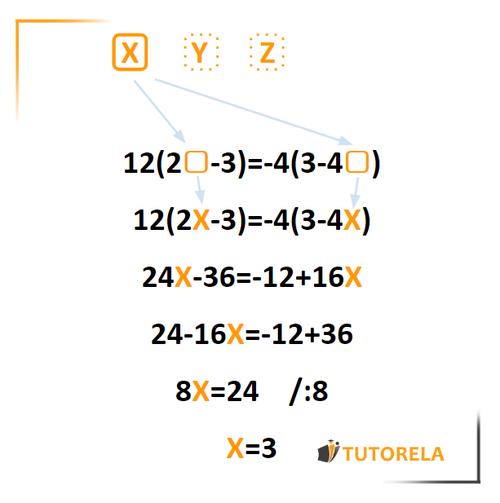
12(2X−3)=−4(3−4X)
Solution:
To solve the equation, we first make the products of the two sides of the equation:
24X−36=−12+16X
Next we will group the like terms, so that on the left side of the equation all the unknowns appear, while on the right side of the equation the numbers appear. Remember, when transposing the terms from one side of the equation to the other, their sign will change. That is, if it is adding, it will go to the other side subtracting, and vice versa.
24X−16X=−12+36
Then we reduce the like terms:
8X=24
Now, to find the value of the unknown, we divide both sides of the equation by 8 and get:
8X/8=24/8
X=3
Thus, X=3 is the solution of the equation.
Answer:
X=3
Exercise 2
Solve the following equation:
8(2−5X)−12(1−X)=0
To solve this equation, we first do the product of the left side of the equation, obtaining:
16−40X−12+12X=0
Next we group the like terms, so that on the left side of the equation all the unknowns appear, while on the right side of the equation the numbers will appear. Remember, when transposing the terms from one side of the equation to the other, the sign of the terms will change.
−40X+12X=12−16
The next step is to reduce the like terms:
−28X=−4
Now, to find the value of the unknown, we divide the two sides of the equation by (-28) and we will get:
−28X/−28=−4/−28
And finally we reduce the fraction:
X=284=71
Answer:
X=71
Join Over 30,000 Students Excelling in Math!
Endless Practice, Expert Guidance - Elevate Your Math Skills Today
Exercise 3
Solve the following equation:
−6(−X−1)+10(2−X)=16
To solve the equation, we first make the products of the two sides of the equation:
6X+6+20−10X=16
We then group the like terms together, so that on the left side of the equation all the unknowns appear, while on the right side of the equation the numbers appear. Remember, when transposing the terms from one side of the equation to the other, their sign will change. That is, if it is adding, it will go to the other side subtracting, and vice versa.
6X−10X=16−6−20
The next step is to reduce the like terms:
−4X=−10
Now, to find the value of the unknown, we divide both sides of the equation by (-4), and we will get:
−4X/−4=−−410
X=410=2.5
Answer:
X=2.5
Exercise 4
Solve the following equation:
321⋅y=21
Solution
Note that:
321=27
Thus the equation is equivalent to:
27⋅y=21
Now, we divide by 7/2 both sides of the equation and get:
y=2721=6
y=6
Answer
y=6
Do you know what the answer is?
Exercise 5
Solve the following equation:
431⋅x=2132
Solution
Note that:
431=313
y
2132=365
Thus, the equation is equivalent to:
313⋅x=365
Divide both sides of the equation by:
313
to simplify
x=313365
x=5
Answer
x=5
Exercise 6
Solve the following equation:
3x+4+x+1=9
Solution
Next we group the like terms, so that on the left side of the equation all the unknowns appear, while on the right side of the equation the numbers appear.
3x+x=9−4−1
We add the like terms:
4x=4
We divide both sides of the equation by 4
4x/4=4/4
Answer
x=1
Exercise 8
Solve the following problem:
What is the domain of application of the equation?
2(3+y)+4xyz=8
Solution
We must calculate when the denominator on the right hand side of the equation equals zero, i.e:
2(3+y)+4=0
We multiply by 2 in the two elements of the parentheses.
6+2y+4=0
We add accordingly
10+2y=0
We go to 10 to the right hand section
2y=−10
Divide by 2
y=−5
y=−5
If Y is equal to minus 5 then the denominator is equal to 0 and the exercise has no solution.
Answer
y=−5
Questions on the subject
What is a first degree equation with one unknown?
It is a mathematical expression consisting of an unknown or variable and numbers in which the value of the variable must be found, which is generally denoted by X.
Examples
a) 3x−5=2x+4.
b) 4−x=10.
c) 4(x−4)+2=2x.
Do you think you will be able to solve it?
How to solve first degree equations with one unknown?
Isolating the unknown, that is, leaving it alone somewhere in the equality.
What are first degree equations with two unknowns?
It is a mathematical expression consisting of two unknowns or variables and numbers in which the value of the variables must be found, which are generally denoted by X and Y.
How to clear an unknown?
Isolating the variable or unknown using operations such as addition, subtraction, multiplication and division.
Do you know what the answer is?
Examples with solutions for Linear Equations (One Variable)
Exercise #1
Solve for X:
3+x−2=7−3
Video Solution
Step-by-Step Solution
First, simplify both sides of the equation:
Left side: 3+x−2=1+x
Right side: 7−3=4
So the equation becomes:
1+x=4
Next, isolate x by subtracting 1 from both sides:
1+x−1=4−1
This simplifies to:
x=3
Answer
Exercise #2
Solve for X:
x−3+5=8−2
Video Solution
Step-by-Step Solution
First, simplify both sides of the equation:
Left side: x−3+5=x+2
Right side: 8−2=6
Now the equation is: x+2=6
Subtract 2 from both sides to isolate x:
x+2−2=6−2
Simplifying gives:
x=4
Answer
Exercise #3
Solve for X:
x+4−2=6+1
Video Solution
Step-by-Step Solution
First, simplify both sides of the equation:
Left side: x+4−2=x+2
Right side: 6+1=7
Now the equation is: x+2=7
Subtract 2 from both sides to isolatex:
x+2−2=7−2
Simplifying gives:
x=5
Answer
Exercise #4
Solve for X:
3−x=10−6
Video Solution
Step-by-Step Solution
First, simplify the right side of the equation:
10−6=4
Hence, the equation becomes 3−x=4.
Subtract 3 from both sides to isolate x:
3−x−3=4−3
This simplifies to:
−x=1
Divide by -1 to solve forx:
x=−1
Therefore, the solution is x=1.
Answer
Exercise #5
Solve for X:
8−x=11−3
Video Solution
Step-by-Step Solution
First, simplify the right side of the equation:
11−3=8
Hence, the equation becomes 8−x=8.
Subtract 8 from both sides to isolate x:
8−x−8=8−8
This simplifies to:
−x=0
Divide by -1 to solve for x:
x=0
Therefore, the solution is x=0.
Answer