Symmetry - Examples, Exercises and Solutions

The axis of symmetry in a parabola is the axis that passes through its vertex in such a way that if we folded the right side over the left side, both sides would appear joined.
Let's see it in an illustration:

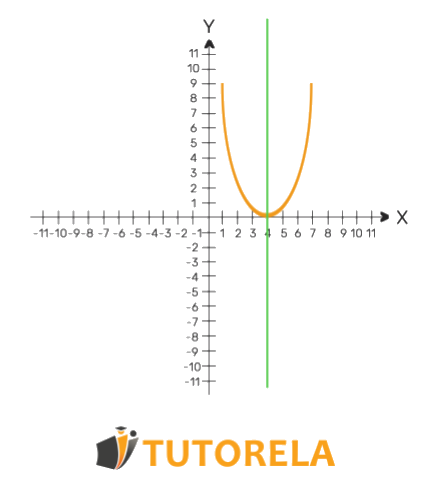
Symmetry 1

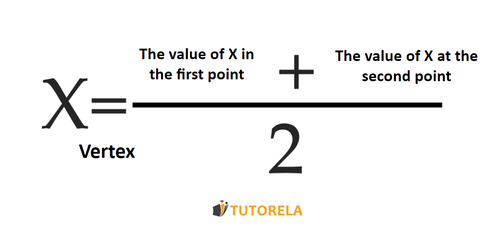
To find the axis of symmetry, we must locate the value of $X$ of the vertex of the parabola or do it through the parabola's vertex formula or with the help of two symmetric points on the parabola.

Vertex Formula of the Parabola

$X=\frac{-b}{2a}$

Formula for two symmetric points:

B3 - The formula to find X a vertex using two symmetric points

$X_{Vertex}=\frac{The~value~of~X~at~the~first~point~+~The~value~of~X~at~the~second~point}{2}$

Detailed explanation

Suggested Topics to Practice in Advance

Practice Symmetry

Question 1

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$\( f(x)=-3x^2+3 \)$

Question 2

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$\( f(x)=7x^2 \)$

Question 3

Given the expression of the quadratic function

Finding the symmetry point of the function

$\( f(x)=2x^2 \)$

Question 4

Given the expression of the quadratic function

Finding the symmetry point of the function

$\( f(x)=-5x^2+10 \)$

Question 5

Given the expression of the quadratic function

Finding the symmetry point of the function

$f(x)=\frac{1}{2}x^2$

examples with solutions for symmetry

Exercise #1

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$f(x)=-3x^2+3$

Video Solution

Answer

$x=0$

Exercise #2

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$f(x)=7x^2$

Video Solution

Answer

$x=0$

Exercise #3

Given the expression of the quadratic function

Finding the symmetry point of the function

$f(x)=2x^2$

Video Solution

Answer

$(0,0)$

Exercise #4

Given the expression of the quadratic function

Finding the symmetry point of the function

$f(x)=-5x^2+10$

Video Solution

Answer

$(0,10)$

Exercise #5

Given the expression of the quadratic function

Finding the symmetry point of the function

$f(x)=\frac{1}{2}x^2$

Video Solution

Answer

$(0,0)$

Question 1

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$\( f(x)=4x^2+6 \)$

Question 2

A quadratic equation is graphed below.

What is the axis of symmetry for the graph $\( f(x)=3x^2+2 \)$ ?

Question 3

Given the expression of the quadratic function

Finding the symmetry point of the function

$\( f(x)=3x^2+6x \)$

Question 4

Given the expression of the quadratic function

Finding the symmetry point of the function

$\( f(x)=3-5x^2 \)$

Question 5

Given the expression of the quadratic function

Finding the symmetry point of the function

$\( f(x)=3+3x^2 \)$

examples with solutions for symmetry

Exercise #1

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$f(x)=4x^2+6$

Video Solution

Answer

$x=0$

Exercise #2

A quadratic equation is graphed below.

What is the axis of symmetry for the graph $f(x)=3x^2+2$ ?

Video Solution

Answer

$x=0$

Exercise #3

Given the expression of the quadratic function

Finding the symmetry point of the function

$f(x)=3x^2+6x$

Video Solution

Answer

$(-1,-3)$

Exercise #4

Given the expression of the quadratic function

Finding the symmetry point of the function

$f(x)=3-5x^2$

Video Solution

Answer

$(0,3)$

Exercise #5

Given the expression of the quadratic function

Finding the symmetry point of the function

$f(x)=3+3x^2$

Video Solution

Answer

$(0,3)$

Question 1

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$\( f(x)=2x^2+8x+4 \)$

Question 2

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$\( f(x)=-2x^2+16 \)$

Question 3

Calculate the axis of symmetry of the quadratic function below:

$\( f(x)=3x^2+6x-6 \)$

Question 4

A quadratic function is graphed below.

What is the axis of symmetry for the graph $\( f(x)=x^2+4x \)$ ?

Question 5

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$\( f(x)=x^2+4x+1 \)$

examples with solutions for symmetry

Exercise #1

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$f(x)=2x^2+8x+4$

Video Solution

Answer

$x=-2$

Exercise #2

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$f(x)=-2x^2+16$

Video Solution

Answer

$x=0$

Exercise #3

Calculate the axis of symmetry of the quadratic function below:

$f(x)=3x^2+6x-6$

Video Solution

Answer

$x=-1$

Exercise #4

A quadratic function is graphed below.

What is the axis of symmetry for the graph $f(x)=x^2+4x$ ?

Video Solution

Answer

$x=-2$

Exercise #5

Given the expression of the quadratic function

The symmetrical axis of the expression must be found.

$f(x)=x^2+4x+1$

Video Solution

Answer

$x=-2$