Family of Parabolas y=(x-p)²

Family of Parabolas $y=(x-p)^2$

In this family, we have a slightly different quadratic function that shows us, very clearly, how the parabola shifts horizontally.
$P$ indicates the number of steps the parabola will move horizontally, to the right or to the left.
If $P$ is positive: (there is a minus sign in the equation) - The parabola will move $P$ steps to the right.
If $P$ is negative: (and, consequently, there will be a plus sign in the equation since minus by minus equals plus) - The parabola will move $P$ steps to the left.

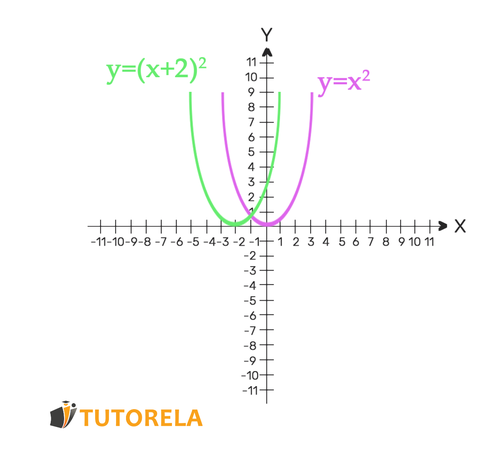
Let's see an example:
The function $Y=(X+2)^2$

shifts two steps to the left.
Let's see it in an illustration:

Detailed explanation

Start practice

Test yourself on parabola of the form y=(x-p)²!

Find the intersection of the function

$$ y=(x+4)^2 $$

With the Y

Practice more now

Change in Slope

When there is an $a$ before the parentheses, it indicates the slope of the parabola.
The larger the $a$ , the closer the parabola will be to its axis of symmetry. Steeper - with a smaller opening.
The smaller the $a$ , the farther the parabola will be from its axis of symmetry. Less steep - with a larger opening.

Let's see an example that shows the balance between the change in slope and horizontal shift:
For example, in the function

$Y=\frac{1}{2} (X-2)^2$

the changes will be:
Shift of $2$ steps to the right
and the change in slope, The parabola will be less steep and with a larger opening
Let's see it in an illustration:

Graphical and Algebraic Solution when $y=0$

Algebraic Solution

when $y=0$ the expression inside parentheses must be equal to $0$ .
$X$ must be equal to $P$ for the equation to be correct.

Graphical Solution

The graphical solution is the vertex of the parabola.
In a quadratic function of this form
$Y=(X-p)^2$
in which the coefficient of $X^2$ is $1$ ,
the vertex of the parabola is composed of $Y=0$ and by $P$ which indicates the $X$ vertex.
$(0,P)$
Note that, when in the equation there is a minus sign before the $P$
indeed, it is positive and, when there is a plus sign before the $P$ it is, in fact, negative.

Examples and exercises with solutions from the family of parabolas y=(x-p)²

Exercise #1

Find the intersection of the function

$y=(x+4)^2$

With the Y

Video Solution

Step-by-Step Solution

To solve this problem, we will find the intersection of the function with the Y-axis by following these steps:

Step 1: Recognize that the intersection with the Y-axis occurs where $x = 0$ .
Step 2: Substitute $x = 0$ into the function $y = (x+4)^2$ .
Step 3: Perform the calculation to find the y-coordinate.

Now, let's solve the problem:

Step 1: Identify the Y-axis intersection by setting $x = 0$ .
Step 2: Substitute $x = 0$ into the function:

$y = (0+4)^2 = 4^2 = 16$

Step 3: The intersection point on the Y-axis is $(0, 16)$ .

Therefore, the solution to the problem is $(0, 16)$ .

Answer

$(0,16)$

Exercise #2

Find the intersection of the function

$y=(x-2)^2$

With the X

Video Solution

Step-by-Step Solution

To solve this problem, we'll find the intersection of the function $y = (x-2)^2$ with the x-axis. The x-axis is characterized by $y = 0$ . Hence, we set $(x-2)^2 = 0$ and solve for $x$ .

Let's follow these steps:

Step 1: Set the function equal to zero:

$(x-2)^2 = 0$

Step 2: Solve the equation for $x$ :

Taking the square root of both sides gives $x - 2 = 0$ .

Adding 2 to both sides results in $x = 2$ .

Step 3: Find the intersection point coordinates:

The x-coordinate is $x = 2$ , and since it intersects the x-axis, the y-coordinate is $y = 0$ .

Therefore, the intersection point of the function with the x-axis is $(2, 0)$ .

The correct choice from the provided options is $(2, 0)$ .

Answer

$(2,0)$

Exercise #3

To find the y axis intercept, you substitute $x=0$ into the equation and solve for y.

Video Solution

Step-by-Step Solution

To determine if the given statement is true, consider the equation of the parabola $y = (x - p)^2$ . The y-intercept occurs where the parabola crosses the y-axis, which is at $x = 0$ .

Step 1: Substitute $x = 0$ into the equation:

$y = (0 - p)^2 = p^2$

Step 2: Calculate the y-intercept:

The y-intercept of the parabola is $y = p^2$ .

Conclusion: The statement "To find the y-axis intercept, you substitute $x = 0$ into the equation and solve for $y$ " is indeed True, as applying this method correctly determined the y-intercept for the given form of a parabola. Therefore, the answer to the problem is True.

Answer

True

Exercise #4

To which chart does the function $y=x^2$ correspond?

Video Solution

Step-by-Step Solution

To solve this problem, let's go through the process of elimination to find the graph corresponding to $y = x^2$ .

The function $y = x^2$ is an upward-opening parabola with its vertex located at the origin point (0, 0). It is symmetric about the y-axis.

Based on our problem statements or diagrams, the given function will match with chart '2'. This chart will depict an upward-facing parabolic shape with no horizontal or vertical shifts.

Therefore, the solution to the problem is the chart labeled 2.

Answer

Exercise #5

To work out the points of intersection with the X axis, you must substitute $x=0$ .

Video Solution

Step-by-Step Solution

To solve this problem, we need to determine whether substituting $x = 0$ gives us the points of intersection with the x-axis for the parabola $y = (x - p)^2$ .

To find the x-intercepts of any function, we set $y = 0$ because the x-intercepts occur where the curve meets the x-axis, which implies a zero output or function value:

Start with the equation: $y = (x - p)^2$ .
Set $y = 0$ for the x-intercept: $(x - p)^2 = 0$ .
Solving this gives $(x - p) = 0$ , which simplifies to $x = p$ .
Thus, the x-intercept happens at the point where $x = p$ , not where $x = 0$ .

Therefore, substituting $x = 0$ does not provide the x-intercepts. Instead, it provides the y-intercept. Therefore, the statement given in the problem is False.

Thus, the solution to the problem is False.

Answer

False

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

Find the intersection of the function

$$ y=(x-2)^2 $$

With the X

Question 2

To work out the points of intersection with the X axis, you must substitute $$ x=0 $$ .

Question 3

To find the y axis intercept, you substitute $$ x=0 $$ into the equation and solve for y.

Start practice

Family of Parabolas y=(x-p)²

Family of Parabolas $y=(x-p)^2$

Test yourself on parabola of the form y=(x-p)²!

Change in Slope

Graphical and Algebraic Solution when $y=0$

Graphical Solution

Examples and exercises with solutions from the family of parabolas y=(x-p)²

Exercise #1

Video Solution

Step-by-Step Solution

Answer

Exercise #2

Video Solution

Step-by-Step Solution

Answer

Exercise #3

Video Solution

Step-by-Step Solution

Answer

Exercise #4

Video Solution

Step-by-Step Solution

Answer

Exercise #5

Video Solution

Step-by-Step Solution

Answer

More Questions

Parabola of the Form y=(x-p)²

Family of Parabolas y=(x-p)²

Family of Parabolas y=(x−p)2y=(x-p)^2y=(x−p)2

Test yourself on parabola of the form y=(x-p)²!

Change in Slope

Graphical and Algebraic Solution when y=0y=0y=0

Graphical Solution

Examples and exercises with solutions from the family of parabolas y=(x-p)²

Exercise #1

Video Solution

Step-by-Step Solution

Answer

Exercise #2

Video Solution

Step-by-Step Solution

Answer

Exercise #3

Video Solution

Step-by-Step Solution

Answer

Exercise #4

Video Solution

Step-by-Step Solution

Answer

Exercise #5

Video Solution

Step-by-Step Solution

Answer

More Questions

Parabola of the Form y=(x-p)²

Family of Parabolas $y=(x-p)^2$

Graphical and Algebraic Solution when $y=0$