In order to convert between fractions and percentages and vice versa, it's important to remember that one percent - .
If you remember this principle, the calculations are simple.
Converting Fractions to Percentages Practice Problems
Master fraction to percentage conversion with step-by-step practice problems. Learn to convert percentages to fractions and vice versa with guided solutions.
- Convert percentages like 25%, 67%, and 225% to simplified fractions
- Transform fractions such as 4/5, 7/20, and 3/4 into percentages
- Master the two-step process for percentage to fraction conversion
- Learn when fractions cannot be converted to percentages without calculators
- Practice reducing fractions to lowest terms after conversion
- Apply conversion skills to mixed numbers and improper fractions
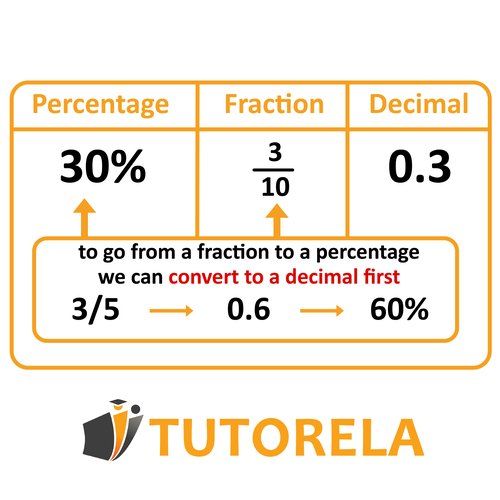
Understanding Converting Fractions to Percentages and Vice Versa
Converting between fractions and percentages and vice versa
Converting Percentages to Fractions
The first stage -
In the numerator, we write the given percentage number (without the percentage sign)
and in the denominator, we always write the number .
The second stage -
We reduce the fraction that we obtained as much as possible in order to achieve the final answer.
Converting Fractions to Percentages
The first stage -
We expand or reduce the fraction so that the number appears in its denominator.
We will make sure to perform the expansion/reduction operation on both the numerator and denominator to maintain the value of the fraction.
The second stage -
What we obtained in the numerator will be the percentage and that will be the final answer.
Important note - not every fraction can be converted to percentages (without a calculator) since not every given denominator can be converted to through expansion or reduction.
Practice Converting Fractions to Percentages and Vice Versa
Write the percentage 89% as a fraction with a denominator of 100.
Examples with solutions for Converting Fractions to Percentages and Vice Versa
Convert the fraction into a percentage.
The fraction:
is actually x percent.
Therefore we use the formula:
Answer:
7%
Convert the fraction into a percentage:
To convert the fraction into a percentage, follow these steps:
- Step 1: Recognize that to convert a fraction to a percentage, you multiply the fraction by 100.
- Step 2: Multiply the numerator of the fraction by 100:
This tells us that is equivalent to 2%.
Therefore, the correct percentage is 2%.
Answer:
2%
Write the percentage 75% as a fraction with a denominator of 100.
To express 75% as a fraction with a denominator of 100, follow these steps:
- Step 1: Recall that a percentage is a fraction out of 100. This means that 75% represents 75 out of 100.
- Step 2: Write the percentage as a fraction: Since 75% means 75 per hundred, this directly gives us the fraction .
Upon checking the given choices, the correct answer is , which matches option 4.
Therefore, the solution to the problem is .
Answer:
Write the percentage 66% as a fraction with a denominator of 100.
To solve the problem of converting 66% into a fraction with a denominator of 100, we follow these simple steps:
- Step 1: Recognize that the percentage 66% means 66 per 100.
- Step 2: Write this as a fraction directly as .
Therefore, the percentage 66% expressed as a fraction with a denominator of 100 is .
Answer:
Write the percentage 54% as a fraction with a denominator of 100.
To solve the problem of converting a percentage to a fraction, follow these steps:
- Step 1: Recognize that the percentage given is 54%.
- Step 2: Use the conversion principle that any percentage can be written as a fraction with a denominator of 100. Thus, 54% is written as .
- Step 3: Check if further simplification is needed. In this case, since we were specifically asked for a denominator of 100, no simplification is required.
Therefore, the percentage 54% as a fraction with a denominator of 100 is .
Answer: