Area of Rectangle Practice Problems & Worksheets Online

Master rectangle area calculations with step-by-step practice problems. Learn base × height formula through interactive exercises and real-world applications.

📚What You'll Practice in These Rectangle Area Exercises
  • Apply the base × height formula to calculate rectangle areas accurately
  • Solve problems with missing dimensions using area and one known side
  • Calculate areas of rectangles in different orientations and positions
  • Find differences between areas of multiple rectangles in complex problems
  • Use Pythagorean theorem to find missing rectangle dimensions in composite figures
  • Work with decimal measurements and mixed units in area calculations

Understanding Area of a Rectangle

Complete explanation with examples

How Do You Calculate the Area of a Rectangle?

Compared to other geometric figures, the rectangle is one of the simplest to work with.

One of the most frequent questions that comes up in exams is related to how to calculate the area of the rectangle.

Before we focus on it, let's do a brief review.

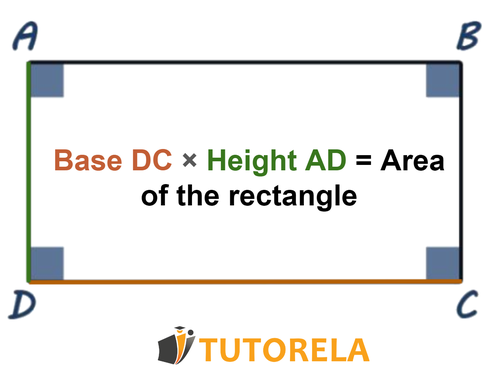
The formula for calculating the area of a rectangle is as follows:

A1 -Base DC x Height AD = Area of the rectangle

Base DC×Height AD=Area of rectangle Base~DC\times Height~AD=Area~of~rectangle

Detailed explanation

Practice Area of a Rectangle

Test your knowledge with 24 quizzes

Given the following rectangle:

666AAABBBDDDCCC3.5

Find the area of the rectangle.

Examples with solutions for Area of a Rectangle

Step-by-step solutions included
Exercise #1

Look at rectangle ABCD below.

Side AB is 10 cm long and side BC is 2.5 cm long.

What is the area of the rectangle?
1010102.52.52.5AAABBBCCCDDD

Step-by-Step Solution

Let's begin by multiplying side AB by side BC

If we insert the known data into the above equation we should obtain the following:

10×2.5=25 10\times2.5=25

Thus the area of rectangle ABCD equals 25.

Answer:

25 cm²

Video Solution
Exercise #2

Given the following rectangle:

111111777AAABBBDDDCCC

Find the area of the rectangle.

Step-by-Step Solution

Let's calculate the area of the rectangle by multiplying the length by the width:

11×7=77 11\times7=77

Answer:

77

Video Solution
Exercise #3

Given the following rectangle:

222555AAABBBDDDCCC

Find the area of the rectangle.

Step-by-Step Solution

Let's calculate the area of the rectangle by multiplying the length by the width:

2×5=10 2\times5=10

Answer:

10

Video Solution
Exercise #4

Given the following rectangle:

888444AAABBBDDDCCC

Find the area of the rectangle.

Step-by-Step Solution

Let's calculate the area of the rectangle by multiplying the length by the width:

4×8=32 4\times8=32

Answer:

32

Video Solution
Exercise #5

Look at the rectangle ABCD below.

Given in cm:

AB = 10

BC = 5

Calculate the area of the rectangle.

101010555AAABBBCCCDDD

Step-by-Step Solution

Let's calculate the area of the rectangle by multiplying the length by the width:

AB×BC=10×5=50 AB\times BC=10\times5=50

Answer:

50

Video Solution

Frequently Asked Questions

Everything you need to know about Area of a Rectangle

What is the formula for calculating the area of a rectangle?

+
The area of a rectangle is calculated by multiplying the base (length) by the height (width): Area = Base × Height. This formula works regardless of the rectangle's orientation or position.

How do you find a missing side of a rectangle when you know the area?

+
Divide the area by the known side length. For example, if the area is 30 cm² and one side is 5 cm, then the other side = 30 ÷ 5 = 6 cm. Use the formula: Unknown side = Area ÷ Known side.

Does the orientation of a rectangle affect its area calculation?

+
No, the orientation does not change the area. Whether a rectangle is horizontal, vertical, or diagonal, the area remains the same as long as the base and height measurements stay constant.

What are common mistakes students make when calculating rectangle area?

+
Common mistakes include: 1) Adding instead of multiplying the sides, 2) Confusing area with perimeter formulas, 3) Not identifying the correct base and height, 4) Forgetting to include square units in the answer.

How is rectangle area different from square area calculation?

+
Rectangle area uses different measurements for length and width (base × height), while square area uses the same measurement for all sides (side × side). Both use multiplication, but rectangles have unequal opposite sides.

Can you calculate rectangle area with decimal measurements?

+
Yes, the same base × height formula applies to decimal measurements. For example, a rectangle with base 2.5 cm and height 10 cm has an area of 2.5 × 10 = 25 cm².

What real-world applications use rectangle area calculations?

+
Rectangle area calculations are used in: flooring and carpeting projects, painting walls, landscaping and gardening, construction and architecture, fabric cutting, and determining land plot sizes.

How do you solve complex rectangle problems with multiple shapes?

+
Break down complex problems into individual rectangles, calculate each area separately, then add or subtract as needed. Always identify what the problem is asking for (total area, difference, or individual areas).

More Area of a Rectangle Questions

Click on any question to see the complete solution with step-by-step explanations

Area of a Rectangle

Rectangle Area: Solving with Width x and Length x/2 When x=4 Calculate Rectangle Area: 3² cm Width × 1.5 cm Length Calculate Rectangle Area with Dimensions 2x and (2x-8): Variable Expression Problem Calculate Rectangle Area: Finding x(x-4) with Variable Dimensions Solve for X: Rectangle with Width 8cm and Area 32cm²

Continue Your Math Journey

Master Area of a Rectangle first, then advance to these related topics that build on your fraction skills

Topics Learned in Later Sections

AreaRectangleAreas of Polygons for 7th GradeHow do we calculate the area of complex shapes?The perimeter of the rectanglePerimeterHow do we calculate the perimeter of polygons?Congruent Rectangles

Practice by Question Type

Choose your preferred learning style and practice format for fraction problems
Applying the formula A shape consisting of several shapes (requiring the same formula) Calculate The Missing Side based on the formula Calculation using the diagonal Extended distributive law Finding Area based off Perimeter and Vice Versa Subtraction or addition to a larger shape Using additional geometric shapes Using Pythagoras' theorem Using ratios for calculation Using short multiplication formulas Using variables Worded problems