Domain - Examples, Exercises and Solutions

Question Types:
Domain: Exercises with fractionsDomain: Identifying and defining elementsDomain: Multiple domainsDomain: Find the domain of an expression with fractions

The transposition of terms involves passing the terms of an equation from one member to another. In fact, it is a group of numbers that, according to mathematical rules, are allowed to be placed in place of the unknown (or variable) within an equation. The concept of transposing terms is especially concerning equations with fractions or square roots in order to find the domain of the equation.

In certain cases we must pay attention to the transposition of terms:

  1. In case of fraction the denominator cannot be equal to zero.
  2. In case of square root, the radicand cannot be negative.

This means that, in such cases, it is not enough to solve the equation, but we must check if the given solution is sustainable or makes sense in the real numbers.

How do you find the domain of an equation with one unknown?
 1. In equations with fractions we will find the domain by equaling the denominator to zero.
The value or values that cause the denominator to equal zero are outside the domain of the equation.

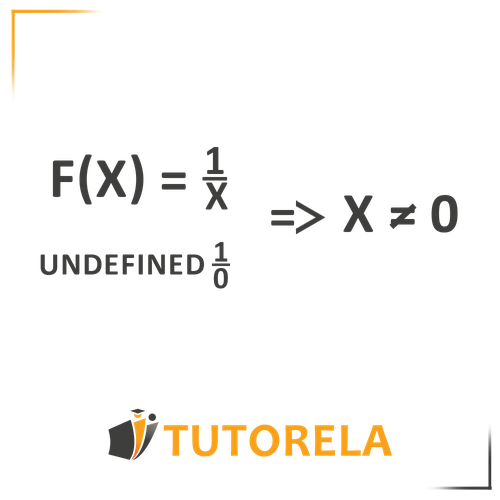
  • In an equation with a root the values that cause the root to be negative are outside the domain of the equation.

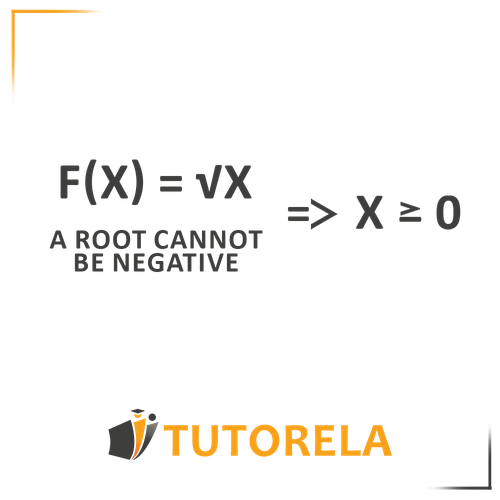
Below we can see an example of how to find the domain of an equation using transpose of terms:

Example :

1(X−2)=1 \frac{1}{(X-2)}=1

This is an equation with fraction in which the unknown appears in the denominator. The denominator cannot be zero, so the expression is not well defined:

0=X−2 0=X-2

Using transposition of terms we can clear the unknown and we obtain:

X=2 X=2

Therefore, the domain of the function is all real numbers except when X=2 X=2 .

Detailed explanation

Suggested Topics to Practice in Advance

  1. Variables in Algebraic Expressions
  2. Equivalent Expressions
  3. Multiplication of Algebraic Expressions
  4. Simplifying Expressions (Collecting Like Terms)
  5. The Numerical Value in Algebraic Expressions

Practice Domain

Question 1

Select the field of application of the following fraction:

\( \frac{6}{x} \)

Question 2

Select the field of application of the following fraction:

\( \frac{8+x}{5} \)

Question 3

Select the field of application of the following fraction:

\( \frac{x}{16} \)

Question 4

\( 2x-3=\frac{4}{x} \)

What is the domain of the exercise?

Question 5

\( 2x+\frac{6}{x}=18 \)

What is the domain of the above equation?

Examples with solutions for Domain

Exercise #1

Select the field of application of the following fraction:

6x \frac{6}{x}

Video Solution

Step-by-Step Solution

Since the domain of definition depends on the denominator, and X appears in the denominator

All numbers will be suitable except for 0.

In other words, the domain of definition:

x≠0 x\ne0

Answer

All numbers except 0

Exercise #2

Select the field of application of the following fraction:

8+x5 \frac{8+x}{5}

Video Solution

Step-by-Step Solution

Since the domain depends on the denominator, we note that there is no variable in the denominator.

Therefore, the domain is all numbers.

Answer

All numbers

Exercise #3

Select the field of application of the following fraction:

x16 \frac{x}{16}

Video Solution

Answer

All X All~X

Exercise #4

2x−3=4x 2x-3=\frac{4}{x}

What is the domain of the exercise?

Video Solution

Answer

x≠0

Exercise #5

2x+6x=18 2x+\frac{6}{x}=18

What is the domain of the above equation?

Video Solution

Answer

x≠0

Question 1

Select the field of application of the following fraction:

\( \frac{x+8}{3x} \)

Question 2

Select the field of application of the following fraction:

\( \frac{3}{x+2} \)

Question 3

Select the field of application of the following fraction:

\( \frac{7}{13+x} \)

Question 4

Select the field of application of the following fraction:

\( \frac{8}{-2+x} \)

Question 5

\( 22(\frac{2}{x}-1)=30 \)

What is the domain of the equation above?

Exercise #6

Select the field of application of the following fraction:

x+83x \frac{x+8}{3x}

Video Solution

Answer

x≠0 x\neq0

Exercise #7

Select the field of application of the following fraction:

3x+2 \frac{3}{x+2}

Video Solution

Answer

x≠−2 x\neq-2

Exercise #8

Select the field of application of the following fraction:

713+x \frac{7}{13+x}

Video Solution

Answer

x≠−13 x\neq-13

Exercise #9

Select the field of application of the following fraction:

8−2+x \frac{8}{-2+x}

Video Solution

Answer

x≠2 x\neq2

Exercise #10

22(2x−1)=30 22(\frac{2}{x}-1)=30

What is the domain of the equation above?

Video Solution

Answer

x≠0

Question 1

\( \frac{6}{x+5}=1 \)

What is the field of application of the equation?

Question 2

What is the domain of the exercise?

\( \frac{5x+8}{2x-6}=30 \)

Question 3

Find the area of domain (no need to solve)

\( 9(4x-\frac{5}{x})=20(3x-\frac{6}{x+1}) \)

Question 4

Find the area of domain (no need to solve)

\( \frac{14}{x}-6x=\frac{2}{x-5} \)

Question 5

Select the field of application of the following fraction:

\( \frac{-8-x}{-x} \)

Exercise #11

6x+5=1 \frac{6}{x+5}=1

What is the field of application of the equation?

Video Solution

Answer

x≠⁡−5 x\operatorname{\ne}-5

Exercise #12

What is the domain of the exercise?

5x+82x−6=30 \frac{5x+8}{2x-6}=30

Video Solution

Answer

x≠3

Exercise #13

Find the area of domain (no need to solve)

9(4x−5x)=20(3x−6x+1) 9(4x-\frac{5}{x})=20(3x-\frac{6}{x+1})

Video Solution

Answer

x≠0,x≠−1 x≠0,x≠-1

Exercise #14

Find the area of domain (no need to solve)

14x−6x=2x−5 \frac{14}{x}-6x=\frac{2}{x-5}

Video Solution

Answer

x≠0,x≠5 x≠0,x≠5

Exercise #15

Select the field of application of the following fraction:

−8−x−x \frac{-8-x}{-x}

Video Solution

Answer

x≠0 x\neq0

Topics learned in later sections

  1. Domain of a Function