Three-Dimensional Figures

Three-dimensional figures

What are three-dimensional figures?

So far we have worked with common two-dimensional figures such as the square or the triangle.
Three-dimensional figures are those that extend into the third dimension, meaning that in addition to length and width, they also have height (that is, the figure has depth).

What differences do three-dimensional figures have?

Three-dimensional figures have several definitions that we will see next:
Below is a three-dimensional figure that we will use to learn each definition - The cube:

Face: it is the flat side of a three-dimensional figure
In the cube we have here, there are 6 faces (one of them is painted gray)
Edge: these are the lines that connect one face to another in a three-dimensional figure
In the cube we have here, there are 12 edges (painted green)
Vertex: it is the point that connects the edges
In the cube we have here, there are 8 vertices (painted orange)

Volume: it is the amount of space contained within a three-dimensional figure.
The units of measurement are $cm^3$ .

Detailed explanation

Start practice

Test yourself on cuboids!

Below is a cuboid with a length of

8 cm.

Its width is 2 cm and its height is

4 cm.

Calculate the volume of the cube.

Practice more now

Cuboid

The cuboid is a three-dimensional figure composed of 6 rectangles.

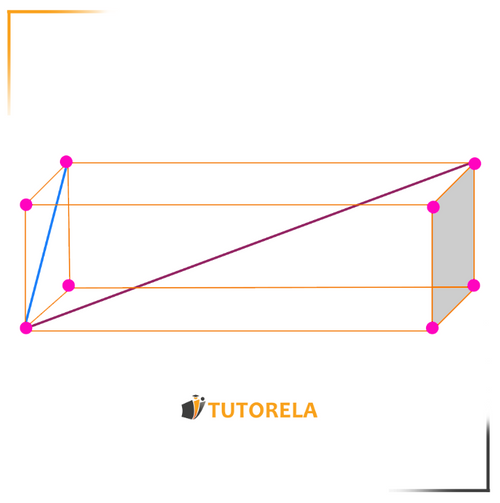
Each cuboid has:

6 faces: the rectangles that make up the cuboid - three pairs of rectangles that can be different from each other.
12 edges: the edges of the cuboid (divided into length, width, and height) - marked in green
8 vertices: the points where the edges meet - marked in orange

You can learn more about the parts of the cuboid by reading this article

Face diagonals:

The diagonals that go from one vertex to another on the same face as long as the vertices belong to the same face - marked in blue

Diagonal of the cuboid:

The diagonals that go from one vertex to another on different faces, as long as the 2 vertices belong to the same face - marked in red

Volume of the cuboid

The volume of the rectangular prism is ( \times \~the~width~\times \~the~length\~the~height\)

For more information about the volume of the rectangular prism click here

Join Over 30,000 Students Excelling in Math!

Endless Practice, Expert Guidance - Elevate Your Math Skills Today

Test your knowledge

Question 1

A cuboid has a length of is 9 cm.

It is 4 cm wide and 5 cm high.

Calculate the volume of the cube.

Question 2

Look at the the cuboid below.

What is its surface area?

Question 3

Look at the cuboid below.

What is its surface area?

Surface area of a cuboid

The formula to find the surface area is(width\times length + height\times width+ height\times length)\times \2

You can read again about the surface area of a rectangular prism by clicking here

Lateral area of a cuboid

It is the sum of the area of the four lateral rectangles (without the bases).
The lateral area of a cuboid can be calculated with the following formula:
a- Length
b- Width
h- Height

2\times \ah+2\times \bh= lateral~area~of~a~cuboid

You can read again about the lateral area of a cuboid by clicking here
For more information about the cuboid click here

Do you know what the answer is?

Question 1

An unfolded cuboid is shown below.

What is the surface area of the cuboid?

Question 2

A cuboid has the dimensions shown in the diagram below.

Which rectangles form the cuboid?

Question 3

Given the cuboid in the drawing, what is the appropriate unfolding?

Cylinder

The cylinder is a three-dimensional figure composed of two identical parallel circles called bases, between which the lateral area expands.

Other properties:

The distance between the two bases is constant and is called the height of the cylinder - we will mark it with an $H$
The radius of both bases is equal, we will mark it with an $R$

Volume of the cylinder

The volume contained within the cylinder is usually denoted by $V$ .
Formula to calculate the volume of the cylinder:
$π\times \R^2\times \H = V$

When:

$π$ = PI ( $3.14$ )
$R$ = Radius of the base
$H$ = Height of the cylinder

For more information about the volume of the cylinder click here

Check your understanding

Question 1

An orthohedron has the dimensions: 4, 7, 10.

How many rectangles is it formed of and what are their dimensions?

Question 2

What are the dimensions of a cuboid composed of two 4X3 rectangles

and of four 4X4 squares?

Question 3

What display can describe an cuboid whose dimensions are 2X3X4?

Total surface area of a cylinder

The total area of the lateral surface and the two bases - we will denote it with $A$
We will use the formula:

$2πR\times \H+2π\times \R^2$

When:

$π$ = PI ( $3.14$ )
$R$ = Radius of the base
$H$ = Height of the cylinder

For more information click here

Lateral area of a cylinder

Only the lateral area without the bases. We will denote it with $S$
We will use the formula:

2πR\times \H

When:

$π$ = PI ( $3.14$ )
$R$ = Radius of the base
$H$ = Height of the cylinder

For more information about the cylinder click here

Do you think you will be able to solve it?

Question 1

Calculate the volume of the cuboid

If its length is equal to 7 cm:

Its width is equal to 3 cm:

Its height is equal to 5 cm:

Question 2

Which dimensions may represent a cuboid?

Question 3

Shown below is a cuboid with a length of 8 cm.

Its width is 2 cm and its height is 4 cm.

Calculate the volume of the cube.

Prism

The right triangular prism is a three-dimensional figure that is composed of 2 triangles and 3 rectangles:

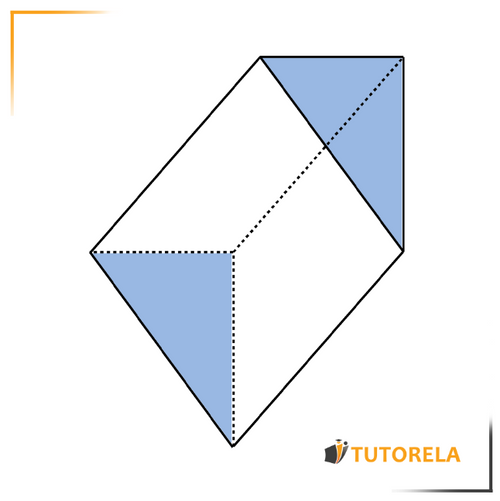
Base of the prism: the 2 triangles that compose it will always be identical (marked in orange).
The triangles can be isosceles, scalene, or equilateral.
To delve deeper into the topic of the bases of the prism click here
Faces of the prism: the 3 rectangles that make up the lateral faces - they will not necessarily be identical.
Heights of the prism: the three lines that join the bases - always have the same length.
For more information about the heights of the prism click here

Let's practice!
In a right triangular prism, are the triangular bases always identical?
Solution:
Yes! The triangles, which are actually the bases, are always the same.
Exercise:
How many heights are there in a right triangular prism?
Are they identical?
Solution:
There are 3 heights in a right triangular prism and they always have the same length.
Exercise:
Do the three rectangles that make up the lateral faces of the prism have to be identical?
Solution:
No.
The edges of the triangle do not necessarily have to be equal and this could create different rectangles.

Volume of the right triangular prism

The volume of the prism is usually expressed through the following formula:
$V= S \cdot H$

$S$ = Area of the base
$H$ = Height of the prism

You can read again about the area of the prism by clicking here

Test your knowledge

Question 1

A cuboid is 9 cm long, 4 cm wide, and 5 cm high.

Calculate the volume of the cube.

Question 2

Look at the cuboid below:

What is the volume of the cuboid?

Question 3

Below is a cuboid with a length of

8 cm.

Its width is 2 cm and its height is

4 cm.

Calculate the volume of the cube.

Area of a right triangular prism

The area of a right triangular prism is, in fact, the total sum of the surfaces of its two bases (the triangles) and its three lateral faces (the rectangles).

For more information about the area of the prism click here

Examples and exercises with solutions of three-dimensional figures

Exercise #1

Look at the the cuboid below.

What is its surface area?

Video Solution

Step-by-Step Solution

First, we recall the formula for the surface area of a cuboid:

(width*length + height*width + height*length) *2

As in the cuboid the opposite faces are equal to each other, the given data is sufficient to arrive at a solution.

We replace the data in the formula:

(8*5+3*5+8*3) *2 =

(40+15+24) *2 =

79*2 =

158

Answer

158

Exercise #2

Look at the cuboid below.

What is its surface area?

Video Solution

Step-by-Step Solution

We identified that the faces are

3*3, 3*11, 11*3
As the opposite faces of an cuboid are equal, we know that for each face we find there is another face, therefore:

3*3, 3*11, 11*3

(3*3, 3*11, 11*3 ) *2

To find the surface area, we will have to add up all these areas, therefore:

(3*3+3*11+11*3 )*2

And this is actually the formula for the surface area!

We calculate:

(9+33+33)*2

(75)*2

150

Answer

150

Exercise #3

An unfolded cuboid is shown below.

What is the surface area of the cuboid?

Video Solution

Step-by-Step Solution

To calculate the surface area of the rectangular prism, we will need to identify its three faces (each face appears twice):

1*3

1*8

3*8

The formula for the surface area of a rectangular prism is the sum of all the areas of the faces, that is:

We replace the data in the formula:

2*(1*3+1*8+3*8)=
2*(3+8+24) =
2*35 =

And this is the solution!

Answer

Exercise #4

A cuboid has the dimensions shown in the diagram below.

Which rectangles form the cuboid?

Video Solution

Step-by-Step Solution

Every cuboid is made up of rectangles. These rectangles are the faces of the cuboid.

As we know that in a rectangle the parallel faces are equal to each other, we can conclude that for each face found there will be two rectangles.

Let's first look at the face painted orange,

It has width and height, 5 and 3, so we already know that they are two rectangles of size 5x6

Now let's look at the side faces, they also have a height of 3, but their width is 6,

And then we understand that there are two more rectangles of 3x6

Now let's look at the top and bottom faces, we see that their dimensions are 5 and 6,

Therefore, there are two more rectangles that are size 5x6

That is, there are
2 rectangles 5X6

2 rectangles 3X5

2 rectangles 6X3

Answer

Two 5X6 rectangles

Two 3X5 rectangles

Two 6X3 rectangles

Exercise #5

Given the cuboid in the drawing, what is the appropriate unfolding?

Step-by-Step Solution

Let's go through the options:

A - In this option, we can see that there are two flaps on the same side.

If we try to turn this net into a box, we'll get a box where on one side there are two faces one on top of the other while the other side is "open",
meaning this net cannot be turned into a complete and full box.

B - This net looks valid at first glance, but we need to verify that it matches the box we want to draw.

In the original box, we see that we have four flaps of size 9*4, and only two flaps of size 4*4,
if we look at the net we can see that the situation is reversed, there are four flaps of size 4*4 and two flaps of size 9*4,
therefore we can conclude that this net is not suitable.

C - This net at first glance looks valid, it has flaps on both sides so it will close into a box.

Additionally, it matches our drawing - it has four flaps of size 9*4 and two flaps of size 4*4.

Therefore, we can conclude that this net is indeed the correct net.

D - In this net we can see that there are two flaps on the same side, therefore this net will not succeed in becoming a box if we try to create it.

Answer

Do you know what the answer is?

Question 1

A cuboid has a length of is 9 cm.

It is 4 cm wide and 5 cm high.

Calculate the volume of the cube.

Question 2

Look at the the cuboid below.

What is its surface area?

Question 3

Look at the cuboid below.

What is its surface area?

Start practice

Three-Dimensional Figures

Three-dimensional figures

What are three-dimensional figures?

What differences do three-dimensional figures have?

Test yourself on cuboids!

Cuboid

Each cuboid has:

Face diagonals:

Diagonal of the cuboid:

Volume of the cuboid

Surface area of a cuboid

Lateral area of a cuboid

Cylinder

Volume of the cylinder

Total surface area of a cylinder

Lateral area of a cylinder

Prism

Volume of the right triangular prism

Area of a right triangular prism

Examples and exercises with solutions of three-dimensional figures

Exercise #1

Video Solution

Step-by-Step Solution

Answer

Exercise #2

Video Solution

Step-by-Step Solution

Answer

Exercise #3

Video Solution

Step-by-Step Solution

Answer

Exercise #4

Video Solution

Step-by-Step Solution

Answer

Exercise #5

Step-by-Step Solution

Answer

More Questions

Cuboids