Perimeter of a Trapezoid Practice Problems with Solutions

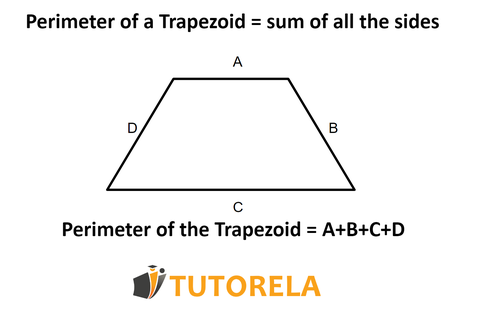
To solve this problem, we'll follow these steps:

• Step 1: Gather the given side lengths of quadrilateral ABCD.

• Step 2: Since it's necessary to understand summation, add all lengths.

• Step 3: Conclude from sum.

Now, let's work through each step:

Step 1: The problem provides:
$\begin{aligned} AB &= 10.5, \\ CD &= 13, \\ AC &= 7.5, \\ BD &= 7.5. \end{aligned}$

Step 2: Add them together:
$\begin{aligned} \text{Perimeter} &= AB + CD + AC + BD \\ &= 10.5 + 13 + 7.5 + 7.5. \end{aligned}$

Step 3: Calculate: $\begin{aligned} \text{Perimeter} &= 10.5 + 13 + 7.5 + 7.5 = 38.5. \end{aligned}$

Therefore, the solution is that the perimeter of quadrilateral ABCD is $38.5$ .

To solve this problem, we'll follow these steps:

• Step 1: Identify the given measurements for the sides.

• Step 2: Use the perimeter formula for a trapezoid, which is summing all sides.

• Step 3: Add the values to get the perimeter.

Now, let's work through each step:

Step 1: The problem gives us four sides to consider. These sides are: $AB = 5$, $CD = 7$, $AC = 4$, and $BD = 4$.

Step 2: The perimeter of a trapezoid or any quadrilateral is simply the sum of all four sides. Hence, we need to add $AB$, $CD$, $AC$, and $BD$.

Step 3: Adding the values, we calculate the perimeter:$AB + CD + AC + BD = 5 + 7 + 4 + 4 = 20$.

Therefore, the perimeter of the given shape is $20$.

In order to calculate the perimeter of the trapezoid we must add together the measurements of all of its sides:

7+10+7+12 =

36

And that's the solution!

To solve this problem, we'll calculate the perimeter of the trapezoid by summing the lengths of all its sides. The steps are as follows:

• List the lengths of the sides: the bases are $10$ and $12$, and the two non-parallel sides are each $7$.
• Apply the perimeter formula for a trapezoid: $P = a + b + c + d$.
• Substitute the given values into the formula: $P = 10 + 12 + 7 + 7$.
• Calculate the sum: $P = 10 + 12 + 7 + 7 = 36$.

Therefore, the perimeter of the trapezoid is $36$.

This matches the correct answer choice from the provided options.

To solve this problem, we'll follow these steps:

• Step 1: Identify all given side lengths of the trapezoid.
• Step 2: Apply the formula for the perimeter of the trapezoid.
• Step 3: Sum up the lengths to find the perimeter.

Now, let's work through each step:
Step 1: The problem gives us the lengths of the trapezoid's sides:
- $AB = 2.5$
- $BC = 10.4$
- $CD = 5.3$
- $DA = 6$

Step 2: We use the formula for the perimeter of a trapezoid:

$P = AB + BC + CD + DA$

Step 3: Plugging in the given values, we calculate:

$P = 2.5 + 10.4 + 5.3 + 6$

Calculating further, we have:

$P = 24.2$

Therefore, the perimeter of the trapezoid is $24.2$.