The intervals of increase and decrease describe the in which the parabola goes up and those in which it goes down.
Let's see it in an illustration:
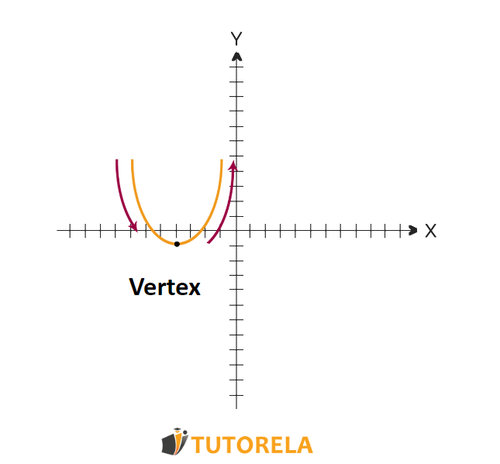
We must always observe the function from left to right.
When we see a negative slope (this is how decrease looks) – the function is decreasing.
When we see a positive slope (this is how increase looks) – the function is increasing.
The parabola will change interval only at one point - at the vertex of the parabola.
To examine the intervals of increase and decrease of the function, we can observe in the illustration
What happens when the x's are smaller than the of the vertex and what happens when the x's are larger than the of the vertex?
What are the intervals of increase and decrease in this example?
We can see that the of the vertex is .
When the function is increasing and, therefore, there is an interval of increase.
When the function is decreasing and, therefore, there is an interval of decrease.
What happens when there is no illustration?
We can examine the equation of the function and determine based on the coefficient of whether it is a function with a minimum or maximum point.
When the coefficient is positive (happy face) - minimum
When the coefficient is negative (sad face) - maximum
Now, let's find the of the vertex according to the formula or symmetric points.
You can read more about how to find the vertex of the parabola here.
And that's it! We have all the information to determine when it is an increasing function and when it is decreasing - the vertex of the parabola is the highest or lowest point according to the concerning parabola.
All that remains for us to do is, draw a sketch to see in it the intervals of increase and decrease clearly.
Let's see an example:
When we see that the of the vertex is
and we conclude that the parabola has a happy face, we will make a small drawing:
It can be clearly seen that the function is increasing when and decreasing when , therefore:
Interval of increase:
Interval of decrease:
