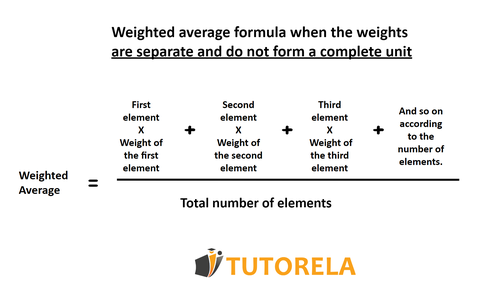
A weighted average is a type of average where each number has a different level of importance, called its weight. This means that some numbers affect the final result more than others.
To better understand the idea of weight, you can think of it as importance. Some values are more important, and others are less important—not because they are bigger or smaller, but because they have more influence on the result.
When a number is more important, it has a greater weight, and therefore it will have a stronger effect on the weighted average.