To convert a decimal fraction to a mixed fraction,
we ask ourselves how to read the decimal fraction or in other words, what the last digit represents –
if we use the word tenths – we place 10 in the denominator
if we use the word hundredths – we place 100 in the denominator
if we use the word thousandths – we place 1000 in the denominator
Converting a Decimal Fraction to a Mixed Number
Converting a decimal to a mixed number
The number itself – everything that appears after the decimal point, we place in the numerator.
The whole number in the decimal fraction, we add to the mixed fraction as the whole number in the mixed fraction.
Test yourself on converting decimal fractions to simple fractions and mixed numbers!
Write the following fraction as a decimal:
\( \frac{5}{100}= \)
Converting a decimal fraction to a mixed number
The transition from a decimal fraction to a mixed number is simple and easy if you just know the right way.
To do it correctly without making mistakes, we recommend that you make sure you know how to read decimal fractions properly.
If you know how to read decimal fractions correctly – the path to success in transitioning from a decimal fraction to a simple fraction is completely paved for you.
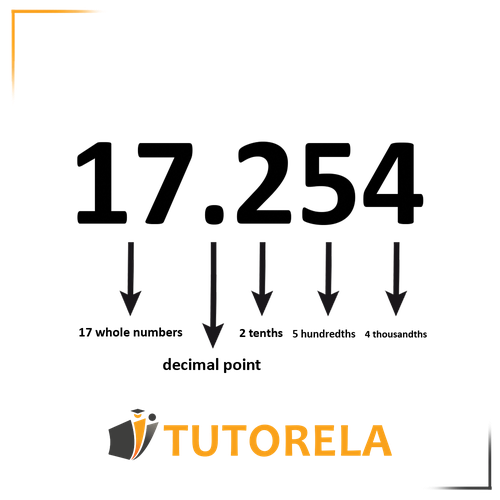
How do you read a decimal fraction?
A decimal fraction represents a fraction or a non-whole number using a decimal point.
The decimal point divides the fraction as follows:

Explanation:
The entire part to the left of the decimal point is called whole numbers.
The entire part to the right of the decimal point is divided as follows:
The first digit after the point represents tenths
The second digit after the point represents hundredths
The third digit after the point represents thousandths
Remember! There is no unity – the counting starts from tenths.
How do we know the denominator of the mixed fraction?
As we saw above, decimal fractions consist of whole numbers that are before the decimal point and parts that are after the decimal point, where the parts are made up of tenths, hundredths, and thousandths.
If we convert the tenths, hundredths, and thousandths to a denominator in a simple fraction, we get:
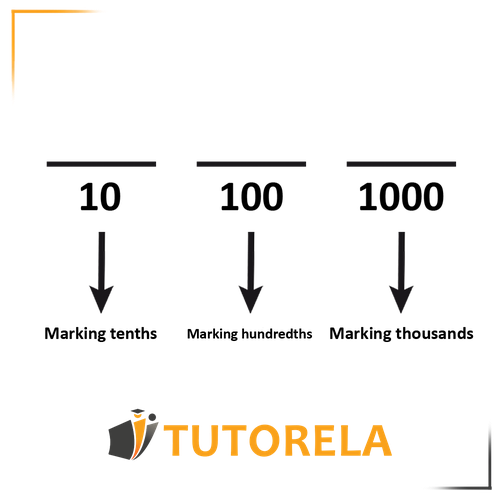
Remember:
Thousandths – 1000 in the denominator
Hundredths – 100 in the denominator
Tenths – 10 in the denominator
How will you remember this?
It is very easy and simple.
Tenths come from the word 10, so the denominator that should appear is 10.
Hundredths come from the word 100, so the denominator that should appear is 100.
Thousandths come from the word 1000, so the denominator that should appear is 1000.
The key to success lies here:
To read a decimal fraction correctly, we need to ask ourselves what the last digit in the decimal fraction represents.
For example:
How do we read the decimal ?
6 is the last digit and it represents hundredths, so we read the decimal as whole and hundredths.
How wonderful! We just learned the notation for hundredths -

All that remains for us is to put in the numerator, in the whole number part and get the simple fraction of the decimal :
Let's practice more:
Convert the decimal to a mixed number
Solution:
Let's ask ourselves – how do we read the fraction?
wholes and tenths.
Therefore, we will use the tenths notation (denominator ) and place in the numerator and in the wholes:
Convert the decimal fraction to a simple fraction
Solution:
Let's ask ourselves – how do we read the fraction?
wholes and thousandths.
Therefore, we will use the thousandths notation (denominator ), place in the numerator and as the whole number:
Note – if the fraction can be simplified, you can simplify it without changing its value:
And indeed we already know that:
Convert the decimal to a simple fraction
Solution:
Let's ask ourselves – how do we read the fraction?
whole and hundredths
Therefore, we will use the hundredths notation – denominator , place in the numerator and as the whole number.
We get:
We can simplify by dividing by and get:
Another example
Convert the decimal to a mixed number
Solution:
Let's ask ourselves – how do we read the fraction?
wholes and hundredths
Therefore, we will use the hundredths notation - denominator and place in the numerator. Let's not forget to add wholes on the side and we get:
Write the following fraction as a decimal:
\( \frac{3}{100}= \)
Write the following fraction as a decimal:
\( \frac{3}{100}= \)
Write the following fraction as a decimal:
\( \frac{11}{100}= \)
Examples with solutions for Converting Decimal Fractions to Simple Fractions and Mixed Numbers
Exercise #1
How much of the whole does the shaded area (blue) represent?
Step-by-Step Solution
To solve this problem, we need to determine the fraction of the whole grid that is represented by the shaded (blue) area. The grid is a 10x10 layout, therefore containing a total of equal-sized squares.
Step 1: We count the number of shaded squares in the grid. According to the illustration, there are 86 shaded squares.
Step 2: Calculate the fraction of the shaded area compared to the whole grid: .
Step 3: Convert this fraction into a decimal. Dividing the numerator by the denominator gives us .
Therefore, the shaded area represents of the total grid, which is equivalent to .
This matches with the correct answer choice, which is: or .
Answer
or
Exercise #2
How much of the whole does the shaded area (blue) represent?
Step-by-Step Solution
To solve this problem, we will determine how much of the whole grid is represented by the shaded area.
The problem provides a 10x10 grid which contains 100 smaller squares in total. Our task is to determine how many of these squares are shaded.
Upon inspection, we count that 80 out of the 100 squares are shaded.
Therefore, the fraction of the whole that the shaded area represents is given by dividing the number of shaded squares by the total number of squares:
Converting this fraction to a decimal gives .
Thus, the shaded area represents or of the whole.
Among the choices provided, the correct answer is: or .
Answer
or
Exercise #3
Convert into fraction form:
Video Solution
Step-by-Step Solution
Let's pay attention to where the decimal point is located in the number.
Remember:
One number after the zero represents tens
Two numbers after the zero represent hundreds
Three numbers after the zero represent thousands
And so on
In this case, there are two numbers after the zero, so the number is divided by 100
Let's write the fraction in the following way:
We'll then remove the unnecessary zeros as follows:
Answer
Exercise #4
Convert into fraction form:
Video Solution
Step-by-Step Solution
Let's pay attention to where the decimal point is located in the number.
Remember:
One number after the zero represents tens
Two numbers after the zero represent hundreds
Three numbers after the zero represent thousands
And so on
In this case, there are two numbers after the zero, so the number is divided by 100
We'll write the fraction like this:
We'll then remove the unnecessary zeros as follows:
Answer
Exercise #5
Convert into fraction form:
Video Solution
Step-by-Step Solution
Let's pay attention to where the decimal point is located in the number.
Remember:
One number after the zero represents tens
Two numbers after the zero represent hundreds
Three numbers after the zero represent thousands
And so on
In this case, there are two numbers after the zero, so the number is divided by 100
We'll write the fraction in the following way:
We'll then proceed to remove the unnecessary zeros as follows:









