In this article, we will study the second criterion of congruence:
Congruence Criterion: Angle, Side, Angle
Angle, Side, Angle
Definition:
Two triangles in which two angles and the included side between them are equal are congruent triangles.
Attention: The two angles must be adjacent to the equal and corresponding side in both triangles!
To prove that two triangles are congruent we can use one of the following postulates:
Example 1 - Congruent Triangles
Given the triangles and such that:

From this, we deduce that the triangles and are congruent, therefore, we will write:
according to the congruence criterion: Angle, Side, Angle (ASA)
Consequently, we will deduce that:
since these are corresponding and equal sides in congruent triangles.
Then, we will also deduce that:
\\sphericalangle C=\sphericalangle F\)
since these are corresponding and equal angles in congruent triangles.
Example 2 - Triangle Congruence Exercise
Given two parallel lines. The line and the line pass between them in such a way that they intersect at point . Also, we are informed that
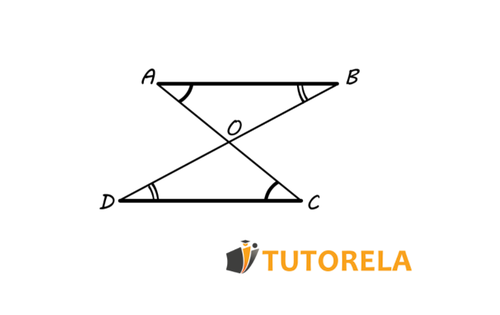
Prove that
Proof:
First, we must show that triangles and are congruent. We will base this on the previous criterion.
Note that (Because they are vertical angles)
Since (Side)
Remember that the two given lines are parallel lines.
Therefore, since they are alternate interior angles between parallel lines (angle).
We observe that now we have triangles in which of their angles and the side included between them are equal.
Consequently, triangles and are congruent
and we write it as according to the Angle, Side, Angle (ASA) congruence criterion
Therefore, we can deduce that (Corresponding sides of congruent triangles).
Angle-Side-Angle Congruence Exercises
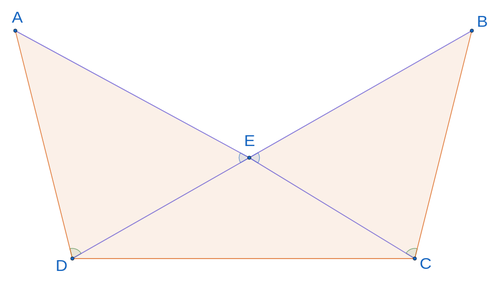
Exercise 1
Assignment
Given that point bisects .
and also
According to which theorem of congruence do the triangles coincide?

Solution
Given that the angles
Given that point cuts
Angles
Opposite angles by the vertex are equal
The triangles are congruent according to the theorem
Answer
Exercise 2
Assignment
Given: the quadrilateral is a rectangle.
By which theorem of congruence do the triangles coincide?
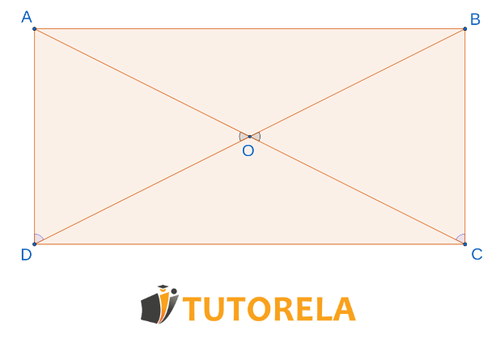
Solution
Since the quadrilateral is a rectangle and in a rectangle there are two pairs of parallel and equal sides
Angles are alternate angles between equal parallel lines.
Angles
opposite angles at the vertex are equal
Therefore, we say that the triangles are congruent according to the theorem
Answer
According to the theorem
Exercise 3
Assignment
In the given figure:
and point bisects segment .
According to which theorem of congruence do the triangles match?
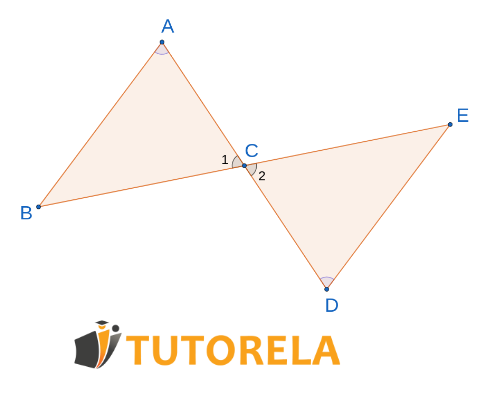
Solution
Given that is parallel to
Angles
Alternate angles are equal between parallel lines
Point bisects the line
Angles
Vertex opposite angles
Triangles congruent according to the superposition theorem
Answer
Superimposed according to
Exercise 4
Assignment
Given the isosceles triangle .
According to which theorem of congruence do the triangles coincide?
Solution
Triangle is an isosceles triangle
In an isosceles triangle, two sides are equal
Angles given
angles the base angles of an isosceles triangle are equal
angles
Subtraction of angles
Triangles are congruent according to the theorem
Answer
Exercise 5
Assignment
Given: quadrilateral square.
And within it is enclosed the kite .
According to which theorem of congruence do the triangles coincide?
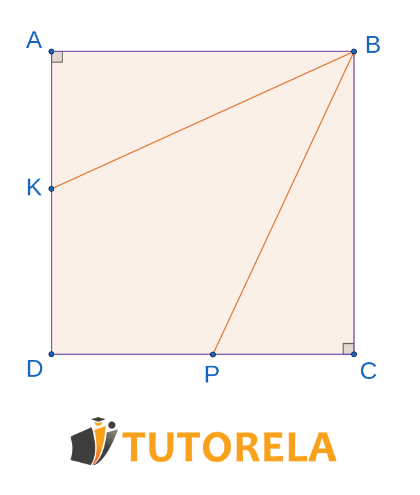
Solution
is a square
In a square all sides are equal
Given that is a kite
In a kite two pairs of adjacent sides are equal
Equal angles in a square are degrees
The angles of the kite are equal
therefore
if two angles are equal then the third is also equal
The triangles are congruent according to
Answer
Review Questions
What is a criterion in geometry?
In mathematics, a criterion is a guideline that allows us to determine certain characteristics depending on the case or topic being studied. In the case of geometry, it allows us to judge certain characteristics for given figures.
What is the triangle congruence criterion?
There are triangle congruence criteria, which help us determine when two triangles are congruent, that is, they help us determine when two triangles have the same dimensions and corresponding angles, thus having the same shape and side measurements regardless of the orientation of these triangles.
What is the ASA criterion?
We say that two triangles are congruent with the ASA criterion when two of their angles and a non-included side are congruent.
What is the angle-side-angle criterion?
This criterion tells us that two triangles are congruent when two angles and the side between them are congruent.
How do you determine the criterion of a triangle?
This depends on the corresponding angles and sides of both triangles.
For example, given two triangles:
If we have three corresponding sides of two triangles that are congruent, then we are talking about the SSS criterion.
If it is found that the two angles and the included side between the corresponding angles are congruent, we refer to the ASA criterion.
When observing that two of their angles and the non-included side are congruent respectively, then we are discussing the AAS criterion.
And finally, when two pairs of corresponding sides and the included angle between these sides are congruent, it will be the SAS criterion.
Based on this, we can determine which congruence criterion we are referring to and deduce whether or not they are congruent triangles.
- Congruent Triangles
- Congruence Criterion: Side, Angle, Side
- Congruence Criterion: Side, Side, Side
- Side, Side, Angle
- Congruence of Right Triangles (using the Pythagorean Theorem)
- The Pythagorean Theorem
- Congruent Rectangles
- Similarity of Triangles and Polygons
- Similarity of Geometric Figures
- Similarity ratio
- Similar Triangles
- Triangle similarity criteria
- Sum of Angles in a Polygon
- Sum of the Interior Angles of a Polygon
- Exterior angles of a triangle
- Sum of the Exterior Angles of a Polygon
- Relationships Between Angles and Sides of the Triangle
- Relations Between The Sides of a Triangle








