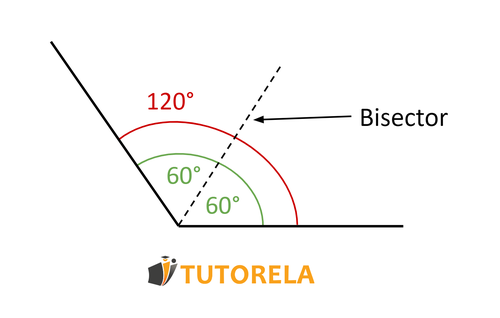
A bisector is a line segment that passes through the vertex of an angle and divides it into two equal angles.
The bisector can appear in a triangle, parallelogram, rhombus and in other geometric figures.
For example, a bisector that passes through an angle of degrees will create two angles of degrees each.
a is a bisector.
\( ∢BAC = 80° \)
Calculate angle \( \alpha \).
Calculate angle given that it is a bisector.
Since an angle bisector divides the angle into two equal angles, and we are given that one angle is equal to 60 degrees. Angle is also equal to 60 degrees
Answer:
60
BD is a bisector.
What is the size of angle ABC?
Since we are given that the value of angle DBC is 65 degrees, and we know that the angle bisector divides angle ABC into two equal angles, we can calculate the value of angle ABC:
Answer:
130
Which of the following figures has a bisector?
The answer is C because the angle bisector divides the angle into two equal angles. In diagram C, the angle bisector divides the right angle, which is equal to 90 degrees, into 2 angles that are equal to each other.
Answer:
ABCD is a square.
Due to the fact that all angles in a square are equal to 90 degrees, and BC bisects an angle, we can calculate angle ABC accordingly:
Answer:
45
ABCD is a deltoid.
As we know that ABCD is a deltoid, and AC is the bisector of an angle and therefore:
Now we focus on the triangle BAD and calculate the sum of the angles since we know that the sum of the angles in a triangle is 180 degrees:
We divide the two sections by 6:
Now we can calculate the angle DAC:
Answer:
30