Equation (+ what is the unknown) - Examples, Exercises and Solutions

To solve the equation $5x = 0$ for $x$, we will use the following steps:

• Step 1: Identify that the equation is $5x = 0$.
• Step 2: To solve for $x$, divide both sides of the equation by 5.

Let's perform the calculation as outlined in Step 2:

$5x = 0$

Divide both sides by 5 to isolate $x$:

Simplifying, this gives:

$x = 0$

Therefore, the solution to the equation $5x = 0$ is $x = 0$.

The correct answer is option 4: $x = 0$.

To solve the equation $5x = 1$, we need to isolate $x$. Here are the steps:

• Step 1: Start with the equation $5x = 1$.
• Step 2: Divide both sides of the equation by the coefficient of $x$, which is 5, to isolate $x$. This gives us:
$\frac{5x}{5} = \frac{1}{5}$
• Step 3: Simplify the left side:
$\frac{5x}{5} = x$
• Step 4: Write the simplified equation:
$x = \frac{1}{5}$

Therefore, the solution to the equation $5x = 1$ is $x = \frac{1}{5}$.

The correct answer choice is:

$x = \frac{1}{5}$

To solve the equation $14x + 3 = 17$, we need to find the value of $x$ that satisfies the equation.

Step 1: Isolate the term containing $x$ by subtracting 3 from both sides of the equation:

$14x + 3 - 3 = 17 - 3$
This simplifies to:
$14x = 14$

Step 2: Solve for $x$ by dividing both sides by 14:

$x = \frac{14}{14}$
Which simplifies to:
$x = 1$

Therefore, the solution to the equation $14x + 3 = 17$ is $x = 1$.

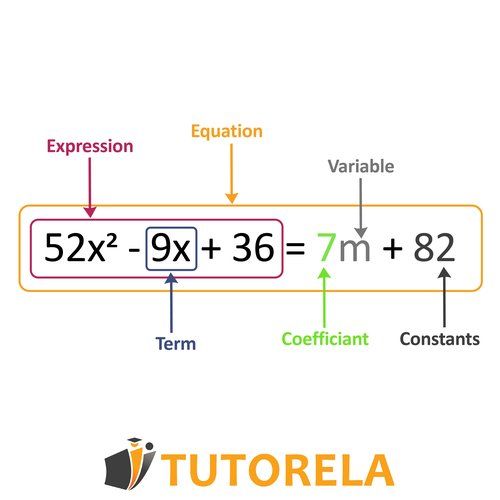
In order to solve this exercise, we first need to identify that we have an equation with an unknown.

To solve such equations, the first step will be to arrange the equation so that on one side we have the numbers and on the other side the unknowns.

$2X+7-5X-12=-8X+3$

First, we'll move all unknowns to one side.
It's important to remember that when moving terms, the sign of the number changes (from negative to positive or vice versa).

$2X+7-5X-12+8X=3$

Now we'll do the same thing with the regular numbers.

$2X-5X+8X=3-7+12$

In the next step, we'll calculate the numbers according to the addition and subtraction signs.

$2X-5X=-3X$
$-3X+8X=5X$

$3-7=-4$$$
$-4+12=8$

$5X=8$

At this stage, we want to reach a state where we have only one $X$, not $5X$,
Thus we'll divide both sides of the equation by the coefficient of the unknown (in this case - 5).

$X={8\over5}$