Vertical Multiplication - Examples, Exercises and Solutions

Vertical multiplication is a method used to multiply numbers by aligning them vertically, with one number on top of the other. This layout makes it easier to multiply digits step by step, especially when dealing with multi-digit numbers.

Solving Vertical Multiplication is easy when following these steps:

1. Write the Numbers Vertically:
Place the larger number on top and the smaller number below it, aligning the digits by their place values.

2. Multiply Each Digit:
Start by multiplying the bottom number’s rightmost digit (ones place) with each digit of the top number. Write the results below, ensuring they are aligned properly.

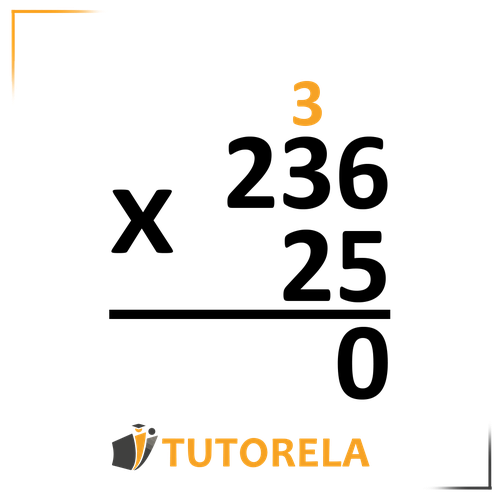
3. Add the Carry:
If the product of two digits exceeds 9, write down the ones place and carry the tens place to the next digit.

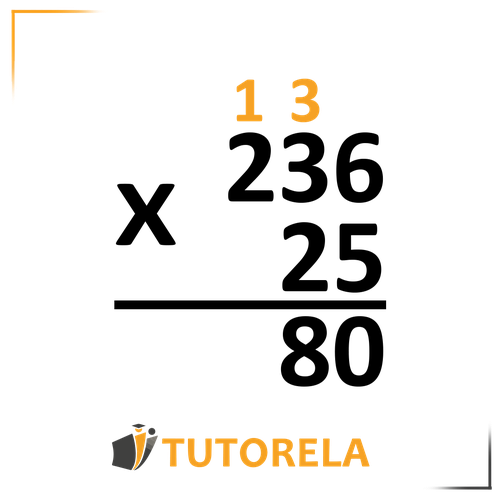
4. Shift for Place Value:
When moving to the next digit of the bottom number, shift the results one place to the left (to account for place value).

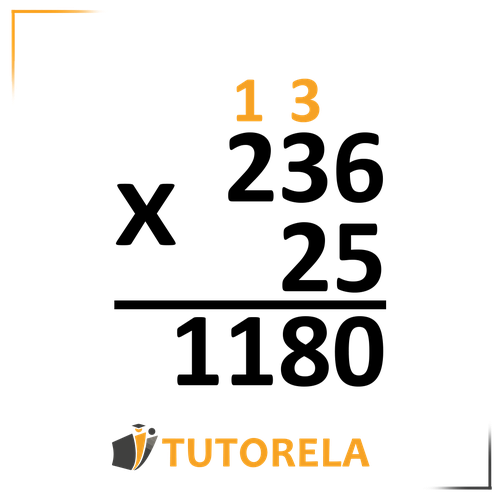
5. Add the Results:
After multiplying with all digits of the bottom number, add the rows of partial products to find the final result.

Learn the multiplication tables thoroughly and follow these rules:

First rule

Write down the exercise correctly:
The ones under the ones, the tens under the tens, and the hundreds under the hundreds.
The number with more digits will be written above the one with fewer digits.

Second rule

When the product is greater than $9$ it is stored at the top left and must be remembered to add it to the next result.

Third rule

Before moving on to multiply the next digit, the "numbers stored" at the top left must be erased to avoid confusion.

Fourth rule

We will add a $0$ below the result to indicate that we have moved to the next digit, each row of results will start one place to the left in relation to the previous row.