Simplifying roots is a way to simplify a root and solve it easily without a calculator.
Simplifying Roots
Simplifying Roots
Steps for solving:
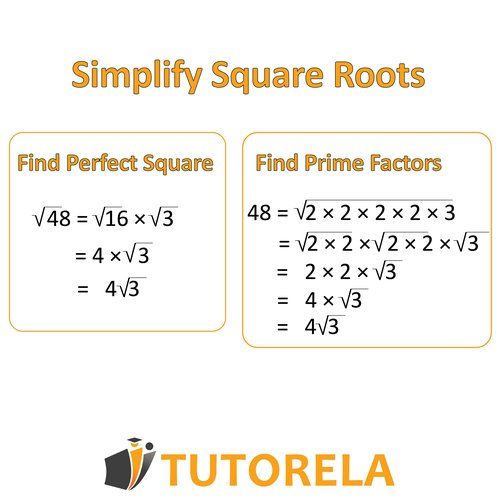
1. Observe the number inside the root and factor it out. Always think about the highest factor that we can take a square root of.
2. The factors that cannot be square rooted will remain as they are.
Test yourself on simplifying square roots!
Which of the following clauses is equal to 100?
Simplifying Roots
What is a root?
A square root of any number, for example √x, is actually a number that when multiplied by itself gives us .
For example:
Therefore:
Another example:
What will happen if we're asked what is the square root of for example:
This is exactly where the square root simplification technique comes in handy!
Simplifying Roots
This technique simplifies the number inside the root by factoring it, making it much easier for us to find the solution!
We can express like this:
And actually write
We can write this exercise like this according to the laws of exponents:
Great! We know what the square root of is, so we can write it in this form:
Let's summarize the solution steps:
- Check if the number under the root can be broken down into simple factors that can easily be square rooted.
- For numbers that can be square rooted, we extract the root, and for those that cannot, we leave them with the root.
- Verify that there are only multiplication operations between the factors.
Tip!
How do we know which factors are best to break down the number inside the square root?
We will always look for the largest factor that has a whole square root!
For example:
Let's think about which is the largest factor we can take out that has a square root?
The answer is . If we take out the factor we obtain the following:
We can then proceed to write that:
If we were to factor out numbers like and or and we wouldn't be able to progress with solving the exercise.
Now let's move on to more complex exercises!
Here is the exercise
Don't be intimidated by its scary appearance! Just imagine a multiplication operation between and the square root sign, and continue to factor the number as you learned before.
The largest number in that we can find a square root for is .
Therefore we'll write the following:
We know that hence we can substitute for and obtain:
Another exercise:
Think about the largest factor that can be extracted from and apply a square root to it..
The answer is of course !
Therefore we can write
Pay attention!!
We know that is therefore we can write the exercise like this:
But what about ?
We can also factor !
The largest factor we can take out of and apply a square root to is .
So the two factors we'll take out are 2 and 4.
We observe that:
We know that
Therefore, we'll insert the data into the exercise and obtain:
Keep practicing and make the exam easier for yourself!
Note- we won't always rush to find and extract factors on autopilot. We know that
therefore this exercise, for example, is really quite simple. We obtain the following solution:
Another exercise:
Let's identify the largest factor we can take out of that has a whole square root?
The answer is that there isn't one. The factors of are only and
Therefore we cannot simplify this square root.
What about this exercise?
With this large number, we'll work in steps.
Let's look at . From the number we can extract hence we'll write as follows:
From we can extract multiples of and
We obtain the following solution as seen below:
Now we'll solve what we can and get:
Which of the following clauses is equal to 100?
Examples with solutions for Simplifying square roots
Exercise #1
Which of the following clauses is equal to 100?
Video Solution
Step-by-Step Solution
To determine which expression equals 100, we need to evaluate each option:
- Option 1:
- Calculate .
- Then compute . - Option 2:
- Calculate .
- Then compute . - Option 3:
- Calculate , which simplified through breakdown is larger than 100 because . Hence this 25 to the power of 4 will definitely be much larger than 100. - Option 4:
- Calculate .
- Calculate .
- Compute .
Therefore, the expression in Option 4, , equals 100. Thus, the correct choice is 4.
Thus, the clause that equals 100 is .









