Look at the parallelogram ABCD below. A A A B B B D D D C C C What can be said about triangles ACD and ABD?

They are equal in area and perimeter.

In the image there are a pair of similar triangles and a triangle that is not similar to the others. Determine which are similar and calculate their similarity ratio. 8 8 8 4 4 4 6 6 6 9 9 9 3 3 3 6 6 6 3 3 3 1 1 1 2 2 2 A A A B B B C C C G G G H H H I I I D D D E E E F F F A B C

$$ a $$ and $$ b $$, similarity ratio of $$ 3 $$

$$ a $$ and $$ c $$, similarity ratio of $$ 2 $$

$$ b $$ and $$ c $$, similarity ratio of $$ 1.5 $$

$$ b $$ and $$ c $$, similarity ratio of $$ 2 $$

Similarity Theorems - Examples, Exercises and Solutions

Understanding Similarity Theorems

Complete explanation with examples

Conditions for the similarity between two triangles

To demonstrate the similarity between triangles it is not necessary to show again and again the relationship between the three pairs of sides and the equivalence between all the corresponding angles. This would require too much unnecessary work.

There are three criteria by which we can see the similarity between triangles:

Conditions for the similarity between two triangles

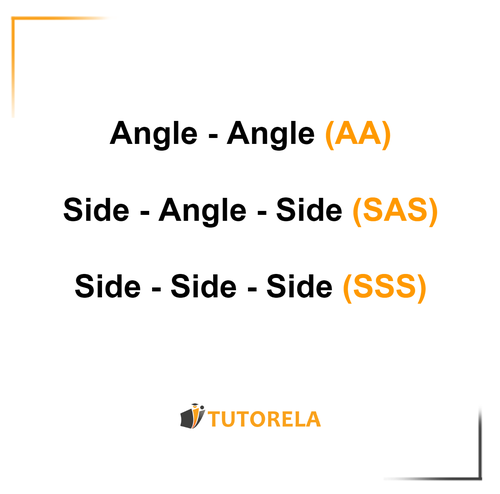
Angle - Angle (AA): two triangles are similar if they have two equal angles.
Side - Angle - Side (SAS): Two triangles are similar if the ratio between two pairs of sides and also the angle they form are equal.
Side - Side - Side (SSS): Two triangles are similar if the ratio between all their sides (similarity ratio) is equal in both triangles.

Detailed explanation

Practice Similarity Theorems

Test your knowledge with 14 quizzes

Look at the two triangles below:

Angle B is equal to angle E.

Angle C is equal to angle F.

Which side corresponds to side AC?

Examples with solutions for Similarity Theorems

Step-by-step solutions included

Exercise #1

Look at the following two triangles:

Angles B and D are equal.
Angles A and F are equal.

Which side corresponds to AB?

Step-by-Step Solution

As we have two equal angles, we will use the angle-angle theorem to simulate triangles.

We will compare the vertices: $A=F,B=D$

According to the data it seems that:

Side AC corresponds to side EF.

Side BC corresponds to side DE.

Therefore, side AB corresponds to side FD.

Answer:

$FD$

Video Solution

Exercise #2

Look at the two triangles below:

Angle B is equal to angle E.
Angle A is equal to angle D.

Which angle corresponds to angle C?

Step-by-Step Solution

As we have two pairs of corresponding angles, we will use the angle-angle theorem for triangle similarity.

Now that we know all angles are equal to each other, we note that the remaining angle that is equal and corresponds to angle C is angle F.

Answer:

$F$

Video Solution

Exercise #3

Angle B is equal to 40°

Angle C is equal to 60°

Angle E is equal to 40°

Angle F is equal to 60°

Are the triangles similar?

Step-by-Step Solution

Given that the data shows that there are two pairs with equal angles:

$B=E=40$

$C=F=60$

The triangles are similar according to the angle-angle theorem, therefore triangle ABC is similar to triangle DEF.

Answer:

Yes

Video Solution

Exercise #4

Angle B is equal to 70 degrees

Angle C is equal to 35 degrees

Angle E is equal to 70 degrees

Angle F is equal to 35 degrees

Are the triangles similar?

Step-by-Step Solution

The triangles are similar according to the angle-angle theorem.

Having two pairs of equal angles is sufficient to conclude that the triangles are similar.

Answer:

Yes

Video Solution

Exercise #5

Look at the two triangles below:

Angle B is equal to angle F.

Angle C is equal to angle D.

Which angle corresponds to angle A?

Step-by-Step Solution

We use the angle-angle theorem to simulate triangles.

Let's observe the data we already have:

Angles B and F are equal.

Angle C is equal to angle D.

Therefore, the remaining angles must also be equal: angles A and E.

Answer:

$E$

Video Solution

Continue Your Math Journey

Master Similarity Theorems first, then advance to these related topics that build on your fraction skills

Topics Learned in Later Sections

Practice by Question Type

Choose your preferred learning style and practice format for fraction problems

Identifying and defining elements Applying the formula Identifying and defining elements

Similarity Theorems - Examples, Exercises and Solutions

Understanding Similarity Theorems

Conditions for the similarity between two triangles

Practice Similarity Theorems

Examples with solutions for Similarity Theorems

Continue Your Math Journey

Suggested Topics to Practice in Advance

Topics Learned in Later Sections

Practice by Question Type