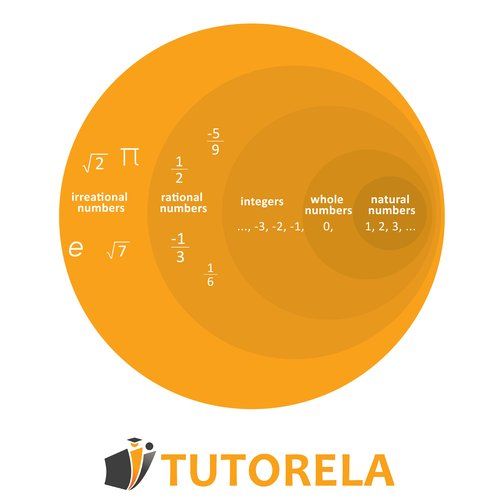
Rational numbers are those numbers that can be represented as a fraction - numerator and denominator.
- Every whole number is a rational number
- Decimal numbers are also rational numbers
- Rational numbers can also be negative
Rational Numbers
Rational Numbers
What is a rational number?
Examples of rational numbers
Rational Numbers
In this article, you will learn everything you need to know about rational numbers and you will practice it in various exercises.
Shall we start?
What is a rational number?
Rational numbers are those numbers that can be represented as the quotient of integers - numerator and denominator.
In other words – A rational number is a fraction.
Observe: Every rational number can be represented as a fraction in several ways.
For example, the rational number can be written like this: or like this or, even, like this:
Every integer is a rational number since we can write it in fraction form with the denominator .
For example, the integer , can be written as a rational number in the following way: .
And what about the ?
The can be represented as a rational number in this way: .
Rational numbers can be negative and also decimals.
And now what do we do? Let's practice!
Determine which numbers are rational within the following set
Solution:
All numbers are rational. They can be written as a quotient in the following way:
: represented as a fraction
: represented as a fraction
: represented as a fraction
: represented as a fraction
Determine which numbers are rational within the following set
Solution:
All the numbers are rational. They can be written as a quotient in the following way:
: represented as a fraction
: represented as a fraction
: Rational number represented as a fraction with numerator and denominator
: Rational number represented as a fraction with numerator and denominator.
Determine which numbers are rational within the following set
Solution:
All numbers are rational. They can be written as a quotient in the following way:
: Rational number, can be represented as a fraction
: Rational number, can be represented as a fraction
: Rational number, can be represented as a fraction
: Rational number, can be represented as a fraction with only numerator and denominator in the following way:
Now that we know how to identify rational numbers, we will move on to the corresponding exercises.
Find the rational numbers that are equivalent to the given rational number
Solution:
We need to find rational numbers, that is, fractions, that are equivalent to the rational number
By expanding the fraction, we can arrive at fractions that express the same value.
Let's expand by . Remember that we must expand both the numerator and the denominator so as not to change the value of the fraction.
We will obtain:
Now let's expand the rational number by .
We will find that:
We have to find more fraction, that is equivalent to the given fraction.
This time we will expand by .
Observe, we can expand the fraction as much as we want. As long as the same operation is performed on both the numerator and the denominator, the value of the fraction will remain unchanged.
We will expand by and we will obtain:
And, in this way, we can conclude the exercise:
Another exercise with rational numbers
Complete the figures in rational numbers to obtain any true statement.
Solution:
This exercise might seem a bit complicated at first, but, if we understand the trick, we will solve it very easily.
One of the methods to match fractions is by applying a common denominator.
When both fractions have a common denominator, it is very easy for us to realize which one of them is larger and which is smaller according to the numerator.
In this exercise, we are looking for a rational number with denominator that is greater than .
are, in fact, \frac{6/9} and, therefore, \frac{7/9} for example, will be greater than \frac{2/3}
Additionally, we are looking for a rational number with denominator that is less than \frac{2/3}
\frac{2/3} are, in fact, \frac{4/6} and, therefore, \frac{3/6} for example, will be less than \frac{2/3}
We will obtain:
\frac{3/6}<\frac{2/3}<\frac{7/9}








