Real Numbers

In this article, you will learn everything you need to know about real numbers and practice exercises on the topic.
Shall we begin?

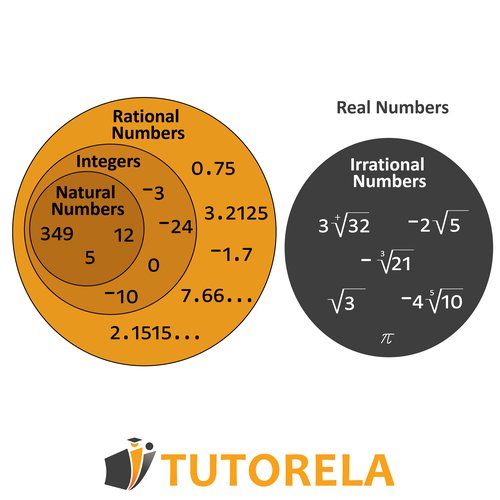
Real numbers are denoted by the letter (R) and, in fact, are all the numbers that are found on the number line.
The number line includes all numbers, from negative infinity to positive infinity, therefore, every number, whether positive, negative, whole, fraction or decimal is called real.

We use real numbers all the time, when we calculate our height, the floor in the elevator, even how many apples we buy.
And what about $0$?
$0$ is also real.

Observe - Real numbers are, in fact, a set that contains all numbers: natural, whole, rational, and irrational numbers.
Therefore, all numbers are real.
With them, we can count everything we can think of and also add, subtract, multiply, and divide.

Find the real numbers within the following set:

$98.98, 0, -4, 12.1$

Solution:
According to the definition, every number is real, whether it is an integer, positive, negative, fraction, or decimal. Therefore:
All the numbers in the set are real.

Find the real numbers within the following set:

$\sqrt2, 2\frac{4}{5}, -100000 , 1.23568$

Solution:
According to the definition, every number is real, whether it is an integer, positive, negative, fraction, or decimal. Therefore:
All the numbers in the set are real.

Find what's in the square:
$(1.5+2) \times ⬜ +\frac{6}{3}=37$

Solution:
In this exercise, there are real numbers, we have to discover which would correspond to place in the square's place.
First, let's find out what the result of the parentheses is and rewrite the exercise.
Additionally, instead of writing the fraction, we can write an integer with the same value.
We will obtain:
$(1.5+2) \times ⬜ +2=37$
Now we can say that: $3.5$ times something + $2$ equals $37$.
If we subtract $2$ from both sides we will get:
$3.5 \times ⬜=35$
Now let's think, what number multiplied by $3.5$ will give us $35$.
Divide both sides by $3.5$ and we will obtain that:
$10 = ⬜$

The price of a vase and four kitchen towels is $210$.
The price of a vase is equivalent to the price of $3$ kitchen towels.

• What is the price of the vase alone?
• What is the price of a kitchen towel?

Solution:
First, let's organize the data.
We know that the price of a vase is equivalent to the price of $3$ kitchen towels.
If we denote the price of a kitchen towel as $X$ we can say that the price of a vase is $3X$.
We also know that a vase and $3$ kitchen towels together cost $210$.
We will write it in the form of an exercise and obtain:
$3X+4x=210$
Let's solve the equation and it will give us:
$7X=210$
$X=30$

Pay attention –> $X$ represents the price of a kitchen towel.
$3X$ is the price of a vase.
If $X=30$
then the answer will be:

• The price of a vase is $90$.

$30 \times 3=90$

• The price of a kitchen towel is $30$.

Find the real numbers that are equivalent to the given real number:
$\frac{6}{3}=⬜=⬜$

Solution:
First, we know that the fraction $6\over 3$ is equivalent to the number $2$.
Furthermore,
By expanding the fraction, we can arrive at another fraction with the same value.
Let's expand $6\over 3$ by $2$. Remember, we must expand both the numerator and the denominator to not alter the value of the fraction.
We will obtain: $\frac{6}{3} = \frac{12}{6}$
Therefore, we can write $2$ and $\frac{12}{6}$.