Multiplication and Division

The order of operations teaches us the correct way to solve an expression with multiple different operations.
When solving such an expression, we will follow these steps:

Let's only solve what's inside of the parentheses - we can also call this stage opening the parentheses.
If there are no parentheses at all in the exercise, we'll move on to the next stage.

Multiplication and division in order.
After opening the parentheses, if there were any, we rewrite the exercise to avoid confusion. We will then proceed to solve the multiplication and division operations from left to right.
Rewrite the exercise once again after solving the multiplication and division operations.

Addition and subtraction in order.
We add and subtract from left to right.

Pay attention!
After each step, we will rewrite the expression. This way it will "shorten" the exercise and we can solve it more easily.
Note - no matter which expression appears inside of the parentheses, we solve it first.
If multiplication and division appear alongside addition and subtraction, multiplication and division will take precedence. Only then will we address the addition and subtraction operation from left to right.

And now? Let's practice!!

Solve the following exercise:
$3\cdot(8:2)=$

Solution:
In the first step, we will open the parentheses (solve the exercise inside the parentheses and continue.
$8:2=4$
We the proceed to rewrite the exercise as shown below:
$3\cdot4=$
Once we obtain the result from the parentheses, there is no need to keep them in the exercise.
We proceed to calculate as follows:
$3\cdot4=12$
The result of the exercise is $12$.

Let's move on to the next exercise:
$3\cdot8:2=$

Solution:
Pay attention! At first glance, it seems like the same exercise...
However there are no parentheses, hence the order of operations will be multiplication and division from left to right.
Let's start solving the exercise as shown below:
$3\cdot8=24$
Rewrite the exercise as follows:
$24:2=12$
Here we also obtain $12$
Does this mean we don't need to consider parentheses? The answer is no.

Another exercise:
$50:10\cdot4=$

Solution:
In this exercise there are no parentheses, it only has multiplication and division, hence we'll solve it from left to right.
Let's start:
$​​​​​​​50:10=5$
Rewrite the exercise as follows:
$5\cdot4=20$
The result is $20$.

Now let's move on to a similar but different exercise with parentheses and see if the result changes:
$50:(10\cdot4)=$

Solution:
Since there are parentheses here, we'll solve them first.
Let's begin:
$10\cdot4=40$
Rewrite the exercise as shown below:
$50:40=1.25$
The result is $1.25$ !
And this proves to us that parentheses change the result of the exercise and we should always address them first.

Another exercise!
$(45+5)\cdot9\cdot2=$

Solution:
First, we'll check if there are parentheses in the exercise, the answer is yes, so we'll address them first and solve them.
$45+5=50$
Rewrite the exercise without parentheses:
$50\cdot9\cdot2=$
Now we have an exercise with only multiplication operations, and according to the commutative property, we can switch between the terms.
We obtain the following:
$50\cdot2\cdot9=$
$100\cdot9=900$
The result is $900$.

Another exercise!
$(180:10)-(3\cdot3)\cdot0=$

Solution:
We'll begin with the parentheses.
Let's start
$180:10=18$
$3\cdot3=9$
Now we'll rewrite the exercise without parentheses as follows:
$18-9\cdot0=$
In the second stage, we see that there is both a multiplication and subtraction operation.
Multiplication takes precedence hence we will solve it first.
$9\cdot0=0$
Rewrite the exercise as shown below:
$18-0=18$
The result is $18$.

Note - If the calculation is simple like in this example, you can draw a small arc above the expression you are calculating and then continue with the problem without rewriting it.
For example:

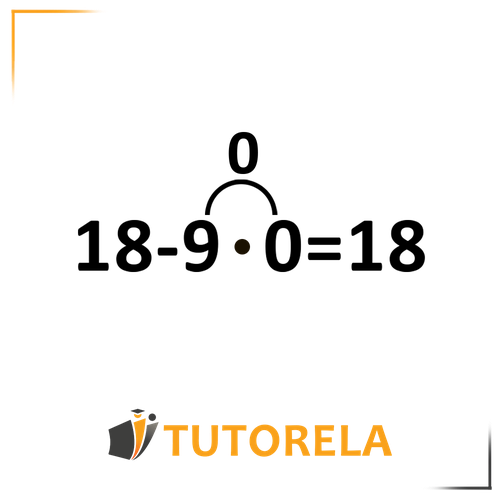
Another exercise:
$(3\cdot2):(2\cdot1)=$

Solution:
First, we'll solve the two expressions inside of the parentheses (with a small arc) and then proceed to rewrite the exercise.
We obtain the following:

The result is $3$.